Intercept Form And Points Of Intercepts: A Comprehensive Guide To Graphing Linear Equations
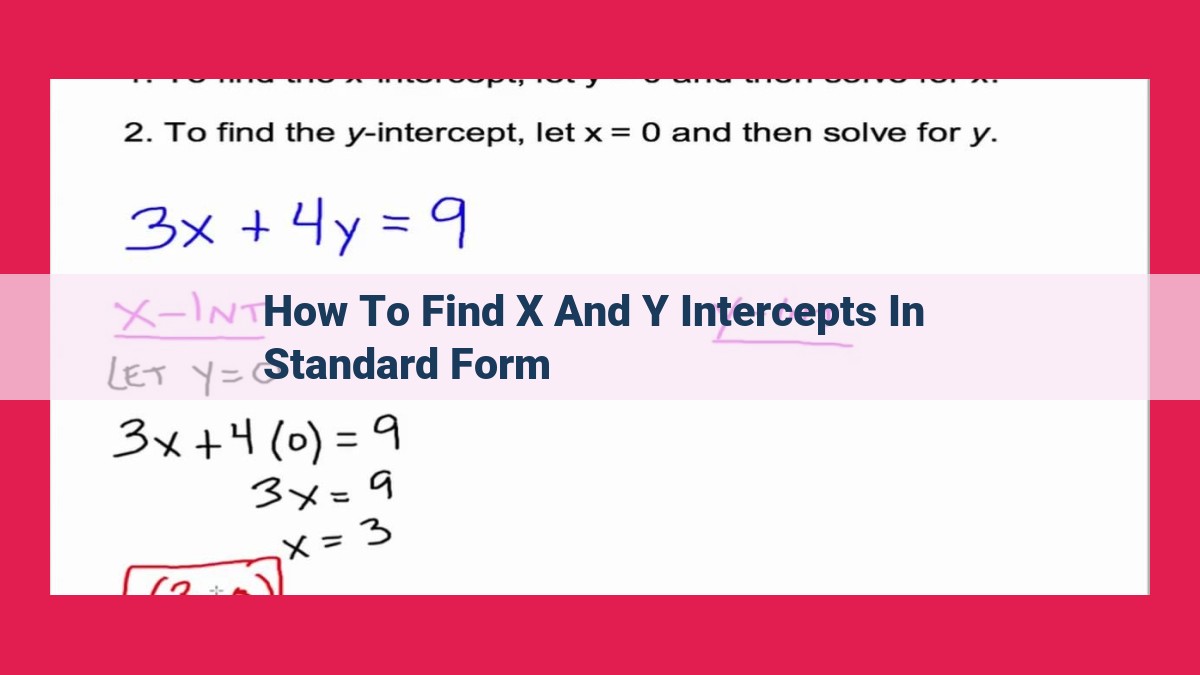
To find the x-intercept in standard form (Ax + By = C), set y = 0 and solve for x. This gives you the point where the graph crosses the x-axis. To find the y-intercept, set x = 0 and solve for y. This gives you the point where the graph crosses the y-axis. To convert standard form to intercept form (y = mx + b), solve for y by isolating it on one side of the equation. The coefficient of x (m) is the slope, and the constant (b) is the y-intercept.
Understanding the Intercept Form of a Linear Equation
Welcome to the world of linear equations and their captivating forms! Today, we’ll unravel the intercept form, a simple yet essential representation that helps us comprehend the relationship between a line’s slope and its intercepts on the x- and y-axes.
Intercept Form: y = mx + b
In the intercept form, the equation of a line is written as y = mx + b, where:
- m represents the slope of the line, indicating its steepness and direction.
- b represents the y-intercept, the point where the line crosses the y-axis.
Connection to Standard Form
The intercept form is closely related to the standard form of a linear equation, Ax + By = C. These two forms can be easily converted into each other:
-
To convert from intercept form (y = mx + b) to standard form (Ax + By = C): Multiply both sides of the intercept form by x to get mx + b = C. Then, rearrange the equation to put it in the form Ax + By = C by identifying A = m, B = 1, and C = b.
-
To convert from standard form (Ax + By = C) to intercept form (y = mx + b): Divide both sides of the standard form by x (if x ≠ 0) to isolate y. This will give you y = (A/x)x + (C/x), which simplifies to y = mx + b, where m = A/x and b = C/x.
Navigating Intercepts
The x-intercept is the point where the line crosses the x-axis, and the y-intercept is the point where the line crosses the y-axis.
- X-Intercept: To find the x-intercept, set y = 0 in the equation y = mx + b and solve for x. The result will be x = -b/m.
- Y-Intercept: To find the y-intercept, set x = 0 in the equation y = mx + b and solve for y. The result will be y = b.
Isolating Variables
Sometimes, it’s necessary to isolate x or y algebraically. For instance, to isolate x, we can subtract mx from both sides of the equation to get x = (y – b)/m. Similarly, to isolate y, we can subtract mx from both sides and then multiply by m to get y = mx + b.
The intercept form of a linear equation is a fundamental tool for understanding the geometry and behavior of lines in the coordinate plane. By knowing the slope and intercepts, we can quickly graph and analyze lines, making it indispensable for solving problems in mathematics, science, and engineering.
The X-Intercept: Unlocking the Secrets of Linear Equations
In the realm of linear equations, the X-intercept shines as a beacon of understanding, revealing where the graph of a line meets the x-axis. This pivotal point unveils a treasure trove of information, allowing us to decipher the equation’s behavior and unlock its hidden truths.
To find the X-intercept, we embark on a simple yet profound journey. We set y = 0 in the equation, effectively asking where the graph crosses the horizontal line that divides the coordinate plane in two. By doing so, we eliminate the variable y, leaving us with an equation that only depends on x.
With y out of the picture, we solve for x, unmasking the value where the graph touches the x-axis. This value represents the x-coordinate of the intercept point. Its significance lies in revealing where the line intersects the horizontal axis, providing a crucial reference point for understanding the line’s overall trajectory.
For instance, if we have the equation y = 2x – 4, to find the X-intercept, we set y = 0 and solve for x:
0 = 2x - 4
2x = 4
x = 2
Therefore, the X-intercept of the line y = 2x – 4 is (2, 0), indicating that the graph crosses the x-axis at the point x = 2. This knowledge empowers us to visualize the graph and grasp its behavior, unlocking the secrets of linear equations one step at a time.
Understanding the Y-Intercept: A Guide to Finding the Point Where a Graph Crosses the Y-Axis
In the world of mathematics, the y-intercept is an essential concept that helps us understand the behavior of linear equations and their corresponding graphs. It represents the point where a line intersects the y-axis, providing valuable insights into the equation’s relationship with the vertical axis.
Definition of the Y-Intercept
The y-intercept is defined as the point at which a graph crosses the y-axis. In other words, it is the point where the y-coordinate is zero and the x-coordinate is undefined. This means that the only part of the point that is visible on the graph is its y-coordinate.
Finding the Y-Intercept
To find the y-intercept of a linear equation, we can use a simple mathematical technique. We set the x-coordinate to zero and solve for the y-coordinate. This is because when x is zero, the equation only has a y-intercept term left.
Example:
Let’s consider the linear equation y = 2x + 1. To find its y-intercept, we set x to zero:
y = 2(0) + 1
y = 1
Therefore, the y-intercept of this equation is 1. This means that the graph of y = 2x + 1 crosses the y-axis at the point (0, 1).
Significance of the Y-Intercept
The y-intercept provides important information about the linear equation:
- Initial Value: It represents the initial value of y when x is zero. In other words, it indicates the value of y when no other variable is considered.
- Graph Behavior: The y-intercept influences the position of the graph in relation to the y-axis. A positive y-intercept means the graph starts above the y-axis, while a negative y-intercept means it starts below the y-axis.
- Linearity: If the y-intercept is zero, then the equation represents a horizontal straight line that is parallel to the x-axis.
Optimizing for SEO
To optimize this blog post for SEO, we can incorporate relevant keywords and phrases throughout the content:
- Y-intercept
- Linear equation
- Graph
- X-coordinate
- Y-coordinate
By using these keywords and phrases, we can help search engines understand the topic of our post and improve its ranking in search results.
Intercept Form, Standard Form, and the Intercepts
Welcome to our mathematical journey, where we’ll explore the world of linear equations in their two fundamental forms: intercept form and standard form. Understanding these forms is crucial for deciphering the language of lines and revealing their hidden secrets.
Let’s begin our expedition with intercept form, represented as y = mx + b. This form is like a trusty roadmap, guiding us through the graph of a line by providing two vital landmarks: the x-intercept and the y-intercept.
The x-intercept marks the spot where the line gracefully touches the horizontal axis, or the x-axis. It tells us the value of x when the line reaches out to meet the ground. To uncover this treasure, simply set y equal to zero (0 = mx + b) and solve for x. It’s like a detective game, finding the hidden coordinate where the line intercepts the x-axis.
In a similar vein, the y-intercept reveals the line’s meeting point with the vertical axis, or the y-axis. This value of y tells us how high or low the line begins its journey when x is a humble zero. To unveil this secret, we set x equal to zero (y = m(0) + b) and effortlessly solve for y.
Now, let’s delve into the enigmatic standard form, expressed as Ax + By = C. This form is our algebra maestro, allowing us to perform mathematical symphonies with lines. It’s like a magic wand that can transform any line into its intercept form counterpart.
To conjure up the intercept form from the standard form, we simply need to perform some algebraic wizardry. By isolating the variable y, we reveal the line’s true identity in the familiar form of y = mx + b. The coefficients A and B become the slope and the y-intercept, respectively. It’s a magical transformation that unveils the line’s intercepts and gives us a glimpse into its behavior.
In the realm of algebra, intercept form and standard form reign supreme, providing us with powerful tools to understand and manipulate linear equations. They are like two sides of the same coin, each offering a unique perspective on the world of lines. By mastering these forms, we unlock the secrets of the linear universe and gain a deeper appreciation for the beauty of mathematics.
Isolating X: Unveiling the Essential Variable
In the realm of linear equations, isolating x is a crucial skill that unravels the mysteries hidden within. By isolating x, we can determine the x-coordinate of key points on the graph, such as the x-intercept.
Step 1: Subtraction in Harmony
First, rid the equation of any constant terms by subtracting them from both sides. This creates a streamlined equation that sets the stage for isolating x.
Step 2: Division with Purpose
Next, focus on the coefficient of x. If it’s not 1, divide both sides by that coefficient. This step brings the equation a step closer to expressing x alone on one side.
Step 3: Unveiling X’s Secret
With the coefficient of x reduced to 1, the final subtraction or addition completes the isolation process. By performing the opposite operation of the constant on the other side of the equation, x stands revealed.
Example: A Journey of Isolation
Consider the equation 3x + 5 = 14. Let’s isolate x step by step:
- Subtract 5 from both sides: 3x = 9
- Divide both sides by 3: x = 3
And there you have it! The value of x is 3.
Isolating x is a fundamental operation that grants access to the x-coordinate of crucial points on the graph. Embracing this technique will empower you to solve linear equations with confidence and unravel the secrets of the coordinate plane.
Isolating Y: Unlocking the Power of the Y-Axis
In the world of linear equations, navigating the X and Y axes is crucial for understanding the behavior of graphs. While we’ve explored the concept of finding the X-intercept, it’s equally important to master the art of isolating Y.
What is Isolating Y?
Isolating Y is the process of algebraically expressing an equation in terms of Y. By rearranging the equation, we can solve for Y and determine the values of the dependent variable for any given value of the independent variable (X).
How to Isolate Y: A Step-by-Step Example
Let’s consider the equation: 2X + 3Y = 12. To isolate Y, we follow these steps:
- Subtract 2X from both sides: This gives us 3Y = 12 – 2X.
- Divide both sides by 3: We obtain Y = (12 – 2X) / 3.
Voila! We have successfully isolated Y.
Why Is Isolating Y Important?
Isolating Y is a valuable skill for several reasons:
- Graphing: It allows us to determine the Y-intercept (where the graph crosses the Y-axis) and plot the graph more accurately.
- Solving Applied Problems: Many real-world problems involve equations where we need to isolate Y to find the value of the dependent variable (e.g., distance, cost, etc.).
- Algebraic Manipulation: Isolating Y is a fundamental step in performing various algebraic operations, such as substituting values or simplifying equations.
Additional Tips
- When isolating Y, avoid multiplying or dividing both sides by X if it is equal to zero, as this can lead to division by zero errors.
- Always check your solution by plugging it back into the original equation to ensure accuracy.