How To Write Inequalities In Interval Notation For Enhanced Clarity And Search Visibility

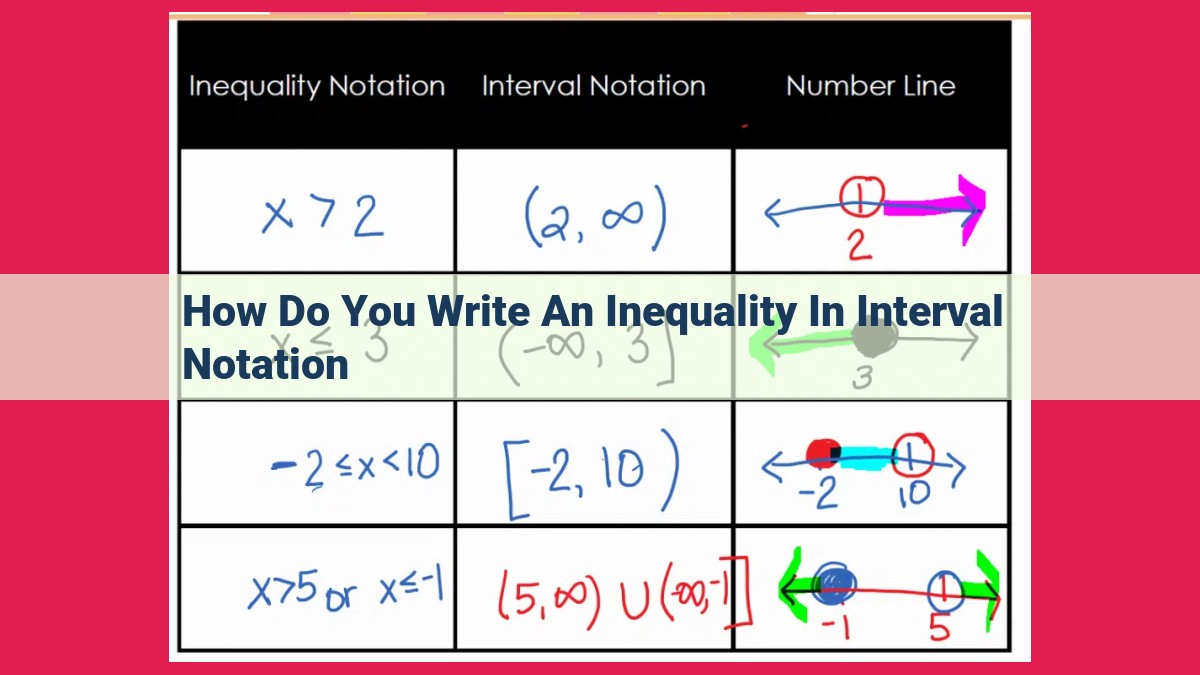
In interval notation, inequalities are represented using symbols to indicate the inclusion or exclusion of endpoints. Open intervals, denoted by parentheses, exclude endpoints. Closed intervals, denoted by brackets, include endpoints. Half-open intervals, using a combination of parentheses and brackets, include one endpoint. Infinity (∞) and negative infinity (-∞) represent unbounded intervals extending infinitely in the positive and negative directions. To write inequalities in interval notation, identify the endpoint values, determine if they are included or excluded, and use the appropriate notation based on the type of inequality (less than, greater than, less than or equal to, greater than or equal to).

Interval Notation: Demystifying the Language of Numbers

In the realm of mathematics, precision is paramount. Interval notation serves as a powerful tool for describing sets of numbers with utmost accuracy. It’s a language that allows us to communicate the boundaries of numerical ranges, both finite and infinite.

Understanding Interval Notation

Think of interval notation as the mathematical equivalent of a fence. It defines the boundaries of a set of numbers, specifying which numbers are included and which are excluded. The type of interval is determined by the endpoints of the fence: open, closed, or half-open.

Open Intervals: Excluding Endpoints

Imagine a fence with both gates swinging open. Open intervals exclude their endpoints. They contain all the numbers between two given endpoints but not the endpoints themselves. We represent open intervals using parentheses: (a, b). For instance, (2, 5) represents the set of all numbers greater than 2 but less than 5.

Closed Intervals: Including Endpoints

Now, picture a fence with both gates locked shut. Closed intervals include their endpoints. They contain all the numbers between two given endpoints, including the endpoints themselves. We use brackets to represent closed intervals: [a, b]. For example, [3, 7] represents the set of all numbers greater than or equal to 3 and less than or equal to 7.

Half-Open Intervals: Including One Endpoint

When one gate is open and the other closed, we have a half-open interval. It includes one endpoint but not the other. We use a combination of parentheses and brackets to represent half-open intervals: [a, b) or (a, b]. For instance, [2, 4) represents the set of numbers greater than or equal to 2 but less than 4.

Types of Interval Notation

In the realm of mathematics, numbers dance in intricate patterns, obeying precise rules. One such rule-based language is interval notation, a powerful tool for describing sets of numbers.

Imagine a number line stretching infinitely in both directions. Along this line, there are three primary types of intervals:

Open Intervals: Excluding Endpoints

Open intervals are like gateways with no doors. They invite numbers to enter, but only those that stay clear of the boundaries. These intervals are represented by parentheses, like (a, b). For example, (2, 5) includes all numbers greater than 2 and less than 5.

Closed Intervals: Including Endpoints

Closed intervals, on the other hand, are like fenced-in pens. Numbers are welcomed to roam within, including the boundaries marked by the endpoints. These intervals are represented by brackets, like [a, b]. For instance, [2, 5] encompasses all numbers from and including 2 to and including 5.

Half-Open Intervals: Including One Endpoint

Half-open intervals are a blend of the previous two. They extend an open invitation to numbers on one side of the boundary but close the door on the other. These intervals use both parentheses and brackets, such as (a, b] or [a, b). For example, (2, 5] includes all numbers greater than 2 and up to and including 5, while [2, 5) includes numbers from and including 2 to less than 5.

**Open Intervals: Excluding Endpoints**

Imagine you’re organizing a party, but you’ve set specific times for guests to arrive and leave. Let’s say you want them to come after 7 pm and leave before 10 pm. This scenario resembles an open interval in mathematics, where numbers are restricted by endpoints that aren’t included.

In mathematical terms, an open interval is a set of numbers that lie between two endpoints, but exclude those endpoints. It’s like a party guest who shows up a little late and leaves a little early, never actually being on time.

To represent an open interval, we use parentheses:

(a, b)

Where a is the lower endpoint (the “after” in our party example) and b is the upper endpoint (the “before”). For instance, the interval (3, 5) includes all numbers greater than 3 but less than 5: 3.1, 4.2, 4.999… but not 3 or 5.

Think of open intervals as two doors that are slightly ajar. You can “enter” the interval from one door (the lower endpoint) and “exit” through the other (the upper endpoint), but you never stand right on either door.

Closed Intervals: Embracing the Endpoints

Closed intervals are mathematical notations that warmly embrace their endpoints. Unlike open intervals that shy away from their boundaries, closed intervals extend their arms to include both end values. This concept is crucial in mathematics and its applications, allowing us to define sets of numbers with precise boundaries.

Representing Closed Intervals with Brackets

Closed intervals are represented using square brackets. These brackets symbolize the firm hold that the endpoints have on the set of numbers. For instance, the closed interval [2, 5] represents all numbers between 2 and 5, including both 2 and 5.

Examples of Closed Intervals

Consider the closed interval [-3, 7]. This interval includes all numbers from -3 to 7, encompassing -3 as its left endpoint and 7 as its right endpoint. Another example is the closed interval [0, 0]. This degenerate interval consists of just the number 0 because its endpoints coincide.

Importance of Closed Intervals

Closed intervals play a vital role in defining sets of numbers with specific boundaries. They are used in various fields, such as calculus and statistics, to represent intervals of values that satisfy certain conditions. By including the endpoints, closed intervals provide a clear and precise understanding of the range of numbers under consideration.

Half-Open Intervals: Embracing an Endpoint, Eschewing the Other

When delving into the realm of interval notation, we encounter a fascinating entity known as the half-open interval. As its name suggests, a half-open interval is a set of numbers that warmly welcomes one endpoint into its fold while politely declining the other.

Think of a half-open interval as a door that is slightly ajar. It invites certain numbers to enter, but it firmly holds the line against others. To illustrate, let’s consider the interval [2, 5). This interval includes the number 2 (the left endpoint) but excludes 5 (the right endpoint). It’s as if there’s an invisible boundary at 5, preventing it from stepping into the interval’s domain.

Another way to represent a half-open interval is using parentheses. For instance, the interval (2, 5) also denotes a half-open interval, with 2 included and 5 excluded. These two notations, [2, 5) and (2, 5), are equivalent and can be used interchangeably.

Half-open intervals find their niche in various mathematical scenarios. They help us precisely describe sets of numbers that fall within a specific range. Whether it’s calculating areas under curves or solving inequalities, half-open intervals serve as versatile tools in the mathematician’s arsenal.

Interval Notation: Exploring Infinity and Negative Infinity as Endpoints

In the realm of mathematics, interval notation serves as a powerful tool for representing sets of numbers. It allows us to describe a range of values with remarkable precision, opening up a world of possibilities in mathematical analysis and problem-solving.

At times, we encounter sets of numbers that extend beyond the boundaries of the real number line. This is where the concepts of infinity (∞) and negative infinity (-∞) come into play as endpoints. They act as ethereal sentinels, marking the boundless limits of mathematical domains.

Understanding Infinity

Infinity, symbolized by the enigmatic symbol ∞, represents a quantity that is limitless and unbounded. It is like a vast, ever-expanding ocean with no discernible end. In interval notation, an open interval extending endlessly to the right is represented by the notation (a, ∞), where a is the left endpoint.

Grasping Negative Infinity

Negative infinity, denoted by (-∞), is the mirror image of infinity. It represents a limitless and unbounded quantity in the negative direction. In interval notation, an open interval extending endlessly to the left is represented by the notation (-∞, a), where a is the right endpoint.

Examples in Action

Let’s illustrate these concepts with examples. The interval (5, ∞) represents the set of all positive numbers greater than 5, including decimals and fractions. The interval (-∞, 10) represents the set of all negative numbers and 10 itself.

Beyond Boundaries

Infinity and negative infinity can also be used in closed intervals. A closed interval [a, ∞) includes the left endpoint a but extends infinitely to the right. Similarly, a closed interval (-∞, b] includes the right endpoint b but extends infinitely to the left.

These extended intervals enable us to describe sets of numbers that have no upper or lower bounds, respectively. This opens up new avenues for mathematical exploration and modeling real-world phenomena that exhibit boundless characteristics.

Infinity and negative infinity as endpoints in interval notation extend the expressive power of this mathematical tool. They allow us to represent sets of numbers that transcend the confines of the real number line, opening up a vast and uncharted territory for mathematical exploration. Embracing these concepts unlocks a deeper understanding of mathematics and empowers us to tackle problems with greater precision and ingenuity.

Writing Inequalities in Interval Notation: A Step-by-Step Guide

Understanding interval notation is crucial in mathematics and its various applications. One important aspect is the ability to convert inequalities into interval notation. Here’s a comprehensive guide to help you master this skill:

Step 1: Identify the Inequality Symbol

Determine the inequality symbol used in the expression, such as <, ≤, >, or ≥. This symbol indicates the relationship between the variable and the constant.

Step 2: Determine the Type of Interval

Based on the inequality symbol, you can identify the type of interval:

• Open Interval: Endpoints are not included, represented by parentheses ( and ).
• Closed Interval: Endpoints are included, represented by brackets [ and ].

Step 3: Represent the Inequality

Depending on the inequality symbol and interval type, represent the inequality as follows:

• Less Than (<): (a, b) (Open Interval)
• Less Than or Equal to (≤): [a, b] (Closed Interval)
• Greater Than (>): (a, b) (Open Interval)
• Greater Than or Equal to (≥): [a, b] (Closed Interval)

Example:

Convert the inequality x > 5 into interval notation.

Since the symbol is > and we exclude the endpoint, it represents an open interval. Therefore, the interval notation is (5, ∞).

Additional Notes:

• Infinity (∞) and Negative Infinity (-∞): They can be used as endpoints to represent unbounded intervals.
• Parentheses and Brackets: Parentheses indicate exclusion of endpoints, while brackets indicate inclusion.
• Compound Inequalities: For inequalities with multiple variables or inequalities, use the interval notation union or intersection depending on the conditions.

Mastering these steps will enable you to effectively convert inequalities into interval notation, enhancing your mathematical understanding.