Identity Properties In Arithmetic: Zero, One, And Their Crucial Roles

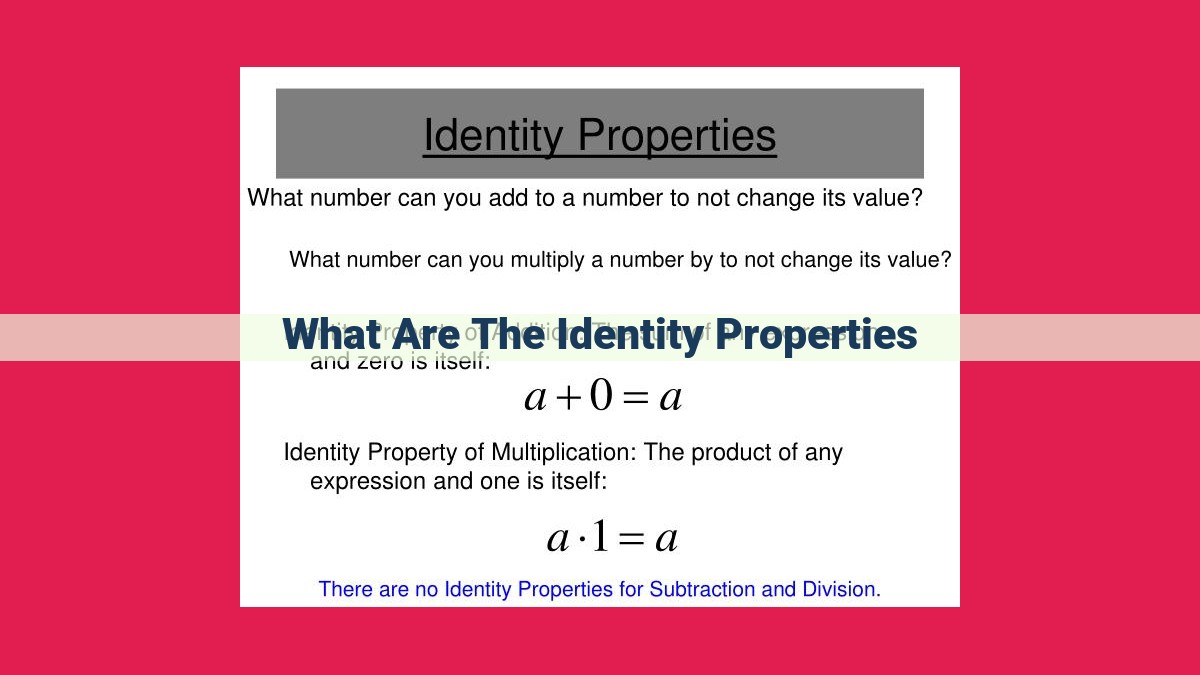
Identity properties define two special numbers, zero and one, that play a crucial role in arithmetic. The additive identity property states that any number added to zero remains unchanged (a + 0 = a). Similarly, the multiplicative identity property states that any number multiplied by one remains unchanged (a * 1 = a). These properties simplify calculations, solve algebraic equations, and enhance understanding of number systems. Identity properties highlight the significance of zero as the additive identity and one as the multiplicative identity, providing a foundation for mathematical concepts and applications.

Identity Properties: The Foundation of Mathematical Operations

In the captivating realm of mathematics, identity properties play a pivotal role, providing the cornerstone for a plethora of algebraic operations. Their significance lies in their ability to maintain the integrity of an expression while simplifying its structure.

Imagine a magical kingdom where every number has a special friend, an identity property, that accompanies it on every adventure. For addition, this companion is the enigmatic zero. When zero joins forces with any number, it leaves that number unchanged, as if a gentle breeze that whispers through a forest, leaving every leaf undisturbed.

Similarly, the realm of multiplication has its own enchanted guardian, the enigmatic one. When one dances with any number, it transforms it into a replica of itself, like a sorcerer who conjures an identical duplicate of his apprentice. This mystical partnership ensures that the essence of a number remains intact.

Identity properties possess a remarkable ability to solve algebraic equations with ease. They act like a pair of hands that gently guide us towards the unknown, revealing the hidden secrets of mathematical puzzles. Their simplifying power is equally impressive, enabling us to streamline complex expressions and expose their underlying elegance.

Furthermore, identity properties serve as the backbone of number systems, providing a framework upon which the intricate tapestry of mathematics is woven. They are the invisible threads that connect the dots, ensuring that the operations of addition and multiplication follow a consistent and harmonious set of rules.

In conclusion, identity properties are not mere mathematical concepts; they are the guardians of simplicity, solvers of equations, and the foundation upon which the grand edifice of mathematics is built. Their significance permeates every corner of our numerical universe, shaping our understanding of the world around us. As we delve deeper into the intricate web of mathematics, let us always remember the power of identity properties, the unsung heroes of algebraic harmony.

Additive Identity: Zero

• Concept of zero as the additive identity
• The additive identity property: a + 0 = a

Understanding Zero as the Additive Identity

In the vast realm of mathematics, certain numbers possess special characteristics that play a pivotal role in defining the way numerical operations behave. One such number is zero, which stands out as the additive identity.

Additive identity is a fundamental concept in mathematics. It refers to the number that, when added to any other number, leaves that number unchanged. Zero embodies this property, making it the additive identity for the set of all real numbers.

To illustrate, consider the simple equation:

a + 0 = a

where a represents any real number. Regardless of the value of a, adding zero to it always results in a itself. This mathematical truth is known as the additive identity property.

The additive identity property holds true in all number systems. For instance, in the integer system, adding zero to 10 yields 10, and in the rational system, adding zero to 1/2 yields 1/2. This property simplifies calculations and allows us to perform operations with ease.

To delve deeper into the significance of zero as the additive identity, let’s consider its applications in real-life scenarios:

• Solving Algebraic Equations: The additive identity property enables us to solve algebraic equations involving addition or subtraction. By isolating the variable on one side of the equation and adding or subtracting zero from both sides, we can simplify the equation and find its solution.
• Simplifying Expressions: Zero’s additive identity property can help simplify algebraic expressions. By adding or subtracting zero to terms, we can rearrange and combine them without altering the overall value of the expression.
• Understanding Number Systems: The concept of additive identity is foundational in understanding the properties of different number systems. It helps us define the basic operations and relationships between numbers in a given system.

The Multiplicative Identity: The Power of One

In the realm of mathematics, identity properties play a pivotal role in shaping our understanding of numbers and operations. One such property is the multiplicative identity, where the number one stands tall as the indispensable factor that leaves every number unchanged.

Delving into the concept of one as the multiplicative identity, we find that when any number is multiplied by one, the result is always the same number. This fundamental property is encapsulated in the multiplicative identity equation: a * 1 = a, where “a” represents any arbitrary number.

Let’s unravel this property with a simple example. Take the number 5. Multiply it by one, and you get… 5! It’s as if one has a magical power to preserve the original value of a number, much like a mirror that reflects an image without altering it.

This seemingly simple property has far-reaching applications. In solving algebraic equations, the multiplicative identity allows us to isolate the variable by multiplying both sides of the equation by one. It also plays a crucial role in simplifying expressions, enabling us to rewrite complex algebraic terms into more manageable forms.

Moreover, the multiplicative identity is indispensable in understanding number systems. It provides the foundation for defining the concept of unity and serves as the starting point for counting and arithmetic operations. Without one as the multiplicative identity, our number system would be incomplete and highly disoriented.

So, there you have it—the multiplicative identity, the unsung hero of mathematics. The number one, with its seemingly modest presence, wields immense power in the mathematical realm, ensuring that the world of numbers remains consistent and predictable. Whether it’s solving equations, simplifying expressions, or understanding number systems, the multiplicative identity is the invisible force that makes it all possible.

Identity Properties: The Cornerstones of Mathematical Operations

In the realm of mathematics, identity properties serve as indispensable tools, aiding us in solving equations, streamlining expressions, and comprehending number systems. These properties introduce us to the unique numbers, zero and one, that hold transformative powers in algebraic operations.

Zero: The Additive Identity

Just as the number zero represents nothingness in real-life scenarios, it also embodies the concept of additive identity in mathematics. The additive identity property dictates that when we add zero to any number, a, the result remains a. This fundamental property forms the foundation for addition and the concept of a “neutral element” in mathematical operations.

One: The Multiplicative Identity

One, the fundamental building block of counting, plays a similar role in multiplication as zero does in addition. It serves as the multiplicative identity, implying that multiplying any number, a, by one yields the original number, a. This property underscores the role of one as the starting point for multiplication and highlights its significance as a neutral element in multiplication operations.

Applications of Identity Properties

The versatility of identity properties extends far beyond their fundamental definitions. They find wide-ranging applications in various mathematical domains:

• Solving Algebraic Equations: Identity properties provide a crucial foundation for solving algebraic equations. By isolating the variable using these properties, we can determine its value. For instance, in the equation x + 0 = 5, using the additive identity property, we can isolate the variable x and find that x equals 5.

• Simplifying Expressions: Identity properties offer powerful techniques for simplifying algebraic expressions. By replacing numbers with their identity counterparts (zero or one), we can streamline expressions and make them easier to solve. For instance, the expression a + 0 – 2 + 1 simplifies to a – 1 using the additive and multiplicative identity properties.

• Understanding Number Systems: Identity properties shed light on how number systems work. By understanding the role of zero and one as additive and multiplicative identities, we gain insights into the structure and operations of different number systems, including the decimal, binary, and octal systems.

In conclusion, identity properties, with their cornerstone numbers zero and one, provide the bedrock for algebraic operations. Their applications extend across various aspects of mathematics, from solving equations to simplifying expressions and unraveling the complexities of number systems. Embracing the power of identity properties empowers us to navigate the world of mathematics with confidence and precision.