Comprehensive Guide To Finding Rational Function Zeros: Essential Methods And Applications

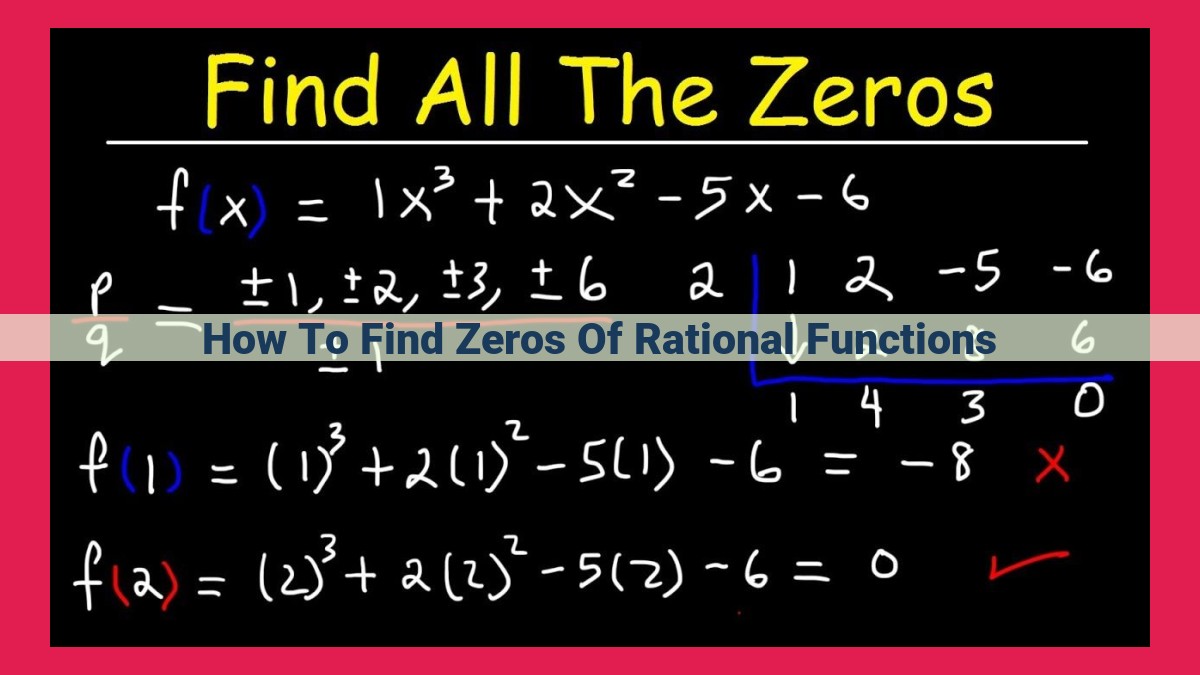
To find the zeros of a rational function, various methods are employed: (1) Identifying linear and quadratic factors in the numerator, (2) Canceling common factors between numerator and denominator, (3) Using synthetic division to divide numerator and denominator, (4) Applying the Rational Root Theorem for polynomials with integer coefficients, (5) Utilizing Descartes’ Rule of Signs to determine the number of positive and negative zeros. These methods facilitate the simplification and analysis of rational functions, allowing for a thorough understanding of their behavior and real-world applications.

• Definition and properties of rational functions
• What are zeroes of rational functions?

Finding the Zeroes of Rational Functions: A Journey into Algebraic Mysteries

In the realm of algebra, rational functions hold a special place as expressions of the quotient of two polynomials. These mathematical equations are often encountered in various fields, from science to engineering. Understanding how to find the zeroes of rational functions is essential for unlocking their secrets.

So, what exactly are rational functions? Rational functions are defined as functions that can be expressed as the ratio of two polynomials. In other words, they take the form of P(x) / Q(x), where P(x) and Q(x) are polynomials.

One crucial aspect of rational functions is their zeroes. The zeroes of a function are the values of the independent variable (in this case, x) that make the function equal to zero. In the context of rational functions, finding the zeroes involves determining the values of x that make the numerator, P(x), equal to zero.

The process of finding zeroes may sound daunting, but fear not! Numerous techniques and tools are available to aid in this algebraic quest. These methods range from simple factoring to more advanced algebraic techniques. In this blog post, we will embark on a step-by-step journey through the various approaches for finding the zeroes of rational functions, making this algebraic adventure accessible and enjoyable.

Unveiling the Zeroes: Delving into Linear Factors of Rational Functions

In the realm of mathematics, rational functions reign supreme, boasting an intricate interplay between polynomials in their numerator and denominator. These functions present us with the tantalizing task of finding their zeroes – the enigmatic points where they gracefully touch the x-axis.

Linear Factors as Guiding Lights

One illuminating approach to unraveling these mysteries lies in scrutinizing the numerator’s linear factors. These factors, akin to the building blocks of the numerator, hold the key to uncovering some of the function’s hidden secrets.

When the numerator possesses linear factors of the form (x – a), where a represents a constant, it holds a profound revelation: the zero of this linear factor (x = a) is also a zero of the entire rational function.

Factoring Techniques: A Path to Revelation

To successfully employ this strategy, we must master the art of factoring the numerator. This intricate process aims to decompose the numerator into its constituent linear factors. Here, a repertoire of factoring techniques beckons us:

• Common Factor Extraction: Identifying and lifting out the greatest common factor from each term of the numerator.
• Grouping: Rearranging terms into groups that share a common factor.
• Difference of Squares: Recognizing expressions of the form (a² – b²) and factoring as (a + b)(a – b).

Illuminating Examples

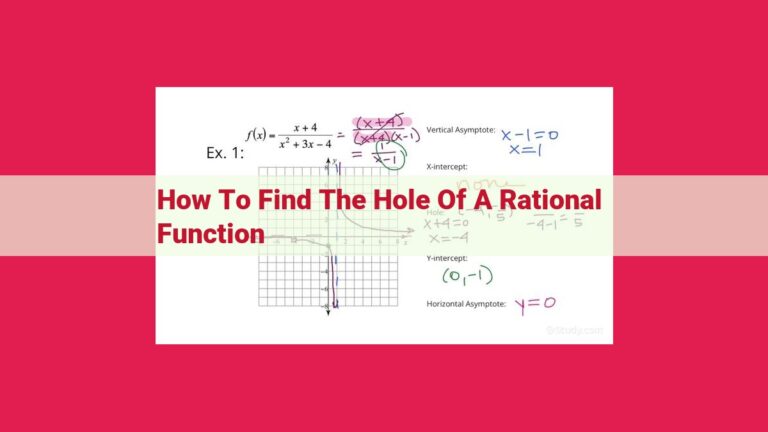
Consider the rational function f(x) = (x – 2)(x + 1) / (x² – 4). To determine its zeroes, we focus on the linear factor (x – 2). The zero of this factor is x = 2, which is indeed a zero of the entire function.

Similarly, for the function g(x) = (x – 3)(x² – 1) / (x³ + 2x² – 3x), the linear factors (x – 3) and (x + 1) reveal the zeroes x = 3 and x = -1, respectively.

Through the meticulous exploration of linear factors, we gain a potent tool for uncovering the zeroes of rational functions. These factors guide us towards the hidden realms of the function’s behavior, illuminating the points where it intersects the x-axis. Armed with factoring techniques and a keen eye for patterns, we can unravel the enigmatic tapestry of rational functions, unlocking the secrets that lie within.

Finding Zeroes of Rational Functions: Unraveling the Mysteries of Quadratic Factors

When dealing with rational functions, understanding how to find their zeroes is crucial. Among the various methods, using quadratic factors is a powerful approach. This blog post will dive into the world of quadratic factors and how they help us uncover the hidden zeroes of rational functions.

Using the Quadratic Formula

The quadratic formula is a valuable tool for finding the zeroes of any quadratic expression. It states that the zeroes of a quadratic equation of the form ax² + bx + c = 0 are given by:

x = (-b ± √(b² - 4ac)) / 2a

By applying this formula to the numerator or denominator of a rational function, we can find its zeroes. For example, consider the rational function f(x) = (x² – 4) / (x + 2). Using the quadratic formula on the numerator, we find that the zeroes of f(x) are x = ±2.

Factoring Quadratic Expressions into Linear Factors

Another approach to finding zeroes using quadratic factors is to factor them into linear factors. For instance, the quadratic expression x² – 4 can be factored as (x + 2)(x – 2). This means that the zeroes of x² – 4 are x = -2 and x = 2.

This technique can be extended to rational functions with quadratic denominators. For example, if we have a rational function g(x) = (x – 3) / (x² – 4), we can factor the denominator into (x + 2)(x – 2). Thus, the zeroes of g(x) are x = 3, x = -2, and x = 2.

Mastering the art of finding zeroes using quadratic factors empowers us to unravel the mysteries of rational functions. Whether it’s employing the quadratic formula or factoring quadratic expressions, these methods provide accurate and reliable results. By delving into the world of zeroes, we unlock a deeper understanding of rational functions and their behavior, enabling us to confidently navigate more complex mathematical challenges.

Simplifying Rational Functions through Common Factor Cancellation

Conceptualizing Rational Functions

Rational functions are mathematical expressions that embody fractions, where the numerator and denominator are polynomials. They offer invaluable insights into various real-world scenarios.

Unveiling Common Factors: A Path to Simplification

Amidst the complex edifice of rational functions, common factors often emerge as beacons of simplification. By adroitly identifying and skillfully canceling these shared elements between the numerator and denominator, we unlock the doorway to streamlined representations.

Advantages:

• Enhanced Comprehensibility: Stripping away unnecessary factors amplifies function clarity, rendering them more intelligible to the uninitiated.
• Efficient Calculations: Eliminating commonalities reduces the computational burden, expediting the evaluation process.

Applications:

Rational function simplification finds widespread use across diverse disciplines:

• Physics: Modeling oscillatory systems and electromagnetic phenomena
• Engineering: Designing circuits and analyzing fluid dynamics
• Economics: Forecasting economic trends and optimizing resource allocation

Mastering the art of common factor cancellation empowers us to elevate our understanding of rational functions. By peeling away the layers of complexity, we not only simplify these functions but also pave the path for more efficient problem-solving and deeper comprehension in a myriad of real-world applications.

Synthetic Division: A Powerful Tool for Finding Rational Function Zeroes

Throughout our mathematical endeavors, we often encounter rational functions, those intertwined expressions of polynomial functions in both the numerator and denominator. Determining the zeroes of these functions, the points where they vanish, can be a crucial step in understanding their behavior. While there exist various techniques to uncover these zeroes, one particularly efficient method stands out: synthetic division.

What is Synthetic Division?

Synthetic division is a simplified form of long division specifically tailored for polynomials. It condenses the process into a series of operations that can be quickly executed. For a rational function f(x) = p(x)/q(x), where p(x) is the numerator and q(x) is the denominator, synthetic division proceeds as follows:

1. Set up the division: Write down the coefficients of the numerator p(x) in order, from highest degree term to constant term. Below these, write down the coefficients of the denominator q(x).

2. Bring down the first coefficient: Copy the first coefficient of p(x) to the result row. This becomes the first term of the quotient.

3. Multiply and add: Multiply the first coefficient of q(x) by the result, then add it to the second coefficient of p(x). Write the result below.

4. Multiply and add: Repeat step 3 for the next coefficient of p(x), multiplying by the quotient and adding to the next coefficient. Continue until you reach the constant term of p(x).

Finding Zeroes with Synthetic Division

The power of synthetic division lies in its ability to identify zeroes. If the remainder of the synthetic division process is zero, then the constant term of q(x) is a zero of f(x). This is because the remainder represents the constant term of the quotient, which is zero when the division is exact.

Applying Synthetic Division

To illustrate how synthetic division works, let’s consider the rational function f(x) = (x² – 2x + 1)/(x – 1). Setting up the synthetic division as follows:

1 | 1 -2  1
---
    1  -1  0

Performing the division, we obtain:

1 | 1 -2  1
---
    1  -1  0

Since the remainder is zero, we conclude that x – 1 = 0, or x = 1, is a zero of f(x).

Synthetic division is an invaluable tool in the arsenal of any mathematician tackling rational functions. Its simplicity and efficiency make it an indispensable technique for finding zeroes and understanding the behavior of these functions. Whether you’re delving into complex mathematical concepts or encountering rational functions in real-world applications, synthetic division will serve you well.

Unveiling the Rational Root Theorem: Finding Zeroes with Integer Coefficients

In the realm of mathematics, extracting the zeroes of rational functions is a fundamental skill. One potent tool in our arsenal is the Rational Root Theorem, which unveils a method to uncover zeroes for polynomials with integer coefficients.

Imagine a polynomial with integer coefficients, a mathematical expression resembling a standard polynomial but with whole number coefficients. The Rational Root Theorem whispers that any rational zero of this polynomial must be expressible as a fraction of two integers, where the numerator is a factor of the constant term and the denominator is a factor of the leading coefficient.

The Technique: A Step-by-Step Journey

To embark on this rational adventure, we follow a six-step process:

1. List the factors of the constant term: Identify all the whole numbers that divide the constant term (the last number in the polynomial) evenly.
2. List the factors of the leading coefficient: Determine all the whole numbers that divide the leading coefficient (the first number in the polynomial) evenly.
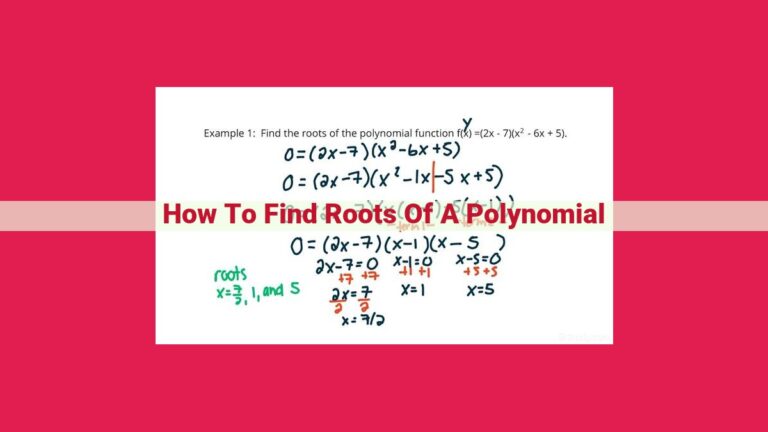
3. Form possible rational zeroes: Combine the factors from both lists to create possible rational zeroes. These are fractions with the factors of the constant term as numerators and the factors of the leading coefficient as denominators.
4. Test the possible zeroes: Substitute each rational zero into the polynomial and verify if the result is zero. If so, you have found a zero.
5. If no exact zeroes are found: Divide the polynomial by the factor corresponding to the rational zero you tested. This will create a new polynomial with a lowered degree.
6. Repeat the process: Recursively apply steps 1-5 to the new polynomial until all rational zeroes are found or there are no more possible zeroes to test.

Limitations to Consider

While the Rational Root Theorem provides a structured approach, it does have limitations:

• It only applies to polynomials with integer coefficients.
• It does not guarantee finding all zeroes, as some zeroes may be irrational (non-fractional).
• The theorem is most effective when the constant term and leading coefficient have relatively few factors.

Applications in the Real World

The Rational Root Theorem extends beyond theoretical exploration, finding practical applications in fields such as:

• Engineering: Designing systems and structures that withstand external forces
• Physics: Modeling the properties of materials and predicting their behavior
• Economics: Analyzing market trends and forecasting financial outcomes

By embracing the Rational Root Theorem, we equip ourselves with a powerful technique to uncover the hidden zeroes of rational functions, unlocking a deeper understanding of mathematical expressions and their real-world applications.

Unraveling the Secrets of Rational Functions: Exploring Descartes’ Rule of Signs

In the realm of mathematics, rational functions hold a unique place, often used to describe real-world phenomena and complex systems. Understanding their properties, including the elusive zeroes, is crucial for solving equations and delving deeper into the complexities of algebra. Among the various techniques for finding these zeroes, Descartes’ Rule of Signs stands out as a valuable tool.

A Glimpse into Descartes’ Rule of Signs

Descartes’ Rule of Signs provides a simple yet powerful way to determine the number of positive and negative zeroes of a polynomial. By analyzing the sequence of coefficients, it offers insights into the behavior of the polynomial and its potential solutions.

Applying to Rational Functions

The beauty of Descartes’ Rule of Signs lies in its applicability to rational functions. By focusing on the numerator of a rational function, we can uncover valuable information about the function’s zeroes. Remember that zeroes represent the values of the input variable that make the function equal to zero.

Counting Positive Zeroes

To count the number of positive zeroes, we first count the number of sign changes in the coefficients of the numerator when written in descending order. Each sign change corresponds to one possible positive zero. For instance, if the numerator is x^3 – 2x^2 + x – 1, we have three sign changes: (+) to (-) to (+) to (-). This suggests that the rational function may have three positive zeroes.

Counting Negative Zeroes

Determining the number of negative zeroes follows a similar process. We replace x with -x in the numerator and count the number of sign changes in the resulting coefficients. Negative sign changes indicate potential negative zeroes. Using the same example, when we replace x with -x, we get x^3 + 2x^2 + x – 1, with two sign changes: (+) to (-) to (+). This implies that the rational function may have two negative zeroes.

Limitations and Considerations

While Descartes’ Rule of Signs is a useful tool, it has limitations. It only provides information about the number of possible zeroes, not their exact values. Additionally, it may overestimate the number of zeroes if the polynomial has complex zeroes or repeated zeroes.

Descartes’ Rule of Signs is a valuable technique for understanding the behavior of rational functions. By applying it to the numerator of a rational function, we can gain insights into the number of positive and negative zeroes. This information can guide our further exploration and analysis, helping us unravel the mysteries of these mathematical expressions.