Common Fractions: Understanding Parts Of A Whole
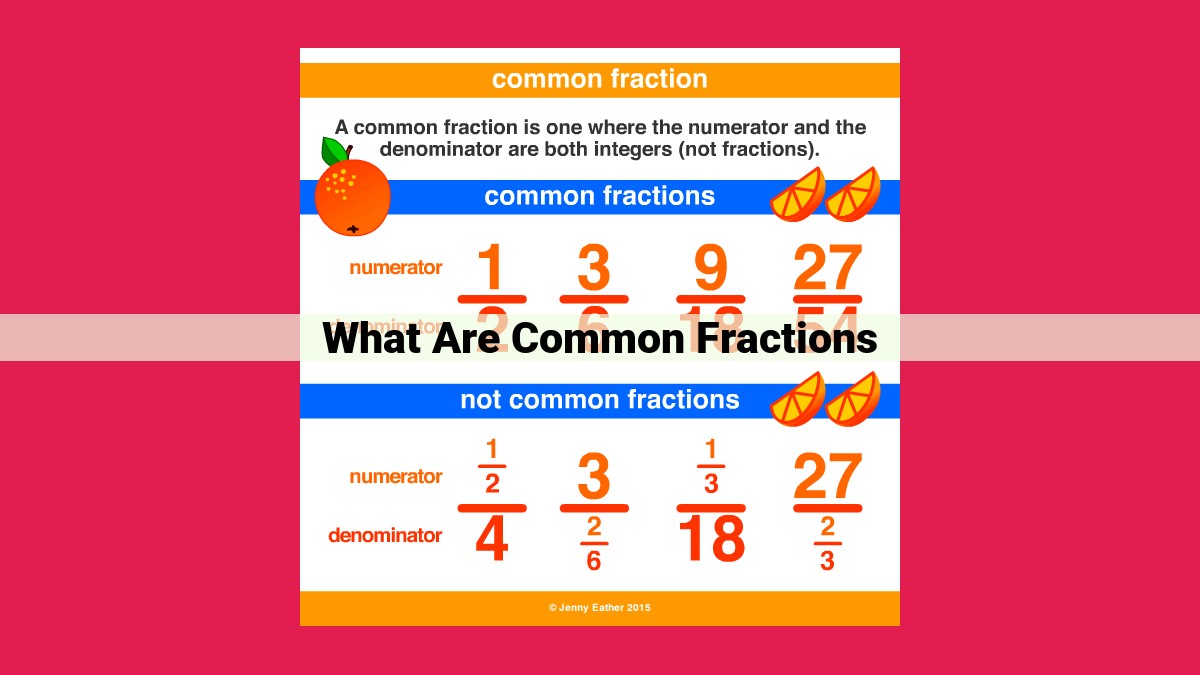
Common fractions, also known as vulgar fractions, represent parts of a whole or group. They consist of two numbers separated by a horizontal line: the numerator (above the line) and the denominator (below the line). The numerator indicates the number of parts taken, while the denominator shows the total number of equal parts in the whole. Fractions are classified as proper (numerator is smaller than the denominator), improper (numerator is larger than or equal to the denominator), and mixed numbers (a combination of a whole number and a fraction). Common fractions are used extensively to represent ratios, parts of measurements, and mathematical operations.
Numerator and Denominator
- Define the numerator and denominator and explain their relationship.
- Describe different types of fractions: proper, improper, and mixed numbers.
- Show how to convert between fractions and mixed numbers.
What Are Common Fractions: An Adventure into the Realm of Numerators and Denominators
In the mathematical kingdom, fractions reign as essential tools for describing parts of a whole. They’re like little explorers, guiding us through a world where quantities are measured in slices and pieces. Imagine a pizza cut into equal portions, and each slice represents a fraction of the whole pie.
The numerator is the number that tells us how many slices of pizza we have, while the denominator reveals how many slices make up the entire pie. These two values work hand-in-hand to define the fraction. For instance, if we have 3 slices out of a pizza cut into 8 equal parts, the fraction is 3/8. The numerator (3) tells us we have 3 slices, and the denominator (8) indicates the total number of slices in the whole pizza.
Types of Fractions: A Spectrum of Proportions
Fractions come in diverse forms, each with its unique characteristics. Proper fractions reside in the realm where the numerator is smaller than the denominator, indicating a part of the whole that’s less than the entire quantity. Improper fractions, on the other hand, have a numerator that’s larger than the denominator, suggesting a part that’s greater than the whole.
Mixed numbers are a hybrid of fractions and whole numbers, presenting both the whole part and the fractional part in one neat package. They occur when the numerator is too large to fit alone in the fraction, like a puzzle piece that doesn’t quite fit in. To convert a mixed number to a fraction, we multiply the whole number by the denominator and add the numerator. For example, converting the mixed number 1 2/3 to a fraction yields 5/3.
Fractions unlock a world of mathematical adventures, enabling us to divide and conquer quantities, and understand the intricacies of our numerical universe. They’re the building blocks of algebra, calculus, and countless other mathematical disciplines, serving as a gateway to a deeper understanding of the world around us. So, embrace the adventure of fractions, and let them guide you through the labyrinth of numbers and quantities.
Fractions: Demystifying the Concept of Equivalent Fractions
In the realm of mathematics, fractions serve as a cornerstone in describing parts-to-whole relationships. While initially they may seem daunting, understanding equivalent fractions is crucial for mastering this fundamental concept.
Defining Equivalent Fractions: The Concept of Equal Parts
Equivalent fractions represent the same value despite appearing different. Just as a whole pizza cut into 8 slices is equivalent to the same pizza cut into 16 slices, fractions can be divided into smaller or larger parts while maintaining their overall value.
Simplifying Fractions: The Path to Simpler Forms
Simplifying fractions is the process of expressing them in their simplest form, i.e., the form with the smallest possible numerator (top number) and denominator (bottom number). This is achieved by dividing both the numerator and the denominator by their greatest common factor (GCF).
Consider the fraction 6/12. Identifying its GCF of 6, we divide both numbers by 6, resulting in the simplified fraction of 1/2. This represents the same value as 6/12, but in a more concise form.
Equivalence in Action
To demonstrate the equivalence of fractions, you can visualize them as parts of a whole. For example, 2/4 represents two equal parts of a whole divided into four parts. This is equivalent to 1/2, which represents one equal part of the same whole divided into two parts.
Applications in the Real World
Equivalent fractions have numerous real-world applications:
- Measuring Quantities: A cook following a recipe may need to substitute 3/4 cup of flour, but only has 2/4 cup. Knowing that they are equivalent, the cook can use either amount.
- Calculating Percentages: If a company has a staff of 20 employees and 8 of them are male, we can express the proportion of male employees as 8/20 (or 4/10), which simplifies to 2/5.
Fraction Operations: Multiplying, Dividing, Adding, and Subtracting
Fractions, with their intriguing dance of numerators and denominators, hold the secrets to countless mathematical adventures. Understanding how to manipulate fractions is like having a superpower that unlocks a world of calculations. Let’s delve into the enchanting realm of fraction operations, where we’ll explore multiplication, division, addition, and subtraction like never before.
Multiplication of Fractions: A Symphony of Numerators and Denominators
In the captivating world of fractions, multiplication brings harmony to numbers. It’s a simple waltz where numerators and denominators join forces to create a new fraction. The secret lies in multiplying the numerators together and then uniting the denominators in a joyful dance. For instance, the multiplication of 2/3 and 3/4 results in 6/12, which with a magical simplification step, transforms into 1/2. It’s like a puppet show, where fractions dance to the tune of your calculations.
Division of Fractions: A Battle of Titans
Dividing fractions is an epic battle where the numerator and denominator of one fraction valiantly challenge the other. The key here is to flip the second fraction on its head and then multiply. It’s like a knight wielding a sword, conquering the challenge. For instance, dividing 6/7 by 2/3 is a heroic act that results in 6/7 x 3/2, which after some clever algebra, becomes an elegant 9/7.
Addition and Subtraction of Fractions: A Balancing Act
Adding and subtracting fractions require a bit of dexterity, especially when their denominators are different. It’s like juggling numbers, ensuring they all balance out. The secret is to find a common denominator, the lowest common multiple that allows all the fractions to dance together in harmony. Once you have that, it’s a simple matter of adding or subtracting the numerators and keeping the common denominator steady. For example, to add 1/2 and 1/3, you’d find the common denominator of 6, giving you 3/6 and 2/6, which can be effortlessly added to form 5/6.
Mastering these fraction operations is like becoming a mathematical magician, capable of performing calculations with grace and precision. So, let’s embrace these fraction adventures and conquer the world of mathematics one fraction at a time.
Additional Concepts in Fraction Arithmetic
Converting Fractions to Decimals
Decimal notation, a more familiar form for calculations, can be derived from fractions. By dividing the numerator by the denominator, we obtain the equivalent decimal representation. This conversion simplifies arithmetic operations and leverages the power of decimals.
Comparing Fractions
Comparing fractions involves identifying their relative values. Various methods exist for fraction comparison:
- Common Denominator: Convert fractions to equivalent forms with the same denominator. The fraction with the greater numerator has the greater value.
- Cross-Multiplication: Multiply the numerator of the first fraction by the denominator of the second and vice versa. The fraction with the greater product is the greater fraction.
Real-World Applications of Fractions
Fractions play a crucial role in our daily lives, extending beyond mathematical equations:
- Cooking: Recipes often measure ingredients in fractions to ensure precise proportions.
- Time: We divide an hour into 60 minutes and a minute into 60 seconds, represented as fractions and used for scheduling.
- Measurements: In construction and engineering, measurements frequently involve fractions to represent precise dimensions.
- Economics: Fractions are employed in calculating percentages, discounts, and ratios in financial transactions.
- Nature: The Fibonacci sequence, a pattern of numerical relationships that occurs in nature, is based on fractions.
Understanding these additional concepts empowers us to navigate fraction arithmetic with confidence and apply it seamlessly to real-world scenarios.