The Fraction Line: A Separator With Significance
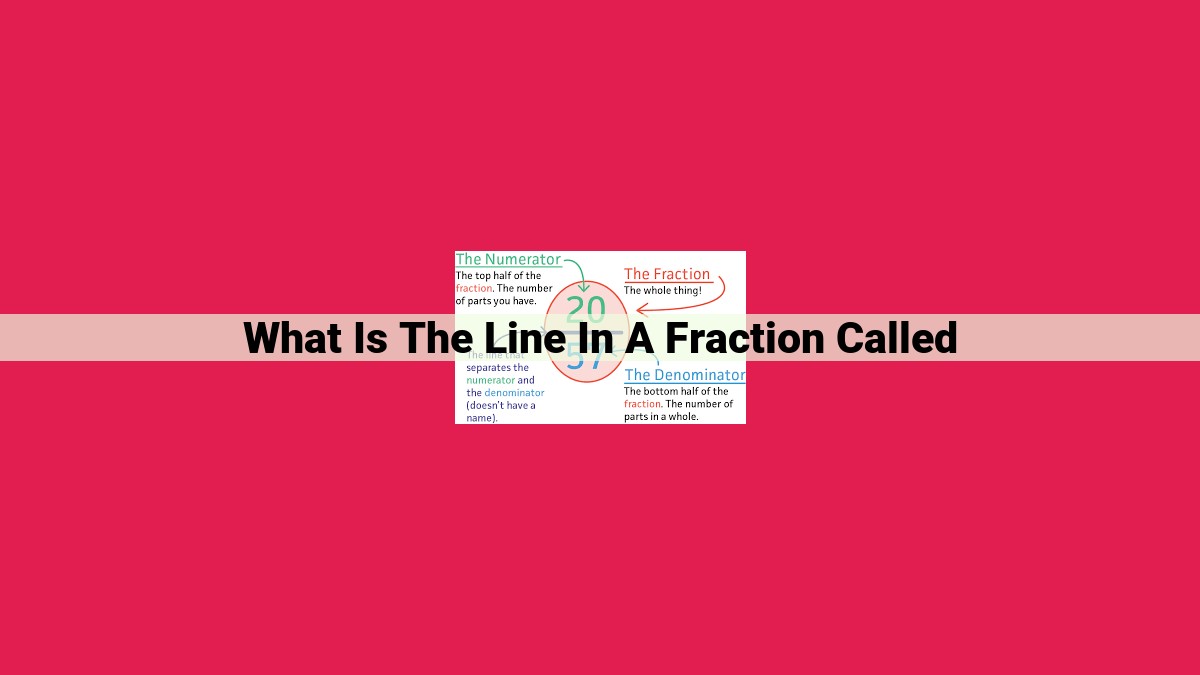
The line in a fraction is called the fraction line. It serves as a separator between the numerator and the denominator, two important parts of a fraction. The numerator is the top number and represents the number of parts being considered, while the denominator is the bottom number and indicates the total number of parts in the whole. The fraction line helps to convey the value of the fraction by visually separating the two numbers and indicating their relationship.
Understanding Fractions: A Beginner’s Guide
What is a Fraction?
Are you still struggling to wrap your head around the concept of fractions? Don’t worry, you’re not alone! Let’s take a step back and delve into the very basics.
A fraction is like a fun math puzzle that helps us describe parts of a whole. Imagine you have a pizza cut into several slices. Each slice represents a fraction of the whole pizza.
The numerator, the number on top, tells us how many slices you have. The denominator, the number on the bottom, tells us how many slices the whole pizza was cut into. So, if you have 1 slice of that pizza cut into 8 slices, the fraction would be 1/8. That means you have one slice out of the eight slices that make up the whole pizza.
Understanding the Fraction Line: A Tale of Division and Proportion
In the world of fractions, the fraction line plays a pivotal role in conveying their meaning and value. It acts as a vivid boundary, separating the numerator from the denominator, and it’s more than just a simple dividing line.
Imagine a pizza party where you’re sharing a pizza with 8 slices. You take 3 slices, and your friend takes the remaining ones. How do you express this distribution mathematically? That’s where the fraction line comes into play.
The numerator, or the top number, tells us how many pieces you have: 3. The denominator, or the bottom number, indicates the total number of pieces in the whole pizza: 8. The fraction line demarcates this division, providing a clear visual representation of the proportion of the pizza you have.
It’s like a measuring tape, the fraction line helps us visualize the size of a fraction in relation to the whole. A fraction with a smaller denominator (like 1/2) indicates a larger proportion of the whole, while a fraction with a larger denominator (like 1/8) signifies a smaller proportion.
Remember, the fraction line is not just a passive observer; it actively communicates the division and proportion inherent in fractions. It’s the visual anchor that allows us to understand and interpret these mathematical building blocks with confidence.
Description of the numerator as the top number
Delving into the Numerator: The Top Number in the Fraction’s Tale
In the realm of fractions, the numerator stands tall as the top number, a key player in this numerical game. Picture this: A pizza, hot and fresh from the oven, is waiting patiently to be sliced and shared. The numerator acts as the intrepid chef, determining the number of slices that will grace your plate.
Let’s take an example to illuminate its role: When we say “1/2,” it means that we have one slice out of a total of two. The numerator, “1,” tells us we have a single slice, while the denominator, “2,” indicates that the pizza has been divided into two equal parts.
The numerator’s job extends beyond mere counting. It also measures the extent to which the fraction represents the whole. In our pizza analogy, the numerator of “1/2” indicates that we have half of the pie. It’s like a fraction of the whole, a glimpse into its magnitude.
So, there you have it, the numerator: The compass guiding us through the numerical landscape, measuring parts and revealing the hidden proportions within fractions. Dive deeper into the world of fractions, and you’ll discover how the numerator and its sidekick, the denominator, work together to paint a vivid picture of numerical relationships.
The Numerator: Measuring the Parts
In the world of fractions, the numerator sits atop the fraction line like a brave knight, showcasing the number of parts under its watchful eye. It’s the valiant warrior that measures the extent of the whole, giving us a glimpse into the size of the puzzle piece.
Imagine a pizza devoured by a hungry family. Each hungry mouth represents a numerator, eagerly counting the delectable slices disappearing into bellies. The numerator stands as a testament to the portions being shared, the slices of joy that bring smiles to faces.
Whether it’s slices of pizza, pieces of a cake, or intervals of time, the numerator acts as our compass, guiding us through the maze of fractions. It’s a beacon of information, telling us how many pieces of the whole we have, like a treasure map leading us to the hidden gold of understanding.
Understanding Fractions: Exploring the Numerator’s Role
What is a Fraction?
Fractions are mathematical symbols used to represent parts of a whole. They consist of two numbers separated by a line: the numerator and the denominator.
The Numerator: Measuring and Counting
The numerator, the top number in the fraction, tells us how many equal parts of the whole we’re dealing with. For instance, in the fraction 3/5, the numerator 3 indicates that we have three equal parts.
Examples and Applications of the Numerator
Numerators play a crucial role in various everyday scenarios. Consider a pizza cut into 8 equal slices. If you take 3 slices, the fraction 3/8 describes the amount of pizza you have, which is three out of the eight total slices.
Another example is measuring ingredients in a recipe. If a recipe calls for 1/2 cup of flour, the numerator 1 tells us we need one part of flour. The denominator 2 indicates that the flour is divided into two equal parts.
Understanding numerators helps us quantify and compare quantities in numerous areas, from cooking and baking to construction and scientific experiments. By grasping the concept of the numerator, we can accurately measure, count, and determine the amount of any given portion or part.
The Denominator: The Foundation of Proportionality
In the realm of fractions, the denominator emerges as the bottom number that plays a pivotal role in the division and proportioning of the whole. It stands as a representative of the total number of parts into which a whole is divided.
Imagine a delicious pizza, freshly baked and ready to be shared. The denominator in this culinary context represents the number of slices into which this celestial culinary creation is divided. For a pizza cut into eight equal portions, the denominator would proudly proclaim itself as “8.”
The denominator’s significance extends beyond pizza distribution. It holds sway over the equivalence of fractions. When fractions share the same denominator, their proportionality becomes evident. Just as a pizza with eight slices can be divided equally among four friends, a fraction with a denominator of 8 can be split into equal parts to represent different proportions.
For instance, the fraction 2/8 implies that two out of the eight slices of pizza belong to a particular friend, while 4/8 suggests that half of the pizza is reserved for another hungry soul. The denominator, like a watchful guardian, ensures that these proportions remain harmonious and that each slice finds its rightful owner.
Moreover, the denominator’s presence lends itself to a deeper understanding of fractions as ratios. By indicating the total number of parts, the denominator allows us to compare fractions and determine their relative sizes. A fraction with a larger denominator represents a smaller portion of the whole, while fractions with smaller denominators represent larger portions.
In the grand scheme of mathematics, fractions serve as indispensable tools for solving problems and making informed decisions. Understanding the denominator’s profound role in dividing, proportioning, and establishing equivalence empowers us to navigate the world of numbers with confidence and precision.
The Denominator: Dividing and Proportioning
The denominator, the bottom number in a fraction, plays a crucial role in understanding the value of a fraction. It serves as a dividing line, separating the total number of parts represented by the fraction. For instance, in the fraction 1/2, the denominator 2 tells us that the whole has been divided into two equal parts.
The denominator also indicates proportionality. It determines the relative size of the fraction compared to a whole or other fractions. For example, in the fraction 1/3, the denominator 3 indicates that the fraction represents one out of three equal parts. This means that the fraction is smaller than a whole and larger than 1/4, which represents one out of four equal parts.
Understanding the denominator’s role in dividing and proportioning is essential for interpreting fractions accurately. By recognizing that the denominator represents the total number of parts and determines the relative size of the fraction, we can better grasp the value and significance of fractions in both mathematical and real-world contexts.
The Denominator: The Key to Proportional Understanding
In the realm of fractions, the denominator plays a pivotal role, acting as the gatekeeper to the concept of proportionality. Proportionality is the idea that two quantities change in a consistent ratio as one varies. This fundamental principle governs a myriad of phenomena in the physical and social world.
Picture this: you’re baking a cake. The recipe calls for a specific ratio of flour to sugar. If you increase the amount of flour by a factor of two, you must also proportionally increase the sugar by the same amount to maintain the desired sweetness. The denominator of the fraction representing the flour-to-sugar ratio dictates this proportionality.
The denominator represents the total number of parts into which a whole is divided. By determining this total, the denominator sets the stage for understanding how the different parts relate to each other. In the cake analogy, if flour is divided into two equal parts (denominator = 2), then sugar must also be divided into two equal parts (denominator = 2).
The magic lies in the inverse relationship between the denominator and the fraction’s value. As the denominator increases, the value of the fraction decreases, and vice versa. This is because a larger denominator indicates a greater number of parts, resulting in a smaller share for each part. Conversely, a smaller denominator implies fewer parts, giving each part a larger share.
By grasping the proportional nature of fractions, we empower ourselves to solve problems and make informed decisions. We can adjust quantities, maintain ratios, and predict outcomes with greater accuracy. The denominator, though seemingly humble, is the unsung hero that unlocks the true power of fractions in our mathematical toolkit.
Unveiling the Fraction Line: A Gateway to Mathematical Understanding
In the realm of numbers, the fraction line serves as a pivotal bridge connecting the numerator and denominator, two indispensable elements that collectively define the essence of a fraction. This humble line, though often overlooked, plays a profound role in elucidating the underlying concepts of measurement, proportion, and mathematical visualization.
Like a delicate thread in a tapestry, the fraction line demarcates the boundary between the numerator and denominator, each with its own unique purpose. The numerator, nestled above the line, measures the quantity under consideration, whether it be a part of a whole or a specific number of objects. The denominator, residing below the line, portions the whole, establishing the total number of equal parts into which the whole is divided.
Together, the numerator and denominator work harmoniously through the fraction line to convey the value of the fraction. This value represents the relationship between the part and the whole, allowing us to quantify and compare fractions with ease. The fraction line acts as a visual cue, enabling us to grasp the concept of proportion and understand how fractions relate to each other.
For instance, consider the fraction 1/2. The numerator, 1, tells us that we have one part. The denominator, 2, indicates that the whole is divided into two equal parts. The fraction line separates the numerator and denominator, clearly showcasing that we have one part out of a total of two parts. This visual representation makes it evident that 1/2 represents half of the whole, a concept that would be much harder to infer without the fraction line.
Thus, while seemingly simple, the fraction line is an indispensable element that plays a pivotal role in our understanding of fractions. By separating the numerator and denominator, the fraction line illuminates the concepts of measurement, proportion, and visualization, empowering us to explore the fascinating world of fractions with clarity and confidence.
The Fraction Line: A Story of Separation and Understanding
In the realm of fractions, the fraction line emerges as a pivotal character, a silent guardian whose role is to separate and delineate the two worlds of the numerator and the denominator. While these two numbers stand apart, their connection remains intertwined, forming the very essence of a fraction.
The fraction line serves as a demarcation, a clear boundary between the numerator and the denominator. It separates the finite world of the numerator from the expansive universe of the denominator, allowing each to retain its distinct identity. Without this separation, the fraction would lose its meaning, becoming merely a collection of numbers without purpose or coherence.
Furthermore, the fraction line acts as a visual cue, a visual representation of the fraction’s value. Just as a line can divide a circle into equal parts, the fraction line divides the whole unit into equal fractions. By observing the position of the numerator and denominator in relation to the line, we can instantly perceive the relative size of the fraction and its relationship to the whole.
This ability to convey the fraction’s value makes the fraction line an invaluable tool in problem-solving and decision-making. It allows us to compare fractions, determine their equivalence, and perform mathematical operations with greater ease. Without the fraction line, these tasks would be far more complex and time-consuming.
In essence, the fraction line plays a crucial role in the world of fractions. It separates the numerator and denominator, providing clarity and definition. It visualizes the fraction’s value, making it easier to grasp and manipulate. And ultimately, it empowers us to navigate the fascinating world of fractions with confidence and comprehension.
Visualizing the Fraction Line: Making Fractions Tangible
The fraction line is not merely a separator; it’s a visual representation that brings fractions to life. It’s the boundary that demarcates and illustrates the relationship between the numerator and denominator.
Imagine chopping a pizza into eight equal slices. If you eat two slices, you’ve consumed two-eighths of the pizza. The fraction line in 2/8 serves as a visual aid, showing that you have two shaded slices (numerator) out of a total of eight slices (denominator).
This visualization helps you grasp the fraction’s magnitude and relationship to other fractions. For example, you can easily see that 2/8 is smaller than 3/8, as there are fewer shaded slices in the fraction with a smaller numerator.
By internalizing the fraction line as a visual cue, you can instantly comprehend the value and comparative size of fractions. It becomes a cognitive tool that empowers you to make informed judgments and solve problems involving fractions.
Fractions: The Building Blocks of Everyday Life
In the tapestry of our daily lives, fractions play an intricate role, often unnoticed but ever-present. They weave their way into our conversations, our decision-making, and even the foods we savor.
Shopping and the Supermarket Adventure
As you navigate the supermarket aisles, fractions guide your choices. The bag of rice you select may be labeled 3/4 cup, indicating that it will feed three-fourths of your hungry family. Similarly, the juicy watermelon you pick up might display a weight of 1/2 pound, informing you of its delectable half-pound proportions.
Cooking and the Culinary Canvas
In the culinary realm, fractions are indispensable. When following a recipe for your favorite chocolate chip cookies, you may encounter instructions like 1/2 cup of brown sugar or 1/4 teaspoon of vanilla extract. These precise measurements ensure that your cookies turn out perfectly balanced and tantalizingly delicious.
Time and the Unseen Flow
Time itself is often measured in fractions. We divide an hour into 60 minutes and further subdivide minutes into seconds (1/60 of a minute). This precise division helps us schedule our appointments, plan our commutes, and savor every moment of our days.
Sports and the Thrill of Competition
In the world of sports, fractions play a pivotal role in determining winners and losers. The yardage gained in football or the batting average in baseball are often expressed as fractions. These precise calculations tell us not only who performed better but also by how much.
Medicine and the Precision of Healing
In the realm of medicine, fractions are essential for precise dosage calculation. Medications are often prescribed in milligrams** (1/1000 of a gram) or *micrograms** (1/1,000,000 of a gram*). These precise measurements ensure that patients receive the correct and effective amount of medication.
By understanding the role of fractions in everyday life, we gain a deeper appreciation for their significance. They are the building blocks of our conversations, decisions, and experiences. So, let’s embrace fractions and see them not as obstacles but as tools that help us navigate the world around us.
Fractions: A Key to Problem-Solving and Decision-Making
From the kitchen to the classroom, fractions are an integral part of our daily lives. They help us measure ingredients, calculate distances, and make informed decisions. By understanding the concepts behind fractions, we unlock a powerful tool for solving problems and navigating the world around us.
The Numerator’s Role in Problem-Solving
The numerator tells us how many parts we have. It’s the top number in a fraction, and it represents the part of the whole that we’re considering. For example, in the fraction 2/5, the numerator 2 indicates that we have two parts.
The Denominator’s Role in Decision-Making
The denominator tells us how many equal parts make up the whole. It’s the bottom number in a fraction, and it helps us compare the size of different fractions. For instance, in the fraction 2/5, the denominator 5 shows that the whole has been divided into five equal parts.
The Fraction Line: A Visual Divide
The fraction line is more than just a separator; it’s a visual representation of the relationship between the numerator and denominator. It shows us that the numerator is part of the whole represented by the denominator. By understanding this visual relationship, we can easily compare and manipulate fractions.
Fractions in Everyday Life
The importance of understanding fractions extends beyond the classroom. They are essential in many real-world applications, including:
- Cooking: Measuring ingredients, calculating serving sizes
- Construction: Calculating materials and estimating costs
- Finance: Understanding loan payments, interest rates
- Medicine: Prescribing dosages, interpreting test results
Fractions are an indispensable tool for problem-solving and decision-making. By grasping the concepts of the numerator, denominator, and fraction line, we can unlock their power to understand the world around us. From baking a perfect cake to making informed financial choices, fractions play a vital role in our daily lives.
Understanding Fractions: The Numerator and Denominator as Quantity Representatives
Fractions, often encountered in everyday life, are mathematical tools that represent parts of a whole, or the ratio between two quantities. To delve into the world of fractions, let’s explore the roles played by its two key components: the numerator and the denominator.
The Numerator: Measuring and Counting
The numerator is the top number of a fraction, indicating the number of equal parts being considered. It serves as a measurement of the quantity represented by the fraction. For example, in the fraction 3/5, the numerator 3 tells us that we are dealing with three out of the five equal parts of the whole.
The Denominator: Dividing and Proportioning
The denominator is the bottom number of a fraction, representing the total number of equal parts into which the whole is divided. Its function is to determine the proportion of the whole that the numerator represents. Continuing with the example of 3/5, the denominator 5 shows that the whole has been divided into five equal parts, and the numerator 3 indicates that we are considering three of those parts.
The Interplay of Numerator and Denominator
Together, the numerator and denominator provide a comprehensive representation of a quantity. By visualizing the fraction line as a separator, we can interpret the fraction as a ratio of the numerator to the denominator. In other words, the fraction 3/5 can be understood as the ratio of 3 to 5, representing a three-fifths portion of the whole.
Contextualizing the Concepts: Applications in Real Life
Fractions find widespread application in various aspects of our lives. From measuring ingredients in cooking to calculating percentages and understanding ratios in science, fractions play a crucial role in problem-solving and decision-making. By grasping the concepts of the numerator and denominator, we gain a deeper understanding of how quantities can be represented and compared.
In summary, the numerator and denominator are the essential building blocks of fractions, serving as measurement and proportioning tools. Their interplay allows us to represent and compare quantities precisely, making fractions indispensable in various practical applications. Understanding these concepts unlocks a gateway to mathematical literacy and empowers us to navigate a world where fractions are prevalent.