Optimized Seo Title:find Midpoint Of Line Segment Easily: Step-By-Step Guide Using Midpoint Formula
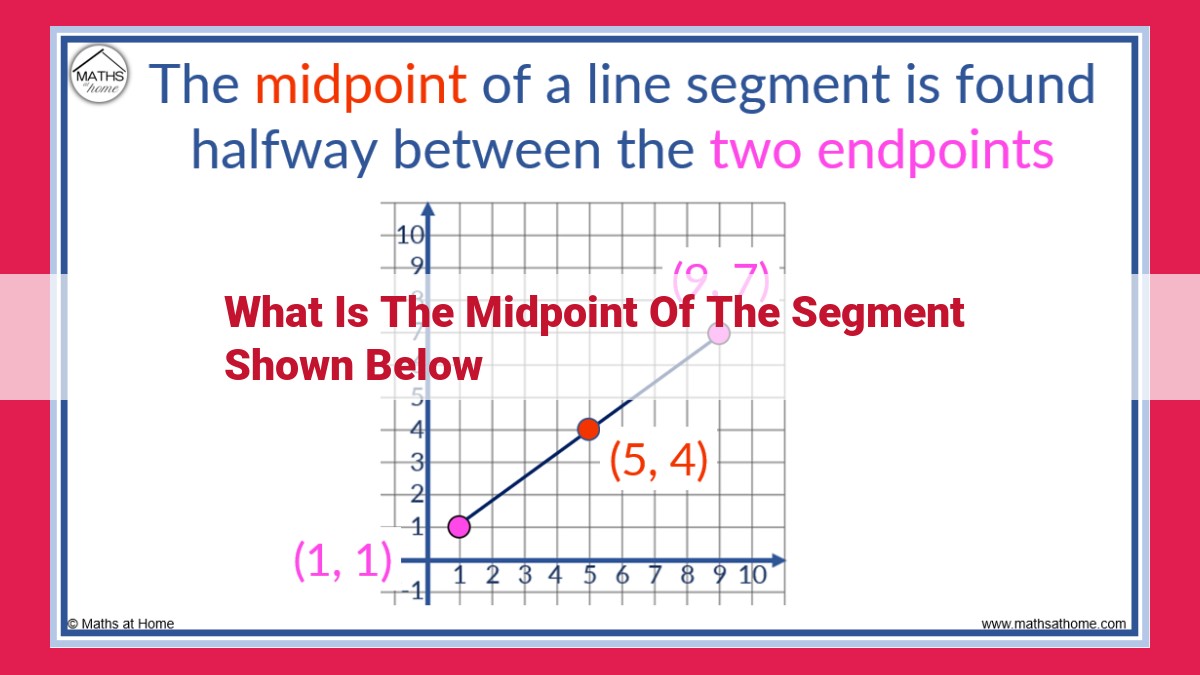
The midpoint of a line segment divides the segment into two equal parts. In coordinate geometry, the midpoint can be found using the midpoint formula, which averages the x-coordinates and y-coordinates of the endpoints of the segment. For example, if the endpoints are (x1, y1) and (x2, y2), the midpoint is ((x1 + x2)/2, (y1 + y2)/2). The midpoint is an important concept in geometry as it provides a way to accurately locate the center or balancing point of a line segment.
The Midpoint of a Line Segment: A Geometric Adventure
In the world of geometry, lines play a crucial role in shaping our understanding of space. Imagine a straight line, stretching out endlessly like a journey unfolding before you. Along this line, lies a special point that divides it into two perfectly equal parts. This magical point is known as the midpoint.
The midpoint holds a unique position on a line segment. It partitions the segment into identical halves, creating a perfect balance. This property is what makes the midpoint a key geometric landmark, allowing us to calculate distances and understand line relationships.
To grasp the concept of the midpoint fully, we need to delve into the fascinating world of coordinate geometry. By introducing a grid system of intersecting lines, we can describe points on a plane using their coordinates. These coordinates, like a map, provide us with precise locations within the geometric landscape.
Armed with this knowledge, we can unlock the secrets of the distance formula, a mathematical tool that allows us to measure the distance between any two points on the plane. The formula, derived from the iconic Pythagorean theorem, empowers us to calculate line segment lengths with precision.
The slope of a line segment, its steepness, can also be determined using coordinate geometry. Slope measures the slant of a line relative to the horizontal axis. By calculating the coordinate difference between two points on the line, we can determine its slope, which provides valuable insights into the line’s orientation.
Finally, we can combine the concepts of distance, slope, and coordinates to derive the midpoint formula for line segments. This formula, a mathematical gem, allows us to find the exact coordinates of the midpoint, given the coordinates of the line segment’s endpoints. It is a powerful tool for геометрические задачи, providing a shortcut to determining the midpoint’s location.
So, dear explorers of geometry, embrace the concept of the midpoint. It is a beacon of balance, a key to understanding line relationships, and a testament to the power of mathematics in unraveling the secrets of space.
The Wonders of Coordinate Geometry: Unlocking the Secrets of the Mathematical Plane
In the realm of mathematics, coordinate geometry emerges as a fascinating tool that enables us to explore the enigmatic world of the coordinate plane. Imagine a vast expanse, where every point can be uniquely identified by its x and y coordinates. This grid-like system provides a precise roadmap for navigating this geometric landscape.
At its heart, coordinate geometry revolves around the concept of a coordinate system. It consists of two perpendicular number lines, the x-axis and the y-axis, which intersect at a point called the origin. The x-axis runs horizontally, while the y-axis extends vertically, creating a two-dimensional space that can accommodate an infinite number of points.
Each point on the coordinate plane is represented by an ordered pair of numbers, enclosed in parentheses: (x, y). The first number, x, indicates the point’s horizontal distance from the origin, while the second number, y, denotes its vertical distance. For instance, the point (3, 5) is located three units to the right of the origin and five units above it.
The coordinate system plays a crucial role in describing the location and relationships between points on the plane. By assigning coordinates to points, we can precisely locate them, measure distances, and determine their slopes. This geometric framework unlocks a wealth of possibilities for exploring and understanding the intricacies of the mathematical plane.
Unraveling the Distance Formula: A Journey into the Heart of Geometry
In the tapestry of geometry, the distance formula stands as a crucial thread, connecting the dots of our coordinate plane. It empowers us to measure the gap between any two points, opening up a realm of possibilities for exploring relationships and deciphering shapes.
At the heart of this formula lies the Pythagorean theorem, an ancient truth that underpins our understanding of right-angled triangles. It states that the square of the hypotenuse (the longest side of the triangle opposite the right angle) is equal to the sum of the squares of the other two sides.
In our coordinate plane, we can harness this theorem to calculate the distance between two points. Picture a ladder leaning against a wall, with the rungs parallel to the ground. The vertical distance from the bottom of the ladder to the wall represents the y-coordinate of the point, while the horizontal distance from the wall to the base of the ladder represents the x-coordinate.
Now, let’s say we have two points: A(x1, y1) and B(x2, y2). Using the Pythagorean theorem, we can derive the distance formula:
Distance = √((x2 - x1)² + (y2 - y1)²)
This formula essentially calculates the length AB using the difference between the x-coordinates and the difference between the y-coordinates, then squaring and summing these differences before taking the square root. It’s a powerful tool for measuring line segments in coordinate geometry, revealing the intricacies of our two-dimensional world.
Understanding Slope: A Journey into the World of Lines
In the realm of geometry, lines are like dancers gracing the stage, each with its own unique personality. A key characteristic that distinguishes one line from another is its slope, a number that captures the line’s inclination or steepness.
Visualizing Slope:
Imagine a line cutting through a coordinate plane. The slope of this line is the ratio of the vertical change (difference in y-coordinates) to the horizontal change (difference in x-coordinates) between any two distinct points on the line. If the line rises from left to right, it has a positive slope. If it falls from left to right, its slope is negative.
Measuring Slope:
Calculating the slope of a line is surprisingly simple. Let’s say we have two points on the line, (x1, y1) and (x2, y2). The slope formula is:
slope = (y2 - y1) / (x2 - x1)
This formula gives us a single number that describes the steepness of the line. The greater the value of the slope, the steeper the line.
Applications of Slope:
The slope of a line has numerous applications in various fields:
- Describing Motion: In physics, slope represents velocity or acceleration.
- Grading Systems: In education, slope measures the difficulty of a course.
- Finance: In economics, slope shows the relationship between stock prices and time.
Importance in Coordinate Geometry:
In coordinate geometry, the slope of a line plays a crucial role in determining:
- Parallel and Perpendicular Lines: Lines with the same slope are parallel; lines with slopes that are negative reciprocals of each other are perpendicular.
- Equations of Lines: The slope and y-intercept of a line are used to write its equation.
By understanding slope, we can unlock a deeper understanding of lines and their behavior in the mathematical world. Let’s explore the other concepts in our outline to complete our journey into the fascinating world of coordinate geometry.
The Midpoint Formula: Unveiling the Secrets of Line Segments
In the vast tapestry of geometry, the midpoint of a line segment stands out as a beacon of symmetry and balance. But how do we pinpoint this elusive geometrical gem? Enter the midpoint formula, a mathematical tool that unlocks the secrets of line segments.
The Coordinate Difference and Average Method
Visualize a line segment stretching from point A(x1, y1) to point B(x2, y2). The midpoint of this segment, let’s call it M, will be located exactly halfway along its length. To find M, we can use the coordinate difference and average method.
First, we calculate the horizontal difference (x2 – x1) and the vertical difference (y2 – y1) between points A and B. Then, we simply average these differences to find the coordinates of the midpoint:
Midpoint M = ( (x2 - x1) / 2, (y2 - y1) / 2 )
For instance, if A is (3, 5) and B is (7, 13), the midpoint M would be ((7 – 3) / 2, (13 – 5) / 2) = (2, 4).
Deriving the Midpoint Formula: Geometry and Algebra Unite
The midpoint formula can also be derived from a blend of geometry and algebra. Imagine the line segment AB as a diagonal in a parallelogram with vertices at A, B, and two other points, C and D. Let’s focus on the midpoint M, which will also be the intersection point of the parallelogram’s diagonals.
Since the diagonals of a parallelogram bisect each other, we have AM = MB and CM = MD. Using the distance formula, we can calculate the lengths of AM and CM:
AM = sqrt((x2 - x1)^2 + (y2 - y1)^2) / 2
CM = sqrt((x1 - x2)^2 + (y1 - y2)^2) / 2
Setting AM = CM and simplifying, we arrive at the midpoint formula:
(x2 - x1) / 2 = (x1 - x2) / 2
(y2 - y1) / 2 = (y1 - y2) / 2
Hence, by harnessing the power of geometry and algebra, we have unveiled the secrets of the midpoint formula.