Comprehensive Guide To Integrating Exponential Functions: Essential Techniques For Advanced Calculus
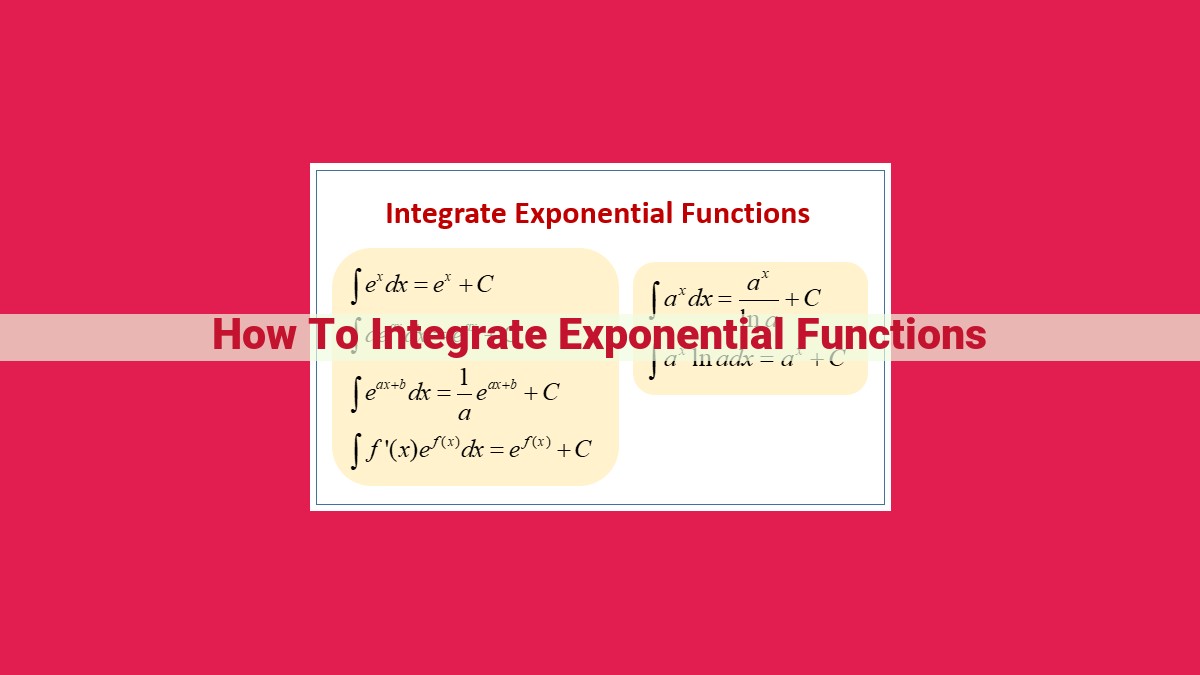
Integrating exponential functions involves several methods. The substitution method replaces the exponential term with a new variable, allowing for integration using standard techniques. Integration by parts divides the integral into two parts and repeatedly applies the product rule in reverse. Logarithmic integration utilizes the relationship between logarithms and exponentials to transform the integral. Reduction formulas simplify integrals of the form ∫xe^x dx, while partial fractions decomposition breaks down rational functions involving exponential functions into simpler partial fractions for integration.
Definition and explanation of the substitution method.
Integrating Exponential Functions: A Journey Through Mathematical Mastery
In the realm of mathematics, integrals represent the area under a curve, revealing essential information about functions. When it comes to exponential functions, finding their integrals requires an array of techniques that unveil their hidden secrets.
1. Substitution Method: A Tale of Transformative Trickery
The substitution method stands as a clever strategy for conquering exponential integrals. It involves introducing a cunning alias, a new variable, to disguise the exponential expression as a more tractable form. For instance, let’s consider the integral of e^(2x) dx. By substituting u = 2x, we effectively transform the exponential function into a linear term e^u. Solving the integral with respect to u, we can then back-substitute to arrive at the desired solution.
This method shines when the exponential function is nested within a more complex expression, offering a surgical path to unraveling its complexities. Just as a magician’s sleight of hand astounds, the substitution method seamlessly transforms daunting integrals into manageable equations.
2. Integration by Parts: A Symphony of Harmonious Cooperation
Integration by parts arises as a harmonious collaboration between two functions, orchestrating a dance of derivatives and integrals. For exponential functions, this partnership holds the key to uncovering their hidden areas. By partitioning the exponential function into two parts, u and dv, we employ a specific formula to transform the integral into a chain of derivatives and integrals.
The art of selecting u and dv lies in identifying the functions with suitable characteristics for differentiation and integration. Common choices include trigonometric functions, such as sine and cosine, and exponential functions themselves. Through this deft interplay, integration by parts unlocks the mysteries of exponential integrals with the grace of a conductor leading an orchestra.
3. Logarithmic Integration: A Revelation Unveiled
Logarithmic integration unveils a profound connection between two seemingly disparate mathematical entities: integrals and logarithms. This method emerges as the secret weapon for tackling integrals of the form (ln x)^n dx. By applying the inverse function theorem and a dash of mathematical finesse, we derive an elegant formula that transforms the integral into a logarithmic expression.
This revelation unveils the intricate partnership between logarithmic and exponential functions, revealing their shared essence and interconnected nature. Through this understanding, we conquer integrals involving exponential functions with the same ease as a sculptor chiseling a masterpiece from raw stone.
Integrating Exponential Functions: A Comprehensive Guide
In the realm of calculus, learning to integrate exponential functions is like unlocking a secret door to a fascinating world. And like any quest, there are various paths to this destination. Let’s embark on a journey to uncover the most common techniques for integrating exponential functions.
1. Substitution Method: The Art of Disguise
Imagine you have an integral that looks like a mysterious equation: ∫e^(2x) dx. How do you tame this beast? The substitution method comes to the rescue. Think of it as putting on a disguise to trick the integral into revealing its secrets.
Let’s define u = 2x. Now, du/dx = 2, which means dx = 1/2 du. Substituting, we get:
∫e^(2x) dx = ∫e^u (1/2 du)
Now, the integral becomes a familiar form: 1/2 ∫e^u du. And voilà! Integration complete.
2. Integration by Parts: Divide and Conquer
Picture this: You’re trying to integrate ∫xe^x dx. Direct integration seems like a dead end. But here’s where integration by parts shines. It’s like breaking down a puzzle into smaller, manageable pieces.
Let’s define u = x and dv = e^x dx. Then, du/dx = 1 and v = e^x. Substituting, we get:
∫xe^x dx = x e^x - ∫e^x dx
And there you have it! We’ve conquered that tricky integral by breaking it down into simpler steps.
3. Logarithmic Integration: A Mathematical Cinderella Story
Sometimes, an integral like ∫1/x dx seems impossible to solve. But with logarithmic integration, it becomes a fairy tale.
Let’s define u = 1/x. Then, du/dx = -1/x^2 and ln|u| = ln|1/x| = -ln|x|. Substituting, we get:
∫1/x dx = ∫du (-ln|x|)
And voila! The integral transforms into a form we can handle.
Integrating Exponential Functions: A Comprehensive Guide
In the realm of mathematics, exponential functions hold a prominent place. Their distinctive form, with a variable in the exponent, presents a unique challenge when it comes to finding integrals. However, with a few clever techniques, we can unlock the secrets of integrating exponential functions and conquer this mathematical enigma.
One such technique is integration by parts. This method involves breaking down an integral into two parts, one of which can be easily integrated and the other involving an exponential function. By multiplying one part by a suitable derivative and the other by the original function and then integrating by parts, we can cleverly convert the exponential integral into a more manageable form. This technique is particularly effective when the integrand involves a trigonometric or exponential function.
For instance, consider the integral ∫xe^x dx. Using integration by parts with u = x and dv = e^x dx, we get du = dx and v = e^x. Substituting these values, we obtain:
∫xe^x dx = x e^x - ∫e^x dx
The remaining integral, ∫e^x dx, is a straightforward exponential integral that can be evaluated as e^x + C. Thus, the final result becomes:
∫xe^x dx = x e^x - e^x + C
By applying integration by parts, we have transformed a seemingly complex exponential integral into a more manageable form. This technique is a powerful tool that can help us solve a wide range of integrals involving exponential functions.
**Integrating Exponential Functions: A Comprehensive Guide**
In the realm of calculus, exponential functions stand tall as mathematical powerhouses, often encountered in various scientific and engineering applications. Understanding how to integrate exponential functions is a crucial skill for navigating these complex landscapes. This article will delve into the world of exponential integration, exploring five key methods that will empower you to conquer these integrals with ease.
**2. Integration by Parts: Breaking Down the Exponential Barrier**
Integration by parts is a fundamental technique that shines when faced with the challenge of integrating products of functions. To master this technique, let’s venture into a storytelling realm.
Imagine yourself as a master detective embarking on a mission to unravel the secrets of integrating exponential functions using integration by parts. You begin by identifying two key players: a clever “u” function and an elusive “dv” function. The “u” function holds the exponential beauty, while the “dv” function embodies the essence of integration.
Like a skilled pianist, you press the first key of the integration process, substituting your chosen “u” function into the integral. However, this substitution is not merely a cosmetic change; it transforms the integral into a duet between “u” and “dv,” where the derivative of “u” and the integral of “dv” harmoniously combine.
To select the perfect “u” and “dv” functions, remember the golden rule of integration by parts: choose functions that make both the integral of “dv” and the derivative of “u” manageable. For exponential functions, common suspects for “u” include logarithmic functions, while “dv” often disguises itself as an exponential or polynomial function.
With the right functions selected, the integration by parts formula reveals its true elegance. It transforms the daunting integral of a product into a sum of simpler integrals, each one a step closer to the ultimate solution. It’s like watching a complex puzzle seamlessly come together, piece by piece.
So, the next time you encounter an exponential integral, don’t shy away. Embrace the power of integration by parts, and let it guide you to a realm where exponential functions become mere pawns in your mathematical game.
Integrating Exponential Functions: A Journey into Mathematical Mastery
Imagine yourself as an intrepid mathematician, embarking on a quest to unravel the mysteries of integrating exponential functions. Our trusty guide, this comprehensive blog post, will illuminate your path with five time-tested techniques:
- The Substitution Method:
Like a skilled magician, this method allows us to transform our exponential function into a simpler form. By introducing a new variable, we can wield the power of substitution to conquer integrals like these:
∫ e^(2x+3) dx
- Integration by Parts:
Next, we invite integration by parts to our team. Similar to a waltz, we harmoniously pair an exponential function with a partner function. By strategically choosing this partner, we can effortlessly compute integrals like so:
∫ x*e^(3x) dx
- Logarithmic Integration:
Unleash the power of logarithms! This technique grants us the ability to tame integrals involving exponential functions with ease. Like a secret decoder ring, we apply the formula:
∫ e^(ln(x)) dx = x
to illuminate hidden patterns within integrals like these:
∫ e^(2ln(x)) dx
- Reduction Formula:
Introducing the reduction formula, a time-saving superhero. This technique empowers us to efficiently solve integrals of the form:
∫ xe^x dx
With each reduction, we move one step closer to victory, revealing the hidden truth within these integrals.
- Partial Fractions Decomposition:
Last but not least, partial fractions decomposition emerges as a sophisticated tool for conquering rational functions. This technique breaks down complex functions into simpler parts, allowing us to integrate functions like these:
∫ (x+1)/(x^2+2x+1) e^(x) dx
with finesse and precision.
Through these techniques, we embark on a journey of mathematical exploration, unlocking the secrets of exponential functions. Embrace the role of a mathematical adventurer and conquer these integrals with confidence. May your mathematical prowess soar to new heights!
Integrating Exponential Functions: A Comprehensive Guide
Logarithmic Integration
Integrating exponential functions can be a daunting task, but logarithmic integration provides a powerful tool for conquering these challenges. This technique transforms an integral that involves an exponential function into one that involves a logarithmic function, which is often easier to solve.
The key to logarithmic integration is the following formula:
∫ e^u du = e^u + C
where u is any differentiable function of x.
To use logarithmic integration, we first identify a suitable function u such that our exponential function can be written as e^u. This is usually achieved by using algebraic manipulations or trigonometric identities.
For example, consider the integral:
∫ e^(2x + 1) dx
We can let u = 2x + 1. Then, du/dx = 2, and we have:
∫ e^(2x + 1) dx = ∫ e^u (du/2) = (1/2) e^u + C = (1/2) e^(2x + 1) + C
Applications
Logarithmic integration is particularly useful for evaluating integrals involving exponential functions that are multiplied by polynomial or trigonometric functions. For instance, we can use it to solve:
∫ x e^(x^2) dx
By letting u = x^2, we get:
∫ x e^(x^2) dx = ∫ e^u (du/2x) = (1/2x) e^u + C = (1/2x) e^(x^2) + C
Logarithmic integration can also be used to evaluate improper integrals involving exponential functions. These integrals typically do not have a closed-form solution, but logarithmic integration can provide an approximation or bound.
Integrating Exponential Functions: A Comprehensive Guide
Introduction
Integrating exponential functions is a fundamental skill in calculus. This comprehensive guide will delve into five methods to help you conquer these integrals with ease: substitution, integration by parts, logarithmic integration, reduction formulas, and partial fractions decomposition.
1. Substitution Method
When you encounter an exponential function of the form e^(ax), the substitution method offers a straightforward solution. Substitute u = ax and compute du/dx = a. The integral becomes 1/a * ∫e^u du, which simplifies to 1/a * e^u + C. Back-substituting u = ax yields the final result.
2. Integration by Parts
Integration by parts is an effective technique for integrating exponential functions multiplied by other functions. The formula is given by ∫u dv = uv – ∫v du. Identify u as the function containing the exponential term and dv as the derivative of the other function.
3. Logarithmic Integration
For integrals of the form ∫e^(f(x)) dx, logarithmic integration shines. Let u = f(x). Then du/dx = f'(x), and the integral becomes ∫e^u * 1/f'(x) du. Using the natural logarithm, we get ln(|u|) + C, where C is the constant of integration.
4. Reduction Formula
Integrals of the form ∫xe^x dx can be solved using a reduction formula. By integration by parts, we derive a formula that reduces the power of x in the integrand. This process can be repeated until we reach an integral that can be solved directly.
5. Partial Fractions Decomposition
Partial fractions decomposition is a powerful tool for integrating rational functions, which can involve exponential functions. By decomposing the rational function into simpler partial fractions, we can integrate each fraction separately.
Mastering Exponential Integrals
By mastering these five methods, you will equip yourself with a robust toolkit for integrating exponential functions. Remember to choose the method that best suits the specific integral you are working on. With practice and perseverance, you will become adept at tackling these integrals with confidence.
Integrating Exponential Functions: Unlocking Mathematical Secrets
Integrating exponential functions is a crucial skill in the mathematical toolkit, unlocking a world of complex integrals. This blog post will embark on a storytelling journey, guiding you through the depths of five powerful integration techniques: the substitution method, integration by parts, logarithmic integration, reduction formulas, and partial fractions decomposition.
Logarithmic Integration: A Path to Exponential Integrals
Among these techniques, logarithmic integration shines brightly as a savior for integrals involving exponential functions. Its formula, ∫ e^u du = e^u + C, where C is the constant of integration, empowers us to solve integrals of the form ∫ f(x)e^x dx. Here, we take the exponential function as our “u” and its derivative, e^x, as our “dv”. By skillfully applying this formula, we can unravel the mysteries of exponential integrals and conquer their complexities.
Applications in Real-World Scenarios
Logarithmic integration finds immense value in practical applications. It finds its place in fields like physics, where it solves integrals modeling radioactive decay. It aids in calculating integrals related to population growth in biology and unravels the complexities of financial integrals in economics.
Integrating exponential functions is a captivating mathematical adventure. With the substitution method, integration by parts, logarithmic integration, reduction formulas, and partial fractions decomposition as our trusty tools, we can conquer any exponential integral that stands in our path. Embrace the storytelling approach of this blog post and let the world of exponential integration unfold before your very eyes.
Step-by-Step Guide to Integrating Exponential Functions with Flair
In the fascinating realm of calculus, where numbers waltz and functions enchant, lies the tantalizing challenge of integrating exponential functions. Fear not, intrepid explorers! With a dash of storytelling and a pinch of mathematical magic, we’ll unveil the secrets to conquering these enigmatic equations.
Method 1: Substitution Magic
Imagine the substitution method as a sorcerer’s spell, transforming complex integrals into simpler ones. We cleverly substitute a part of the function with a new variable, unleashing the power of integration to solve the original equation.
Method 2: Integration by Parts
Integration by parts is a tango of two functions, the “u” and the “dv.” Like a skilled dancer, we carefully identify the appropriate functions and perform a delightful sequence of differentiation and integration, leading us to the desired result.
Method 3: Logarithmic Integration
Logarithmic integration is an enchanting tale of logarithms, those magical numbers that unravel the mysteries of exponential functions. By utilizing the inverse relationship between logarithms and exponentials, we can cast new light on integrals that once seemed impenetrable.
Method 4: Reduction Formula
Introducing reduction formulas, the superheroes of exponential integration! These formulas are like magic wands, reducing complex integrals to simpler ones, paving the way for elegant solutions.
Method 5: Partial Fractions Decomposition
Partial fractions decomposition is a master disguise, breaking down complex rational functions into simpler fractions. Once we’ve unraveled the disguise, integration becomes a breeze, revealing the hidden secrets within.
Embark on Your Integration Odyssey
Now, armed with these powerful techniques, you’re ready to embark on your integration odyssey. Remember, with patience, practice, and a touch of storytelling magic, you’ll conquer even the most daunting exponential integrals.
Additional SEO Optimization Tips:
- Use keyword-rich headings (e.g., “Integrating Exponential Functions: A Step-by-Step Adventure”)
- Include relevant keywords in the article (e.g., “exponential integration,” “substitution method,” “integration by parts”)
- Optimize images with descriptive alt text (e.g., “A graph illustrating the substitution method”)
- Link to authoritative sources for further exploration (e.g., textbooks, online resources)
Mastering the Art of Integrating Exponential Functions: A Comprehensive Guide
Are you grappling with the enigmatic world of exponential functions and their integration? Fret not, for this detailed guide will equip you with the essential techniques to conquer these mathematical challenges.
From the ingenious substitution method to the powerful integration by parts, we’ll delve into each approach with clarity and precision. You’ll learn the secrets behind logarithmic integration, unlocking a new dimension to your mathematical repertoire.
But wait, there’s more! We’ll introduce you to the reduction formula, a valuable tool for simplifying complex exponential integrals. And for those seeking a deeper understanding, we’ll venture into the realm of partial fractions decomposition. By harnessing these techniques, you’ll transform from a mere observer into an integral-conquering master.
Derivation of the Reduction Formula for ∫xe^x dx
Now, let’s tackle the reduction formula, a true game-changer in the world of exponential integrals. We’re going to prove it through a series of clever substitutions.
Starting with ∫xe^x dx, let’s substitute u = xe^x. By the chain rule, du/dx = xe^x + e^x. Rearranging, we get e^x = (du/dx) – xe^x.
Plugging this into our integral, we get ∫xe^x dx = ∫u (du/dx – xe^x) dx = ∫u du – ∫xu e^x dx.
But wait, the integral on the right looks suspiciously like our original one! So, we can rewrite it as ∫xu e^x dx = ∫u (du/dx – xe^x) dx – ∫u du = ∫xe^x dx – ∫u du.
Combining this with the integral on the left, we get 2∫xe^x dx = ∫u du. Dividing both sides by 2, we finally arrive at our reduction formula:
∫xe^x dx = (1/2)∫u du = (1/2)xe^x + C
Armed with this formula, you’ll have a potent weapon in your mathematical arsenal, ready to conquer any exponential integral that comes your way.
Examples of using the reduction formula to solve exponential integrals.
Integrating Exponential Functions: A Comprehensive Guide
Journey with us through the realm of calculus as we delve into the captivating world of integrating exponential functions. We’ll uncover five powerful methods that will empower you to conquer these integrals with ease.
Method 1: Substitution Method
Harness the power of substitution to transform complex exponential integrals into simpler forms. Visualize this method as a secret disguise, where the original function takes on a new identity that simplifies the integration process.
Method 2: Integration by Parts
Think of integration by parts as a dance between two terms: the “u” function and the “dv” function. By skillfully integrating these terms in tandem, you’ll uncover the secrets hidden within exponential integrals.
Method 3: Logarithmic Integration
Unleash the logarithmic trick and you’ll discover a potent weapon against integrals involving e^x. Through a clever derivation, you’ll unlock a formula that empowers you to effortlessly solve these equations.
Method 4: Reduction Formula
Introducing the reduction formula, a magical shortcut that transforms integrals of the form ∫xe^x dx into a simpler version. This technique will shave off precious time and simplify your calculations.
Method 5: Partial Fractions Decomposition
Enter the realm of partial fractions, where we unravel complex rational functions into simpler building blocks. With this skill, you’ll be able to integrate functions involving exponential terms with finesse.
Examples of Using the Reduction Formula to Solve Exponential Integrals
Imagine yourself facing an exponential integral of the form ∫xe^x dx. Fear not! The reduction formula will come to your rescue. By applying this formula, you can effortlessly transform the complex integral into a simpler one: ∫e^x (x-1) dx. This substitution will lead you to the final answer with ease.
Mastering these five integration methods will equip you to conquer any exponential integral that comes your way. Embrace the journey, practice diligently, and you’ll become an integration wizard!
Integrating Exponential Functions: A Comprehensive Guide
In the realm of calculus, exponential functions play a pivotal role, demanding specialized integration techniques. In this blog post, we unravel the secrets of integrating exponential functions, empowering you with a comprehensive toolbox to conquer these mathematical puzzles.
Method 1: Substitution Method
Imagine a function in disguise, hiding its exponential essence within a nested expression. The substitution method comes to the rescue, allowing you to shed that disguise and reveal the true exponential form. By substituting the nested expression with a new variable, you expose the exponential function, making integration a breeze.
Method 2: Integration by Parts
When exponential functions join forces with other functions, integration by parts offers an elegant solution. This technique involves clever factorization, breaking down the integral into manageable chunks. Think of it as a dance between two functions, one as the “u” and the other as “dv,” each contributing to the final solution.
Method 3: Logarithmic Integration
For integrals involving exponential functions, logarithmic integration emerges as a powerful tool. By applying the formula, you transform the integral into a simpler logarithmic form. It’s like unlocking a secret code, revealing the solution hidden beneath the exponential veil.
Method 4: Reduction Formula
Faced with a particularly challenging integral involving an x multiplied by an exponential function? The reduction formula comes to the rescue. It’s a clever trick that simplifies the integral by one step, leading you to the ultimate solution.
Method 5: Partial Fractions Decomposition
When rational functions containing exponential terms dance onto the stage, partial fractions decomposition takes center stage. This technique involves decomposing the function into simpler fractions, each with an exponential denominator. By integrating these fractions separately, you conquer the complex integral with ease.
Integrating Exponential Functions: A Comprehensive Guide
Hello there, intrepid explorers of the mathematical realm! Today, we embark on a captivating journey to conquer the enigmatic world of exponential functions and their integration. Buckle up as we unravel the mysteries of five powerful integration techniques, leaving no stone unturned in our quest for mathematical mastery.
Substitution Method: The Art of Disguise
First up, we encounter the Substitution Method, a master of disguise. By substituting a cleverly chosen variable, we transform our exponential beast into a more manageable form, allowing us to apply our trusty integration techniques. Let’s say we have the integral of e^(x^2) dx. By substituting u = x^2, we can rewrite the integral as (1/2) * e^u du, which we can now integrate with ease.
Integration by Parts: A Dance of Functions
Next, we introduce Integration by Parts, a graceful dance between two functions. We choose two functions, u and dv, and perform a series of manipulations to transform the integral into a more integrable form. This technique works its magic on integrals involving exponential functions paired with trigonometric or exponential functions, such as ∫ e^x sin(x) dx.
Logarithmic Integration: Undoing the Exponential Puzzle
Logarithmic Integration emerges as a savior for integrals involving exponential functions multiplied by polynomials. We introduce a new function, ln(u), where u is an exponential function, and apply the chain rule to undo the exponential function, revealing a simpler integral. This technique shines in integrals like ∫ x^2 * e^(x^3) dx.
Reduction Formula: The Shortcut King
The Reduction Formula emerges as our royal shortcut, particularly for integrals of the form ∫ xe^x dx. By applying integration by parts repeatedly, we derive a formula that drastically simplifies the integration process, reducing the integral to a lower-order exponential integral.
Partial Fractions Decomposition: Splitting the Rational
Finally, we encounter Partial Fractions Decomposition, a master of rational function dissection. We split a rational function into a sum of simpler fractions, each of which can be integrated individually. This technique proves invaluable for integrating rational functions involving exponential functions, such as ∫ (x^2 + 1) / (e^x + 1) dx.
And so, my curious readers, armed with these five techniques, you are now equipped to conquer the challenges of exponential function integration. May your mathematical explorations bear fruitful results!
Mastering the Art of Integrating Exponential Functions: A Comprehensive Guide
In the realm of calculus, exponential functions emerge as a formidable force that demands a refined approach to integration. This blog post will guide you through five powerful integration techniques that will unlock the secrets of exponential functions, empowering you to conquer any integral that comes your way.
1. Substitution Method: A Strategic Approach
Substitution is a cunning technique that transforms an integral into a more manageable form. Here’s how it works:
- Apply the u-substitution by substituting a suitable u = f(x).
- Replace dx with du = f'(x) dx to simplify the integral.
- Solve the integral in terms of u, then substitute back x to find the final answer.
2. Integration by Parts: A Step-by-Step Conquest
Integration by parts resembles a well-choreographed dance between two functions, u and dv. To master this technique, follow these steps:
- Choose u and dv wisely. u should be a function that becomes simpler when differentiated, while dv should be an exponential function.
- Apply the formula: ∫ u dv = uv – ∫ v du.
- Differentiate u and integrate dv to find v and du.
- Plug in these values and simplify to obtain the final result.
3. Logarithmic Integration: A Triumph of Insight
Logarithmic integration unveils the hidden powers of logarithmic functions. Its essence lies in the formula:
∫ e^x dx = e^x + C
To harness this technique, simply:
- Take the natural logarithm of e^x, resulting in ln(e^x) = x.
- Integrate ln(e^x), which yields e^x + C.
- Exponentiate both sides to retrieve the final answer: e^x + C.
4. Reduction Formula: A Recurring Solution
For integrals of the form ∫ xe^x dx, the reduction formula offers a recurring solution:
∫ xe^x dx = x e^x – ∫ e^x dx
This formula can be proven by using integration by parts. By repeatedly applying this formula, you can break down the integral into simpler components, eventually yielding the final result.
5. Partial Fractions Decomposition: A Versatile Tool for Rational Functions
Partial fractions decomposition excels in integrating rational functions. Here’s how it works:
- Decompose the rational function into partial fractions of the form A/(x-a)^n.
- Find the values of A using algebraic techniques.
- Integrate each partial fraction using various integration methods, such as integration by parts or logarithmic integration.
By mastering these five integration techniques, you will become an invincible force in the world of calculus. Conquer any exponential function integral with confidence and uncover the secrets that lie hidden within.