Unveiling The Distribution Coefficient: A Guide To Lipophilicity, Ionization, And Environmental Fate
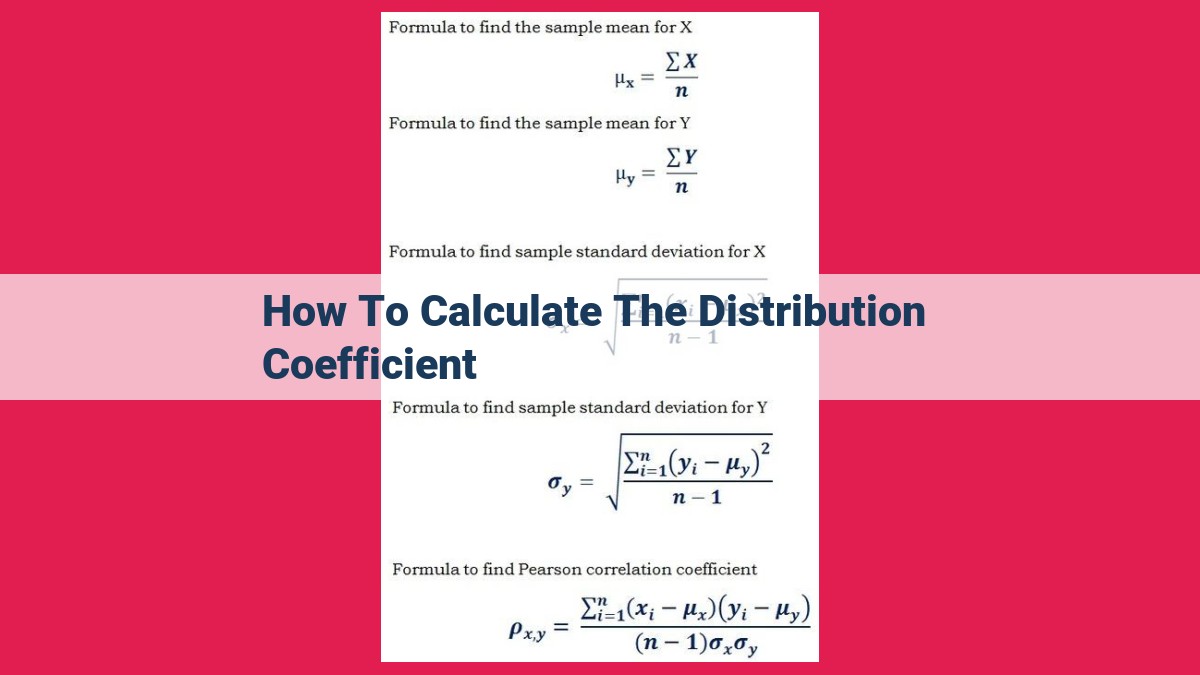
The distribution coefficient, log D, quantifies the ratio of solute concentrations in organic and aqueous phases. It measures lipophilicity (preference for nonpolar environments) and hydrophobicity (resistance to water). Ionization affects distribution due to pH and pKa. The equation for distribution coefficient is [Concentration in Organic Phase] / [Concentration in Aqueous Phase]. Methods for calculating log D include measuring concentrations or using computational models. Factors influencing distribution coefficient include lipophilicity, ionization, pH, temperature, and concentration. Understanding distribution coefficient is crucial for predicting drug behavior, assessing environmental fate, and designing extraction processes.
Unraveling the Distribution Coefficient: A Journey into Solute Distribution
Prepare to embark on a captivating journey into the world of solute distribution. At the helm of our exploration lies the distribution coefficient, a pivotal concept that holds immense significance across diverse fields.
In essence, the distribution coefficient reveals the tendency of a solute to partition between two immiscible phases, such as an organic and an aqueous phase. Measuring the distribution coefficient allows us to gauge the solute’s preference for a particular phase, arming us with crucial insights into its physical and chemical nature.
This versatile concept finds widespread applications in numerous disciplines, including:
- Pharmaceutical Sciences: Predicting the behavior of drugs within the body
- Environmental Chemistry: Understanding the fate of chemicals in the environment
- Extraction Processes: Optimizing the separation and purification of substances
As we delve deeper, we’ll unravel the fascinating concepts related to the distribution coefficient, unraveling the secrets of solute distribution and propelling our understanding of myriad scientific processes.
Concepts
The distribution coefficient, a crucial concept in many scientific fields, quantifies the affinity of a solute for two immiscible phases. It measures the ratio of solute concentrations between an organic (nonpolar) phase and an aqueous (polar) phase. To account for wide-ranging values, the logarithmic distribution coefficient, or log D, is often used.
Related concepts include the partition coefficient and log P. While similar to the distribution coefficient, the partition coefficient specifically refers to the ratio of concentrations in a single-phase system. Log D is a broader term encompassing the distribution coefficient and partition coefficient.
Lipophilicity, the tendency of molecules to dissolve in nonpolar solvents, strongly influences the distribution coefficient. Substances with high lipophilicity are attracted to nonpolar environments, leading to higher concentrations in the organic phase. Lipophilicity is closely linked to hydrophobicity (affinity for nonpolar solvents) and polarity (affinity for polar solvents).
Ionization plays a significant role in altering the distribution coefficient. As pH changes, the ionization state of solutes can vary, affecting their solubility in the two phases. The pH and pKa (acid dissociation constant) are crucial in understanding these ionization effects.
Calculating the Distribution Coefficient: Unveiling the Secrets of Solute Distribution
To determine the distribution coefficient, scientists have devised a mathematical equation that encapsulates the ratio of solute concentrations between two immiscible phases:
Distribution Coefficient (D) = (Concentration in Organic Phase) / (Concentration in Aqueous Phase)
Measuring these concentrations can be achieved through a variety of techniques, including:
- Experimental Methods: Using analytical instruments like chromatography or spectroscopy to quantify solute concentrations.
- Computational Methods: Employing software to predict distribution coefficients based on molecular properties and solvent characteristics.
By harnessing these measurement and computational approaches, scientists can uncover the intricacies of solute distribution, paving the way for a deeper understanding of various phenomena and applications.
Factors Affecting the Distribution Coefficient
The distribution coefficient, a crucial parameter in various fields, is influenced by several key factors that determine the distribution of solutes between organic and aqueous phases. Understanding these factors is essential for accurate predictions and effective applications.
Lipophilicity
Lipophilicity refers to the tendency of molecules to dissolve in nonpolar solvents, such as oils or organic solvents. It is a critical factor in determining the distribution coefficient, as more lipophilic molecules will partition preferentially into the organic phase. This is because lipophilic molecules have a stronger affinity for nonpolar solvents, which have similar molecular structures.
Ionization
Ionization is the process by which a molecule loses or gains electrons, acquiring a net electrical charge. The ionization state of a molecule significantly affects its distribution coefficient. Ionized molecules have a higher solubility in water, as they are attracted to the polar nature of water molecules. Therefore, the distribution coefficient of an ionizable molecule is pH-dependent, with higher ionization favoring the aqueous phase.
pH
The pH of the solution plays a crucial role in the distribution coefficient of ionizable molecules. At a low pH, where the solution is acidic, the molecule is more likely to be protonated and become positively charged. This increases the molecule’s hydrophobicity, making it more likely to partition into the organic phase. Conversely, at a high pH, the molecule becomes deprotonated and negatively charged, favoring the aqueous phase due to its increased polarity.
Temperature
Temperature also affects the distribution coefficient. In general, an increase in temperature results in a decrease in the distribution coefficient. This is because higher temperatures promote the kinetic energy of molecules, making them more likely to overcome the energy barrier between the two phases and distribute more evenly.
Concentration
The concentration of the solute can influence the distribution coefficient, particularly in systems with high solute concentrations. The distribution coefficient may decrease with increasing concentration due to saturation effects or changes in the properties of the solvent. Therefore, it is important to consider the concentration range in which the distribution coefficient is being measured.
Applications of the Distribution Coefficient
Understanding the distribution coefficient is crucial in various fields, and its applications extend to predicting drug behavior, assessing environmental fate, and optimizing extraction processes.
Predicting Drug Behavior
In pharmacokinetics, the distribution coefficient plays a significant role in predicting how drugs will distribute within the body. This is because drugs with higher distribution coefficients tend to accumulate in more lipophilic (fat-soluble) tissues, such as the brain, adipose tissue, and muscle. By knowing the distribution coefficient, researchers can estimate the volume of distribution of a drug, which is essential for determining appropriate dosing and understanding potential drug-drug interactions.
Environmental Fate
The distribution coefficient also provides insights into the environmental fate of chemicals. Chemicals with high distribution coefficients tend to accumulate in non-polar environmental compartments, such as soil and sediments. This information is valuable for assessing the potential for bioaccumulation and understanding the environmental persistence of chemicals. It helps researchers and policymakers make informed decisions about chemical usage and environmental management practices.
Extraction Processes
In separation science and industrial processes, the distribution coefficient is used to optimize extraction processes. By selecting appropriate solvents, engineers can selectively extract target compounds from complex mixtures. This knowledge is vital in industries such as food processing, pharmaceuticals, and environmental remediation. By understanding the distribution of compounds between different phases, scientists can design more efficient and environmentally friendly extraction processes.