Discover Horizontal Asymptotes: A Comprehensive Guide Using Limits

To find the horizontal asymptote of a function using limits, evaluate the limit of the function as x approaches infinity and negative infinity. If the limits exist and are finite, the function has horizontal asymptotes at those values. For example, if lim(x -> ∞) f(x) = 5 and lim(x -> -∞) f(x) = 2, then the function has horizontal asymptotes at y = 5 and y = 2. This method helps determine the end behavior and long-term behavior of functions, providing insights into their overall shape and characteristics.

Understanding Horizontal Asymptotes

Embark on an intriguing journey into the realm of mathematics, where we unravel the secrets of horizontal asymptotes. These elusive lines dance at the edges of functions, revealing crucial insights into their behavior.

A horizontal asymptote is a magical line that a function approaches ever so closely as its input values become infinitely large or infinitely small. It represents a limit that the function can never quite reach but forever chases after.

End behavior, the way a function behaves as its input values wander towards infinity, holds immense significance. Horizontal asymptotes serve as guiding lights, illuminating the path the function takes as it ventures into the far reaches of the numerical landscape.

Limit Definition of Horizontal Asymptotes: Understanding the Key Formula

When examining the behavior of a function at infinity, horizontal asymptotes provide crucial insights into its long-term trends. Mathematically, a horizontal asymptote represents a line that the function approaches as the input (x) tends to either positive or negative infinity. Understanding the limit definition of horizontal asymptotes is essential for accurately identifying and interpreting these significant lines.

The limit definition states that a horizontal asymptote y = L exists if and only if the following limits hold:

• lim(x -> ∞) f(x) = L
• lim(x -> -∞) f(x) = L

In essence, these limits establish that as x approaches positive or negative infinity, the values of f(x) get arbitrarily close to the value L. Imagine a function whose graph extends upwards infinitely on one side and downwards infinitely on the other. The horizontal asymptote would then be a line at a fixed height representing the value that the function approaches as it moves further and further away in both directions.

The existence of these limits indicates that the function has a horizontal asymptote at y = L. Conversely, if either limit does not exist or is infinite, then the function does not have a horizontal asymptote in that direction.

By mastering the limit definition of horizontal asymptotes, we gain a powerful tool for analyzing functions and predicting their behavior at infinity. This understanding forms the foundation for exploring other types of asymptotes and unlocking the full potential of mathematical analysis.

Finding Horizontal Asymptotes Using Limits

• Step-by-step procedure
  • Evaluating the limit at infinity (lim(x -> ∞) f(x))
  • Evaluating the limit at negative infinity (lim(x -> -∞) f(x))

Finding Horizontal Asymptotes Using Limits

Asymptotes are lines that functions approach but never quite reach. Horizontal asymptotes are lines parallel to the x-axis that a function approaches as the input (x) becomes very large (either positive or negative infinity).

To find horizontal asymptotes, we use the limit definition. The limit of a function as x approaches infinity is the value that the function gets arbitrarily close to as x gets very large. If the limit exists and is finite, then the function has a horizontal asymptote at that value.

Step-by-Step Procedure:

1. Evaluate the limit at infinity: Find the limit of the function as x approaches infinity (lim(x -> ∞) f(x)).
2. Evaluate the limit at negative infinity: Find the limit of the function as x approaches negative infinity (lim(x -> -∞) f(x)).

If both limits exist and are equal, then the function has a horizontal asymptote at that value.

Example:

Consider the function f(x) = (x + 2) / (x – 1).

• Limit at infinity: lim(x -> ∞) f(x) = lim(x -> ∞) ((x + 2) / (x – 1))
  = lim(x -> ∞) ((x / x) + (2 / x)) / ((x / x) – (1 / x))
  = lim(x -> ∞) (1 + 2/x) / (1 – 1/x)
  = 1

• Limit at negative infinity: lim(x -> -∞) f(x) = lim(x -> -∞) ((x + 2) / (x – 1))
  = lim(x -> -∞) ((x / x) + (2 / x)) / ((x / x) – (1 / x))
  = lim(x -> -∞) (1 + 2/x) / (1 – 1/x)
  = 1

Since both limits exist and are equal to 1, the function f(x) has a horizontal asymptote at y = 1.

Horizontal Asymptotes: Understanding and Finding Them

Discovering Horizontal Asymptotes

Horizontal asymptotes are imaginary lines that a function approaches as the input value (x) gets very large or very small. They can be visualized as horizontal boundaries that the function’s graph cannot cross.

Limit Definition of Horizontal Asymptotes

Mathematically, a horizontal asymptote y = L exists for a function f(x) if the limit of f(x) as x approaches infinity or negative infinity exists and is equal to L.

Finding Horizontal Asymptotes

To find horizontal asymptotes, you can evaluate the limit of the function as x approaches infinity and negative infinity:

• Limit at Infinity (lim(x -> ∞) f(x)): If this limit exists and is finite, the function has a horizontal asymptote at y = L.
• Limit at Negative Infinity (lim(x -> -∞) f(x)): If this limit exists and is finite, the function has a horizontal asymptote at y = L.

Examples and Applications

Example 1: Consider the function f(x) = (2x + 1)/(x-1).

• lim(x -> ∞) f(x) = 2, so there’s a horizontal asymptote at y = 2.
• lim(x -> -∞) f(x) does not exist, so there’s no horizontal asymptote as x approaches negative infinity.

Example 2: Consider the function g(x) = e^(-x^2).

• Both lim(x -> ∞) g(x) and lim(x -> -∞) g(x) approach 0, so there’s a horizontal asymptote at y = 0.

Practical Applications

Horizontal asymptotes are used in various fields:

• Population Modeling: They can model the limiting value of a population’s growth or decline.
• Chemistry: They can represent the constant concentration of a substance in a reaction as time progresses.
• Economics: They can predict long-term trends in economic indicators like inflation or interest rates.

Other Asymptotes in Functions

• Brief introduction to vertical and oblique asymptotes
• Differences and similarities with horizontal asymptotes

Other Asymptotes in Functions: A Brief Overview

While horizontal asymptotes provide insights into the end behavior of functions, they are not the only types of asymptotes that can occur. Two other notable asymptotes are vertical and oblique asymptotes.

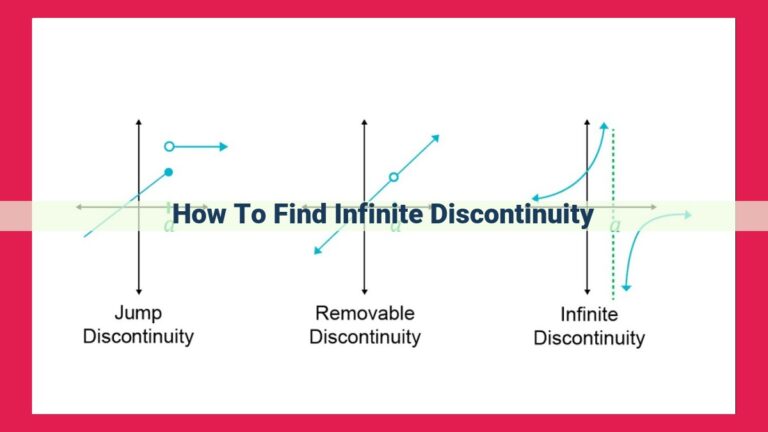
Vertical Asymptotes:

• Vertical asymptotes are vertical lines that the function approaches but never actually touches.
• They occur when the denominator of a rational function becomes zero, making the function undefined at that point.
• Unlike horizontal asymptotes, vertical asymptotes do not indicate a specific y-value that the function approaches.

Oblique Asymptotes:

• Oblique asymptotes are slanted lines that the function approaches as the independent variable becomes very large.
• They occur when the function can be rewritten as a quotient of two polynomial functions with the same degree.
• Unlike horizontal and vertical asymptotes, oblique asymptotes intersect the function at one or more points.

Differences and Similarities with Horizontal Asymptotes:

Characteristic	Horizontal Asymptote	Vertical Asymptote	Oblique Asymptote
Direction	Horizontal	Vertical	Slanted
Behavior	The function approaches the line but never touches it	The function approaches but does not touch the line	The function approaches and intersects the line
Equation	y = L	x = a	y = mx + b
Examples	f(x) = 1/(x-1)	f(x) = 1/x	f(x) = x/(x-1)

In summary, horizontal, vertical, and oblique asymptotes are all important concepts in understanding the behavior of functions. They provide insights into the limits of functions as the input approaches specific values or becomes very large. By analyzing these asymptotes, we can gain a deeper understanding of the overall shape and behavior of a function.