Diffusion In Biological Systems: Understanding Fick’s First Law

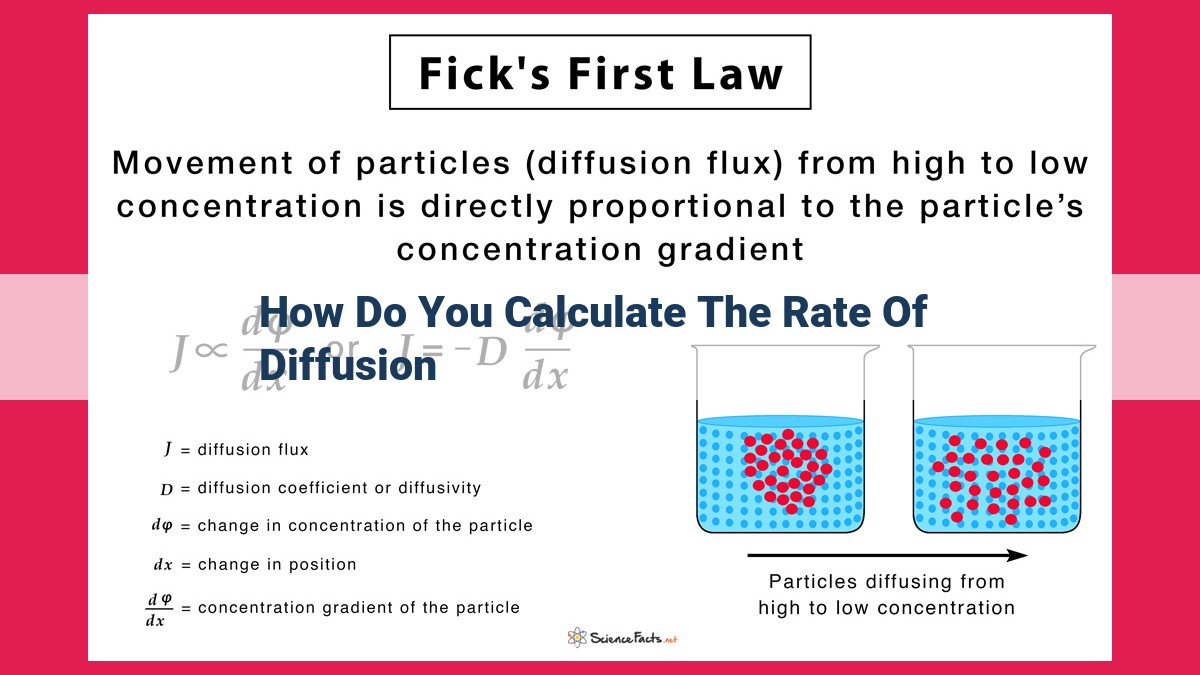
Diffusion is a crucial process in biological systems. Fick’s first law, J = -D * (dC/dx), quantifies diffusion, where J is the flux (rate), D is the diffusion coefficient (influenced by factors like temperature and molecular size), and dC/dx is the concentration gradient. To calculate the diffusion rate, J = -D * [(C2 – C1) / (x2 – x1)], substitute the concentration difference (C2 – C1) and the distance (x2 – x1) over which diffusion occurs. This equation has applications in gas exchange, nutrient transport, drug delivery, and more.

Diffusion: The Invisible Force that Connects the Living World

Every living organism, from the smallest bacteria to the grandest whale, relies on the constant movement of molecules to survive. This movement, known as diffusion, is the invisible force that delivers oxygen to our lungs, nutrients to our cells, and waste products out of our bodies.

Diffusion is a fundamental process in biology, allowing substances to move passively from areas of high concentration to areas of low concentration. It is driven by the random motion of molecules and does not require energy input.

Fick’s first law of diffusion, which we will explore further later, provides a mathematical framework to quantify this movement. It states that the flux of a substance (the rate at which it moves) is proportional to the concentration gradient, or the difference in concentration between two points.

In biological systems, diffusion plays a crucial role in:

• Gas exchange: Oxygen from the air diffuses into our lungs, while carbon dioxide diffuses out.
• Nutrient transport: Nutrients absorbed in the small intestine diffuse into the bloodstream.
• Hormone signaling: Hormones released from glands diffuse to target cells in the body.
• Waste removal: Carbon dioxide and other waste products diffuse out of cells and into the bloodstream to be removed.

Understanding diffusion is essential for comprehending the functioning of the living world and for developing technologies that mimic biological processes, such as drug delivery systems and artificial tissues. By harnessing the power of diffusion, we can improve our health and advance scientific research.

Introduce Fick’s first law of diffusion as the fundamental equation for quantifying diffusion.

Diffusion: The Vital Dance of Molecules in Biological Processes

Diffusion, a pivotal phenomenon in biology, is the unstoppable journey of molecules from areas of high concentration to areas of low concentration, driven by their innate desire to achieve equilibrium. Thanks to this ceaseless dance, biological processes such as gas exchange in lungs and nutrient transport across cell membranes thrive.

At the heart of quantifying diffusion lies Fick’s First Law, an equation that turns the whims of molecular motion into predictable patterns. This law states that the rate of diffusion, or flux (J), is proportional to both the diffusion coefficient (D) and the concentration gradient (dC/dx): J = -D * (dC/dx).

The diffusion coefficient (D) is a measure of a molecule’s mobility in a particular medium. It’s influenced by factors like temperature, viscosity, and the size and shape of the molecule. A smaller molecule, for instance, diffuses faster than a larger one.

The concentration gradient (dC/dx) reflects the difference in concentration between two points. The steeper the gradient, the faster the diffusion. It’s akin to a slope guiding molecules downhill, where each molecule aims to fill the concentration valley.

Diffusion’s significance extends far beyond theoretical equations. It’s a crucial factor in drug delivery systems, ensuring medications reach their targets. It’s also essential for gas exchange in lungs, allowing oxygen to enter and carbon dioxide to exit our bodies.

So, as the molecular orchestra plays its vital role in biological processes, diffusion stands as the conductor, orchestrating the movement and exchange of molecules that sustain life.

Diffusion: The Key to Life’s Movements

Imagine you’re at a crowded party, trying to make your way to the punch bowl. You’ll find it easier to navigate if there are fewer people blocking your path. Similarly, molecules in our bodies move from areas of high concentration to low concentration, guided by the principle of diffusion.

Meet Fick’s First Law: The Diffusion Equation

The mathematical equation that describes diffusion is known as Fick’s first law. It’s like a roadmap for molecules, telling them how much and how quickly they can spread out. The law states that the flux (J), or rate of diffusion, is proportional to the diffusion coefficient (D) and the concentration gradient (dC/dx).

Unpacking the Diffusion Equation

• Diffusion coefficient (D): This value represents how easily a molecule can move through its surroundings. Think of it as the molecule’s “dance skill.”

• Concentration gradient (dC/dx): This term indicates the difference in concentration between two points. It’s like the difference in the number of people between the punch bowl and your current location at the party. A steeper gradient means faster diffusion.

Factors Influencing Diffusion

Several factors can influence the diffusion coefficient and concentration gradient, affecting the rate of diffusion:

Diffusion Coefficient

• Temperature: Higher temperatures give molecules more energy to “dance” around.
• Medium viscosity: Thicker fluids (like honey) slow down diffusion compared to thinner fluids (like water).
• Molecular size and shape: Smaller and more spherical molecules diffuse more quickly.

Concentration Gradient

• Concentration difference: A larger difference in concentration creates a steeper gradient, leading to faster diffusion.
• Distance: The greater the distance between high and low concentration areas, the slower the diffusion.

Putting It All Together

Combining these factors, we can calculate the diffusion rate using Fick’s first law: J = -D * [(C2 – C1) / (x2 – x1)].

• C2 and C1: Concentrations at points x2 and x1
• x2 and x1: Distances from the respective points

Applications in the Real World

Diffusion plays a crucial role in various biological and technological processes:

• Gas exchange in lungs: Oxygen diffuses from the lungs into the blood.
• Nutrient transport: Nutrients diffuse across cell membranes.
• Drug delivery systems: Drugs diffuse through the body to target specific areas.

Understanding diffusion helps us design efficient drug delivery systems, optimize cell function, and even control chemical reactions.

Diffusion is the foundation of many vital processes in our bodies and the world around us. From the movement of nutrients to the delivery of medicines, diffusion is the driving force that keeps things flowing. By understanding the principles of diffusion, we gain a deeper appreciation for the intricate workings of life.

Understanding the Essence of Diffusion: A Journey into Fick’s First Law

Embarking on the Diffusion Enigma

Diffusion, a phenomenon ubiquitous in biology, governs the movement of molecules from areas of high concentration to regions of low concentration. It plays a pivotal role in innumerable biological processes, from oxygen exchange in lungs to nutrient transport across cell membranes. To unravel the intricacies of diffusion, we turn to Fick’s first law, the cornerstone for quantifying this fundamental process.

Deciphering Fick’s First Law: A Blueprint for Diffusion

Fick’s first law, expressed as J = -D * (dC/dx), elucidates the relationship between flux (J), diffusion coefficient (D), and concentration gradient (dC/dx). Flux, the rate of diffusion, depicts the amount of substance traversing a unit area over time. The diffusion coefficient, a substance-specific constant, reflects its inherent ability to diffuse. Finally, the concentration gradient, the crux of diffusion, gauges the change in concentration over a distance.

Dissecting the Diffusion Coefficient: Unveiling the Determinants

The diffusion coefficient, a key player in diffusion, is influenced by temperature, medium viscosity, molecular size, and molecular shape. Higher temperatures enhance molecular mobility, leading to a greater diffusion coefficient. Fluids with lower viscosity facilitate diffusion, as molecules encounter less resistance. Smaller molecules diffuse faster than larger ones, owing to their reduced size. Additionally, elongated or irregular molecules encounter more drag, hindering diffusion.

Concentration Gradient: The Guiding Force of Diffusion

The concentration gradient, the driving force of diffusion, manifests as a disparity in concentration between two points. Substances flow from regions of higher concentration to regions of lower concentration, attempting to equilibrate the system. Factors shaping the concentration gradient include the concentration of the diffusing substance and the distance between points.

Integration of Concepts: Unveiling the Diffusion Rate Equation

By harmonizing the aforementioned concepts, Fick’s first law can be transformed into a comprehensive equation for calculating diffusion rate: J = -D * [(C2 – C1) / (x2 – x1)]. Here, C2 and C1 represent the concentrations at two points, while x2 and x1 denote the corresponding distances.

Diffusion: The Invisible Force Driving Life’s Processes

Diffusion, the silent but crucial process of molecular movement, plays a vital role in life’s symphony, ensuring the seamless flow of substances within and between cells. It’s the invisible force behind the exchange of oxygen in our lungs, the transportation of nutrients through cell membranes, and the delivery of drugs to their targets.

Fick’s First Law: Quantifying Diffusion

At the heart of understanding diffusion lies Fick’s first law, a mathematical equation that quantifies its rate. This law, J = -D * (dC/dx), provides a window into the world of molecular movement. Here, J represents the flux, the rate at which molecules cross a surface; D is the diffusion coefficient, a measure of how easily molecules can move through a given medium; and dC/dx is the concentration gradient, the change in concentration over distance.

The concentration gradient is crucial, as it drives the movement of molecules. Diffusion occurs from areas of high concentration to areas of low concentration, with molecules moving down their concentration gradient to balance out the differences. Imagine a drop of food coloring in a glass of water. The high concentration of food coloring at the drop’s center creates a concentration gradient that causes the molecules to spread throughout the water, eventually creating a uniform color.

Influencing Factors: Diffusion Coefficient

The diffusion coefficient, represented by D, is a dynamic value influenced by several factors. Temperature plays a significant role, with higher temperatures generally increasing the diffusion coefficient as molecules have more energy to overcome resistance. Medium viscosity also comes into play, as molecules diffuse more slowly through thicker, more viscous environments. Molecular size and shape are also crucial, with smaller and more streamlined molecules diffusing more quickly.

Applications: Diffusion in the Real World

Understanding diffusion has practical applications across various fields. In medicine, it aids in calculating drug delivery rates, ensuring optimal drug delivery to affected areas. In engineering, diffusion principles guide the design of membranes and filters for water purification and gas separation. And in environmental science, diffusion equations help predict the spread of pollutants in air and water, enabling the modeling of environmental impacts.

Diffusion, the invisible force that shapes life’s processes, is a testament to the intricate workings of nature. Understanding its principles empowers us to harness this invisible force, opening doors to advancements in medicine, engineering, and other fields. By embracing the power of diffusion, we can unlock the potential of life’s molecular dance and shape a future where its invisible influence is a force for progress.

Diffusion: The Silent Orchestrator of Life’s Symphony

Diffusion, the gentle movement of particles from an area of higher concentration to an area of lower concentration, plays a pivotal role in the intricate web of life. It’s the driving force behind everything from gas exchange in our lungs to the transport of nutrients into our cells. To understand this fundamental process, we turn to Fick’s first law of diffusion, the cornerstone of quantifying diffusion rates.

Fick’s First Law: The Mathematical Expression of Diffusion

Fick’s first law of diffusion – J = -D * (dC/dx) – is an elegant equation that captures the essence of diffusion. It states that the flux of a substance, or the rate at which it moves, is directly proportional to its diffusion coefficient and the concentration gradient.

The diffusion coefficient, D, is a measure of how easily a substance can move through a medium. Factors like temperature, viscosity, molecular size, and shape all influence its value. The higher the diffusion coefficient, the faster the substance will move.

The concentration gradient, dC/dx, represents the change in concentration over distance. It drives diffusion by creating an imbalance in the distribution of particles, causing them to move towards areas of lower concentration.

Putting It All Together: Solving the Puzzle of Diffusion

Substituting D and dC/dx into Fick’s law gives us the equation for calculating diffusion rate: J = -D * [(C2 – C1) / (x2 – x1)]. This formula allows us to quantify the rate of diffusion across a specific distance and concentration gradient.

For instance, in the delicate dance of gas exchange in our lungs, oxygen diffuses from the air into our blood because the concentration of oxygen in the lungs is higher than in the blood. Similarly, carbon dioxide diffuses from our blood into the lungs for the same reason.

Diffusion in Action: From Lungs to Cells

Diffusion’s reach extends far beyond gas exchange. Nutrients such as glucose and amino acids rely on diffusion to enter our cells, where they fuel vital processes. Even in the realm of medicine, diffusion plays a crucial role in drug delivery systems, ensuring that medications reach their target sites.

Understanding diffusion is fundamental to comprehending the intricacies of biological systems and technological innovations. It is the silent orchestrator behind life’s essential processes, from the exchange of gases in our lungs to the transport of molecules within our cells. By unraveling the mysteries of diffusion, we gain insights into the delicate balance that governs the symphony of life.

Fick’s Law of Diffusion: Unveiling the Secrets of Diffusion in Biological Processes

Diffusion is the cornerstone of countless biological processes, allowing substances to move passively down a concentration gradient. Think of it as the microscopic dance of molecules, shuffling from areas of high concentration to those of low concentration, like tiny chemical travelers on an uncharted path through the biological labyrinth.

At the heart of this molecular ballet lies Fick’s first law of diffusion, a fundamental equation that quantifies the rate at which substances diffuse. It’s a mathematical equation that paints a picture of this microscopic waltz, unraveling the secrets of how molecules traverse biological barriers.

Concentration Gradient: The Guiding Force of Diffusion

The concentration gradient, represented as dC/dx, is the driving force behind diffusion. It’s the difference in the concentration of a substance between two points, like the contrast between light and shadow. A steep gradient, with a large difference in concentration, creates a stronger driving force, propelling molecules along the diffusion pathway like eager explorers. Conversely, a shallow gradient, with only a subtle difference in concentration, creates a weaker driving force, causing molecules to meander more leisurely.

Imagine a crowded dance floor, with molecules jostling for space. A steep gradient is like pumping up the music, urging molecules to weave through the crowd with fervor. A shallow gradient, on the other hand, is like playing soft background music, allowing molecules to amble along at their own pace.

Factors that influence the concentration gradient include the concentration of the diffusing substance itself. A higher concentration at the starting point creates a steeper gradient, while a lower concentration leads to a shallower one. Additionally, the distance between points plays a role: a shorter distance results in a steeper gradient, while a longer distance yields a shallower one.

Understanding the concentration gradient is critical for deciphering the dynamics of diffusion. Think of it as the map guiding molecules on their diffusive journey, propelling them towards lower concentrations and shaping the biological landscape.

Diffusion Coefficient: The Lifeline of Molecular Movement

Diffusion, the silent but pivotal force behind the seamless exchange of molecules in our bodies and beyond, has a secret weapon: the diffusion coefficient. This magical number quantifies the inherent ability of a substance to meander through its surroundings. It’s the metric that determines how quickly molecules spread out, whether it’s oxygen infiltrating our lungs, nutrients nourishing our cells, or drugs navigating our bodies.

The diffusion coefficient, symbolized by the letter D, reflects the unique characteristics of the diffusing molecule and its environment. D depends on temperature, the catalyst for molecular movement; viscosity, the medium’s resistance to flow; and molecular size and shape, with smaller, more streamlined molecules dancing through space with greater ease.

Picture D as a conductor in an orchestra of molecules. A high D means molecules waltz elegantly across the stage, while a low D forces them to stumble and weave through the crowd. It’s a crucial factor in determining whether molecules reach their intended destinations swiftly or dawdle along the way, affecting everything from cell function to drug delivery efficiency.

Discuss the key factors influencing the diffusion coefficient, such as temperature, medium viscosity, molecular size, and shape.

Diffusion: The Silent Mover of Biological Processes

Diffusion, the passive movement of particles from an area of high concentration to an area of low concentration, plays a crucial role in biological processes. Imagine oxygen moving from your lungs to your bloodstream or nutrients being absorbed from your intestines. These critical transfers happen through the silent power of diffusion.

Fick’s first law of diffusion, a fundamental equation in physics, quantifies this process. It states that the flux of diffusion (J), or the rate at which particles move, is directly proportional to the concentration gradient (dC/dx) and inversely proportional to the diffusion coefficient (D).

The Fickle Diffusion Coefficient

The diffusion coefficient, a substance-specific constant, determines how quickly a molecule moves through a medium. Several factors influence D, including:

• Temperature: Heat increases molecular motion, leading to higher diffusion coefficients.
• Medium viscosity: Thicker media, like honey, hinder molecular movement, lowering diffusion coefficients.
• Molecular size and shape: Smaller, spherical molecules diffuse faster than larger, irregularly shaped ones.

The Guiding Force: Concentration Gradient

The concentration gradient, the difference in concentration between two points, drives diffusion. A steeper gradient leads to a faster rate of diffusion. The distance between the two points also matters; a shorter distance facilitates a higher diffusion rate.

Factors That Modify Diffusion

Besides D and dC/dx, other factors influence diffusion rates:

• Surface area: A larger surface area allows for more particles to diffuse, increasing the rate.
• Distance: The shorter the distance, the faster the diffusion.
• Time: Diffusion is a time-dependent process; with more time, more particles will diffuse.

Calculating Diffusion Rates: The Fick’s Math

By combining the concepts above, we can derive Fick’s law equation: J = -D * [(C2 – C1) / (x2 – x1)].

Here, C2 and C1 represent the concentrations at two points, and x2 and x1 represent the corresponding distances. A negative sign indicates that diffusion occurs from an area of higher to lower concentration.

Real-World Applications: Diffusion in Action

Diffusion is essential in numerous biological processes, including:

• Gas exchange in lungs: Oxygen moves from the lungs into the bloodstream.
• Nutrient absorption: Nutrients pass from the digestive tract into cells.
• Drug delivery systems: Drugs are designed to diffuse into targeted areas.

Understanding diffusion is vital in biology, chemistry, and technology. By mastering the concepts of Fick’s law, we can better comprehend the silent yet fundamental role diffusion plays in the world around us.

Diffusion: Understanding the Flow of Molecules in Biology

The intricate world of biology is governed by a fundamental process known as diffusion, where molecules move effortlessly from areas of high concentration to areas of low concentration. This phenomenon is essential for a vast array of biological processes, ranging from the exchange of gases in our lungs to the transport of nutrients into our cells. To unravel the secrets of diffusion, we turn to Fick’s first law of diffusion, a cornerstone of understanding this fascinating phenomenon.

At the heart of Fick’s law lies the concentration gradient, a crucial factor that drives the movement of molecules. Imagine a swarm of tiny particles suspended in a fluid, eager to escape from crowded regions to less densely populated spaces. The concentration gradient acts as a guiding force, directing these particles along a path of least resistance.

The greater the difference in concentration, the steeper the concentration gradient and the faster the diffusion rate. Molecules sense this gradient and respond accordingly, moving downhill from high concentrations to low concentrations. This relentless pursuit of equilibrium ensures that molecules are evenly distributed, creating a state of dynamic balance.

Just as the gradient of a mountain path determines the ease of descent, the concentration gradient influences the rate of particle movement. A steep gradient encourages rapid diffusion, while a gentle gradient leads to a more leisurely pace. This process is the driving force behind numerous biological processes, such as the absorption of oxygen from the lungs into the bloodstream and the removal of waste products from cells.

Understanding the concept of concentration gradient is paramount to comprehending the dynamics of diffusion. It is the driving force that orchestrates the movement of molecules, shaping the molecular landscape within our bodies and enabling the vital processes that sustain life.

Diffusion: A Journey from Concentration to Equilibrium

Diffusion plays a crucial role in the symphony of life. It’s the driving force behind the exchange of nutrients, gases, and molecules within our bodies and the environment. To unravel the secrets of this molecular ballet, we turn to Fick’s first law of diffusion, the fundamental equation that quantifies this enigmatic process.

At the heart of Fick’s law lies the concept of the concentration gradient, a measure of how unevenly a substance is distributed in space. It’s a tale of concentration highs and lows, where molecules yearn to journey from areas of high concentration to regions of low concentration.

Concentration, the Guiding Light

The concentration of the diffusing substance plays a pivotal role in shaping the concentration gradient. Imagine a crowded party where everyone eagerly attempts to find their way to a quieter corner. The more people there are, the steeper the concentration gradient and the more intense the diffusion.

Distance: The Obstacle Course

The distance between points also influences the concentration gradient. Think of a long, winding road that separates a bustling city from a tranquil countryside. The farther apart these points are, the shallower the gradient and the slower the diffusion.

Diffusion’s Orchestra: Additional Players

Surface area, distance, and time join forces with the concentration gradient to orchestrate the dance of diffusion.

• Surface area: The larger the surface area, the more opportunities there are for diffusion to occur. Think of the difference between a large sponge and a small pebble.
• Distance: The shorter the distance, the less resistance molecules encounter on their journey.
• Time: Diffusion is a patient process that unfolds gradually over time. The longer the time, the more molecules have the opportunity to relocate.

Calculating the Diffusion Rate: A Matter of Balance

Fick’s law weaves together these concepts into a harmonious equation:

**J = -D * [(C2 - C1) / (x2 - x1)]**

where:

• J represents the flux, the rate of diffusion.
• D is the diffusion coefficient, a constant that reflects the ease with which a substance diffuses.
• C2 – C1 is the concentration difference between the two points.
• x2 – x1 is the distance between the two points.

This equation reveals the delicate balance between concentration gradients and diffusion coefficients. The steeper the gradient, the higher the flux, driving molecules towards equilibrium. Conversely, a high diffusion coefficient facilitates faster movement, leading to a more rapid balancing act.

Applications: Diffusion’s Practical Magic

Diffusion’s dance plays a crucial role in a myriad of biological and technological realms:

• Gas exchange in lungs: Oxygen from inhaled air diffuses into the bloodstream, while carbon dioxide takes the opposite journey.
• Transport of nutrients: Molecules such as glucose and amino acids diffuse across cell membranes, fueling cellular activities.
• Drug delivery systems: Controlled diffusion ensures that medications are released gradually into the body, maximizing their therapeutic effects.

Diffusion is the maestro that orchestrates the movement of molecules, shaping the intricate tapestry of life. By understanding the factors that influence this process, we unravel the secrets of biological systems and unlock the potential for technological advancements.

The Influence of Surface Area on Diffusion Rates: A Journey Through the Biological Realm

In the intricate world of biology, diffusion plays a pivotal role in countless essential processes, from the exchange of gases in the lungs to the transport of nutrients within cells. Understanding the dynamics of diffusion is crucial for comprehending these phenomena and their significance in our daily lives.

One of the key factors influencing diffusion rates is surface area. Imagine a crowded street during rush hour. The more lanes you have, the faster the traffic can flow. Similarly, in the context of diffusion, a larger surface area provides more pathways for molecules to move from areas of high concentration to low concentration.

Here’s a real-life example: Your body’s lungs are designed with an enormous surface area. This allows for efficient gas exchange between the lungs and the bloodstream, ensuring a steady supply of oxygen to your cells and the removal of waste products like carbon dioxide.

The relationship between surface area and diffusion rate is captured by Fick’s First Law of Diffusion, which states that the rate of diffusion is directly proportional to the surface area through which the molecules can move. This principle underscores the importance of maximizing surface area in applications such as drug delivery systems and biomedical devices.

By understanding the impact of surface area on diffusion, we gain insights into the intricate workings of our bodies and the potential for harnessing diffusion principles in technological advancements that enhance our health and well-being.

Distance: The Spatial Dimension of Diffusion

In the realm of diffusion, distance plays a pivotal role, dictating the rate at which molecules traverse space. Visualize a crowded party where people mingle and move about. Those closer to the doorway can enter and leave more quickly than those stuck in the throng further away.

Similarly, in diffusion, the distance between the source of a substance and the point of interest influences the rate of diffusion. The greater the distance, the longer it takes for molecules to reach their destination. This is because molecules must overcome obstacles and navigate mazes of other molecules as they travel.

Consider a plant’s root system absorbing water from the soil. The roots are in direct contact with the water, so water molecules can easily diffuse into them. However, as water moves up the stem, it faces a greater distance from the source. This increased distance slows down the rate of diffusion, ensuring a steady but sustained flow of water throughout the plant.

In biological systems, diffusion often occurs over microscopic distances. For instance, oxygen molecules must diffuse across cell membranes to reach the mitochondria, where they are used for energy production. The short distances involved in these processes allow for rapid diffusion, facilitating efficient cellular function.

Conversely, in larger-scale systems, diffusion can be hindered by long distances. Imagine a lake filled with dissolved nutrients. The nutrients will diffuse from the surface into the deeper layers, but the great distance slows down this process. As a result, nutrient concentrations may vary significantly with depth, affecting the distribution of aquatic organisms.

Understanding the influence of distance on diffusion is crucial in various fields. In medicine, it helps optimize drug delivery systems by ensuring that drugs can penetrate deep enough into tissues to reach their target sites effectively. In engineering, it guides the design of filters and membranes by optimizing pore sizes for efficient molecular transport.

By unraveling the intricacies of distance and diffusion, we gain a deeper appreciation for the intricate dance of molecules that shapes life and technology alike.

The Passage of Time in Diffusion: An Intricate Dance of Molecules

Imagine a world where tiny molecules dance and travel, propelled by a relentless force that connects them to their surroundings: diffusion. This seemingly simple process is the driving force behind countless biological wonders, from the exchange of oxygen in our lungs to the delivery of nutrients to our cells.

Time’s Influence

In the world of diffusion, time plays a crucial role, like a conductor orchestrating the molecular ballet. The time it takes for molecules to traverse a distance is directly proportional to the distance they must travel. As the distance between two points grows, so too does the time required for molecules to diffuse across that expanse.

Distance Matters

Think of a crowd of people trying to evacuate a building after a fire alarm. The farther away from the exit, the longer it takes people to reach safety. Similarly, in diffusion, the farther the distance between two points of different concentrations, the more time molecules take to spread out and equalize their concentrations.

Surface Area and Shape

Another factor influencing the rate of diffusion is the surface area through which molecules can move. Just as a wider door allows more people to pass through, a larger surface area enables molecules to diffuse more rapidly. Additionally, the shape of the diffusing molecules also plays a role, with smaller and more streamlined molecules diffusing faster than larger or irregularly shaped ones.

Concentration Gradient: The Driving Force

The concentration gradient is the key driving force behind diffusion. This gradient refers to the difference in the concentration of a substance between two points. The greater the concentration gradient, the faster the molecules will diffuse to equalize the concentrations.

Understanding the role of time, distance, surface area, and concentration gradient is essential for comprehending the intricate dance of diffusion. These factors govern how quickly molecules move, enabling biological processes to function seamlessly. From the exchange of gases in our lungs to the delivery of life-sustaining nutrients, diffusion’s relentless pulse ensures that the symphony of life continues.

Substitute the above concepts into Fick’s law to derive the equation for calculating diffusion rate: J = -D * [(C2 – C1) / (x2 – x1)].

Diffusion: The Silent Symphony of Life

In the intricate tapestry of life, diffusion plays a pivotal role, silently orchestrating the ebb and flow of vital substances. It’s the unseen force that transports oxygen into our lungs, nutrients into our cells, and hormones through our bodies. Understanding diffusion is crucial to unraveling the mysteries of biological processes and engineering technological marvels.

Fick’s Law: The Maestro of Diffusion

Amongst the scientific tools that guide us in quantifying diffusion, Fick’s first law stands out as the maestro. It elegantly articulates that the flux (rate of diffusion) is directly proportional to the diffusion coefficient and the concentration gradient.

J = -D * dC/dx

Here’s a quick breakdown of the key variables:

• J: The flux represents how quickly a substance moves over time and distance.
• D: The diffusion coefficient reflects a substance’s intrinsic ability to move through a particular medium.
• dC/dx: The concentration gradient measures the change in concentration over distance, guiding the movement of substances from areas of higher to lower concentrations.

Diffusion Coefficient: A Fingerprint of Movement

The diffusion coefficient is a unique fingerprint for each substance, influenced by several factors. Temperature plays a crucial role, with higher temperatures enhancing diffusion rates. Viscosity of the medium, like the thickness of a fluid, also affects diffusion; the thicker the medium, the slower the diffusion. Additionally, the molecular size and shape influence diffusion, with smaller and more spherical molecules diffusing more readily.

Concentration Gradient: The Driving Force

The concentration gradient acts as the invisible force driving diffusion. Substances tend to move from areas where they are abundant to where they are scarce, equalizing concentrations and creating a state of equilibrium. The concentration difference and the distance between points determine the steepness of the concentration gradient, which in turn governs the rate of diffusion.

Related Concepts: A Trifecta of Influence

Diffusion is influenced by several related concepts:

• Surface Area: The larger the surface area available for diffusion, the faster the rate of diffusion. Think of a porous membrane allowing substances to pass through more easily.
• Distance: A longer distance between points means a greater concentration gradient, leading to a higher diffusion rate.
• Time: Over time, diffusion tends to equalize concentrations, reducing the concentration gradient and slowing down the diffusion process.

Calculating Diffusion Rates: Unveiling the Unseen

Combining these concepts with Fick’s law allows us to calculate diffusion rates accurately. The equation J = -D * [(C2 – C1) / (x2 – x1)] reveals the interplay between flux (J), diffusion coefficient (D), initial concentration (C1), final concentration (C2), initial position (x1), and final position (x2).

Applications: Diffusion’s Dance in the Real World

Diffusion is a ubiquitous phenomenon with countless applications in the real world:

• Gas Exchange in Lungs: Oxygen and carbon dioxide swiftly diffuse across the delicate lung membranes, sustaining life’s breath.
• Nutrient Transport: Nutrients are transported into cells through cell membranes, fueling cellular processes and enabling growth and repair.
• Drug Delivery Systems: Controlled drug delivery relies on the understanding of diffusion principles, ensuring precise and targeted drug delivery.

Diffusion, the silent symphony of life, is an integral process that underpins numerous biological functions. By delving into Fick’s first law and exploring related concepts, we gain a deeper appreciation for the intricate dance of substances in our bodies and the technological advancements that harness this vital force. Understanding diffusion empowers us to optimize drug delivery, enhance gas exchange, and unravel the mysteries that lie at the heart of life’s biological processes.

The Secret Formula: Unraveling the Significance of Diffusion Coefficients

Imagine a bustling city where molecules mingle like commuters rushing through subway stations. This dynamic dance is powered by diffusion, the key to countless biological processes, from oxygen reaching our cells to nutrients fueling our bodies. Yet, quantifying this molecular motion requires a secret formula: Fick’s First Law of Diffusion.

2. Fick’s First Law: The Equation That Governs Diffusion

Like a roadmap, Fick’s law guides us through the realm of diffusion. It states that the flux, or rate of molecular movement, is equal to the negative of the diffusion coefficient multiplied by the concentration gradient:

J = -D * (dC/dx)

3. The Diffusion Coefficient: A Key Player in Molecular Mobility

The diffusion coefficient, symbolized by D, is a constant that reflects how easily molecules can navigate their surroundings. Temperature plays a crucial role, with higher temperatures leading to faster diffusion. Additionally, the viscosity of the medium (think of it as molecular resistance) and the size and shape of the diffusing molecules influence the ease of their movement.

4. The Concentration Gradient: The Compass Guiding Molecular Movement

The concentration gradient, represented by dC/dx, measures the change in concentration over distance. It acts as a compass, directing molecules from areas of higher concentration to areas of lower concentration. Picture two reservoirs of water, one filled with salt and the other pure. The salt molecules will naturally flow from the higher concentration (salty water) to the lower concentration (pure water), driven by the concentration gradient.

5. Putting It All Together: Calculating Diffusion Rate

Now, let’s substitute the diffusion coefficient and concentration gradient into Fick’s law and solve for J, the diffusion rate:

J = -D * [(C2 – C1) / (x2 – x1)]

This equation shows that the diffusion rate depends on four key factors:

• C2 and C1: The concentrations of the substance at two points
• x2 and x1: The distances between those points

6. Real-World Applications: Diffusion in Action

Diffusion plays a vital role in many practical applications, such as:

• Gas exchange in our lungs: Oxygen diffuses from the air into our bloodstream, while carbon dioxide diffuses out.
• Nutrient transport through cell membranes: Sugars and amino acids diffuse into cells to fuel cellular processes.
• Drug delivery systems: Controlled diffusion ensures the targeted release of medication into the body.

By understanding the significance of the diffusion coefficient, concentration gradient, and other key factors, we gain a deeper appreciation for the intricate dance of molecules in biological systems. Diffusion is not just a fascinating phenomenon; it’s a cornerstone of life, enabling the constant exchange of nutrients, gases, and other vital substances that sustain our very existence.

Diffusion in Biological Processes: Understanding Fick’s Law for Real-World Applications

Diffusion, the movement of particles from an area of high concentration to an area of low concentration, plays a crucial role in numerous biological processes. Fick’s first law of diffusion quantifies this movement, providing insights into how substances move within and between living organisms.

Understanding Fick’s First Law

According to Fick’s law, the flux (rate of diffusion) of a substance is directly proportional to the diffusion coefficient (D) and the concentration gradient (dC/dx). The diffusion coefficient is a constant unique to each diffusing substance, influenced by factors like temperature, medium viscosity, molecular size, and shape. The concentration gradient measures the difference in concentration between two points.

Calculating Diffusion Rates

Using Fick’s law, we can calculate diffusion rates for various applications, including:

• Gas Exchange in Lungs: Carbon dioxide diffuses out of the blood into the lungs, while oxygen diffuses from the lungs into the blood. The diffusion rate depends on the partial pressure gradients of these gases.
• Transport of Nutrients through Cell Membranes: Nutrients pass through cell membranes via diffusion. The rate is determined by the nutrient’s concentration gradient and the diffusion coefficient, which varies depending on the membrane’s composition.
• Drug Delivery Systems: Drug delivery devices rely on diffusion to release drugs into the body. The release rate is controlled by factors such as the drug’s concentration gradient, surface area, and diffusion coefficient.

Influencing Factors on Diffusion

Besides the diffusion coefficient and concentration gradient, other factors also influence diffusion rates:

• Surface Area: The larger the surface area, the greater the diffusion rate.
• Distance: Diffusion rates decrease with increasing distance between the source and destination of the diffusing substance.
• Time: Over time, diffusion gradually equalizes concentrations, reducing the concentration gradient and slowing down the diffusion rate.

Fick’s first law of diffusion provides a powerful tool for understanding and calculating diffusion rates in biological and technological applications. By manipulating factors such as the diffusion coefficient, concentration gradient, surface area, distance, and time, we can design systems that optimize diffusion for desired outcomes, such as efficient gas exchange, nutrient transport, and drug delivery.

Understanding Diffusion: A Guide to Fick’s First Law

Diffusion plays a crucial role in various biological processes, such as gas exchange in the lungs, nutrient transport through cell membranes, and even the delivery of drugs. Gaining a solid understanding of diffusion is essential for comprehending these vital functions.

Fick’s first law of diffusion serves as the cornerstone for quantifying this fundamental process. This law states that the flux (or rate of diffusion) is directly proportional to the concentration gradient and the diffusion coefficient. In other words, diffusion occurs from areas of high concentration to low concentration, and the rate of diffusion depends on the steepness of this concentration gradient and the ease with which molecules can move through the medium.

Let’s delve into the key components of Fick’s first law:

• Flux (J): This represents the rate at which molecules move across a given area. It is measured in moles per second per square meter.

• Diffusion coefficient (D): This measures the ease with which molecules can move through a medium. It is influenced by factors such as temperature, viscosity, molecular size, and shape.

• Concentration gradient (dC/dx): This describes the change in concentration over distance. It indicates the direction and magnitude of diffusion.

In the human respiratory system, gas exchange in the lungs is a prime example of diffusion at work. Oxygen from the inhaled air diffuses across the thin alveolar membranes and into the bloodstream, while carbon dioxide moves in the opposite direction. Fick’s law helps quantify this exchange, which is essential for maintaining proper oxygen levels in the body.

Calculating Diffusion Rates

To calculate diffusion rates, we can substitute the above components into Fick’s first law:

J = -D * [(C2 - C1) / (x2 - x1)]

• J: Diffusion rate
• D: Diffusion coefficient
• C2: Concentration at position 2
• C1: Concentration at position 1
• x2: Position 2
• x1: Position 1

This equation enables researchers and medical professionals to determine diffusion rates in various biological and technological applications, such as drug delivery systems and the design of artificial lungs.

In conclusion, Fick’s first law of diffusion provides a powerful tool for understanding and predicting the movement of molecules across concentration gradients. Its applications span a wide range of fields, from medicine to engineering. By mastering this fundamental concept, we gain a deeper appreciation for the intricate workings of biological systems and the ability to harness diffusion for technological advancements.

Diffusion: Key to Life’s Essential Processes

Diffusion, the movement of molecules from areas of high concentration to low concentration, is a crucial process in biological systems. From nutrient absorption to oxygen exchange, diffusion plays a pivotal role in sustaining life.

Fick’s First Law of Diffusion:

The fundamental equation describing diffusion is Fick’s first law, which quantifies the rate of diffusion (J) as the product of the diffusion coefficient (D) and the concentration gradient (dC/dx). The concentration gradient, in turn, measures the change in concentration over distance.

Diffusion Coefficient:

The diffusion coefficient is a characteristic of the diffusing substance and the surrounding medium, influenced by factors such as temperature, viscosity, and the size and shape of the molecule.

Concentration Gradient:

The concentration gradient arises from differences in substance concentration at different points. It drives diffusion as molecules move down the gradient, from areas of high concentration to areas of low concentration.

Calculation:

Combining Fick’s law and the concepts above, we can derive an equation to calculate J:

J = -D * [(C2 - C1) / (x2 - x1)]

where C2 and C1 are concentrations at points x2 and x1, respectively.

Applications: Transport of Nutrients through Cell Membranes

Cell membranes selectively regulate the movement of nutrients into and out of cells. Diffusion plays a key role in this process, allowing essential nutrients like glucose and amino acids to cross the membrane down their concentration gradients. This transport is critical for cell growth, energy production, and protein synthesis.

Understanding diffusion is essential for understanding many biological and technological processes. By applying Fick’s law, we can calculate diffusion rates and gain insights into the transport of nutrients and other substances through cell membranes and other biological systems.

Diffusion: The Vital Force Driving Life Processes and Drug Delivery

Diffusion, the silent orchestra behind countless biological processes, is a phenomenon of paramount importance in the world of living organisms. The constant movement of molecules from regions of high concentration to low concentration drives a symphony of cellular activities. Understanding the principles of diffusion, as elegantly described by Fick’s first law, empowers us to unravel the intricacies of life and devise innovative drug delivery systems.

Fick’s First Law of Diffusion: The Equation of Motion

Imagine a molecular ballet, where molecules dance along a concentration gradient, transitioning from crowded regions to less dense ones. This orchestrated movement is quantified by Fick’s first law:

J = -D * (dC/dx)

where:

• J represents the flux – the rate at which molecules traverse a given area
• D is the diffusion coefficient – a measure of how easily molecules can move through a medium
• dC/dx is the concentration gradient – the change in concentration over distance

Diffusion Coefficient: The Molecule’s Passport

The diffusion coefficient is akin to a passport that determines a molecule’s mobility. Temperature acts as a traffic controller, increasing the temperature accelerates molecular motion, and vice versa. Likewise, the medium’s viscosity – its resistance to flow – influences diffusion. The size and shape of molecules also play a vital role, with smaller and more compact molecules enjoying greater ease of movement.

Concentration Gradient: The Guiding Light

The concentration gradient serves as a guiding light, directing molecules towards areas of lower concentration. The greater the gradient, the more pronounced the diffusion, like water flowing downhill. Factors such as the concentration of the diffusing substance and the distance between points significantly influence the gradient.

Surface Area, Distance, and Time: The Diffusion Triangle

The surface area through which diffusion occurs is like a wide-open gate, allowing for a more rapid exchange of molecules. The distance between points acts as a hurdle, slowing down diffusion. Time, the ever-ticking clock of life, plays a crucial role, as the longer the diffusion process continues, the more molecules reach their destination.

Calculation: Unlocking the Secrets of Diffusion

By combining these concepts, we can derive the equation for calculating the diffusion rate:

J = -D * [(C2 - C1) / (x2 - x1)]

where C2 and C1 represent the concentrations at points x2 and x1, respectively. This equation provides a powerful tool for quantifying diffusion in various biological and technological applications.

Applications: Diffusion in the Real World

Diffusion finds myriad applications in the realm of drug delivery. By understanding the principles of diffusion, scientists can design drug delivery systems that effectively target specific tissues and cells. Controlled release of drugs can be achieved through carefully engineered diffusion barriers, ensuring optimal therapeutic efficacy.

Diffusion stands as a cornerstone of biological processes, underpinning the exchange of oxygen and nutrients, the transport of molecules across cell membranes, and the delivery of life-saving drugs. By unraveling the secrets of diffusion, we gain invaluable insights into the intricate workings of life and unlock the potential for novel therapeutic interventions.

Summarize the key concepts involved in calculating the rate of diffusion.

Diffusion: The Vital Force in Biological Processes

In the intricate tapestry of life, diffusion plays a pivotal role. It’s the silent dance of molecules, a constant flow that sustains the very essence of existence. From the exchange of gases in our lungs to the delivery of nutrients into our cells, diffusion is an indispensable force driving countless biological processes.

Fick’s Guiding Equation

To quantify this enigmatic phenomenon, scientists have devised Fick’s first law of diffusion. This equation, like a mathematical roadmap, charts the course of diffusion:

J = -D * (dC/dx)

Here, J represents the flux, the rate at which molecules traverse a given area. D is the diffusion coefficient, a measure of how readily a molecule moves through its surroundings. And dC/dx captures the concentration gradient, the difference in concentration between two points.

Factors that Shape Diffusion

The diffusion coefficient is a fickle creature, influenced by an array of factors. Temperature sets the pace of molecular motion, while medium viscosity acts as a sticky barrier. Molecular size and shape also play a crucial role, with smaller, more streamlined molecules gliding effortlessly through the crowd.

The concentration gradient, too, is a dynamic force. It’s shaped by the concentration of the diffusing substance and the distance between points. The steeper the gradient, the more molecules are driven to move from high-concentration zones to low-concentration zones.

Related Concepts: The Diffusion Dance

Like partners in a waltz, diffusion is intertwined with other concepts:

• Surface area: The larger the surface area, the faster the diffusion rate. Imagine a sprawling coastline, where the vast expanse of sand welcomes the influx of seawater.
• Distance: The shorter the distance, the more efficiently molecules can travel. It’s like a bustling metropolis, where a pedestrian can quickly cross a narrow street.
• Time: Diffusion is a gradual process, but time is on its side. Over time, molecules will spread throughout a space, like raindrops gently saturating the earth after a storm.

Calculating the Diffusion Rate: A Formula for Understanding

To truly harness diffusion’s power, we must calculate its rate. By substituting the concepts we’ve explored into Fick’s law, we arrive at the equation:

J = -D * [(C2 – C1) / (x2 – x1)]

Here, C2 and C1 represent the concentrations at two points, while x2 and x1 denote their respective positions. This equation allows us to precisely determine how quickly molecules will diffuse.

Applications in the Real World: Diffusion’s Practical Magic

Diffusion finds countless applications in our world:

• In our lungs, it orchestrates the vital exchange of oxygen and carbon dioxide.
• It transports nutrients across cell membranes, fueling the intricate machinery of our bodies.
• Drug delivery systems rely on diffusion to precisely release medication into targeted areas.

The Essence of Diffusion: Unlocking the Secrets of Biological and Technological Processes

Diffusion, an invisible yet fundamental force, plays a vital role in shaping the world we inhabit. From the exchange of oxygen in our lungs to the delivery of nutrients to our cells, diffusion underpins countless biological processes. It is a silent orchestrator, facilitating the movement of substances across boundaries.

One of the most important laws governing diffusion is Fick’s first law. This equation quantifies the rate of diffusion, or the flux, as the product of a diffusion coefficient and a concentration gradient. The diffusion coefficient measures the ease with which a substance can move through a medium, while the concentration gradient represents the change in concentration over distance.

In biological systems, diffusion is crucial for the transport of substances across cell membranes. Cell membranes are selectively permeable barriers that allow certain molecules to pass through while blocking others. Diffusion enables nutrients, ions, and other essential substances to enter and exit cells, maintaining cellular homeostasis.

Diffusion also finds applications in a variety of technological fields. In medical devices, diffusion is used to deliver drugs to specific targets within the body. In engineering, diffusion is harnessed to design materials with controlled release properties, such as biodegradable implants.

Understanding diffusion is essential for comprehending the workings of living organisms and designing effective technologies. By unraveling the intricacies of diffusion, we gain insights into the hidden forces that shape our world.