How To Determine Reaction Rate Constants: A Comprehensive Guide

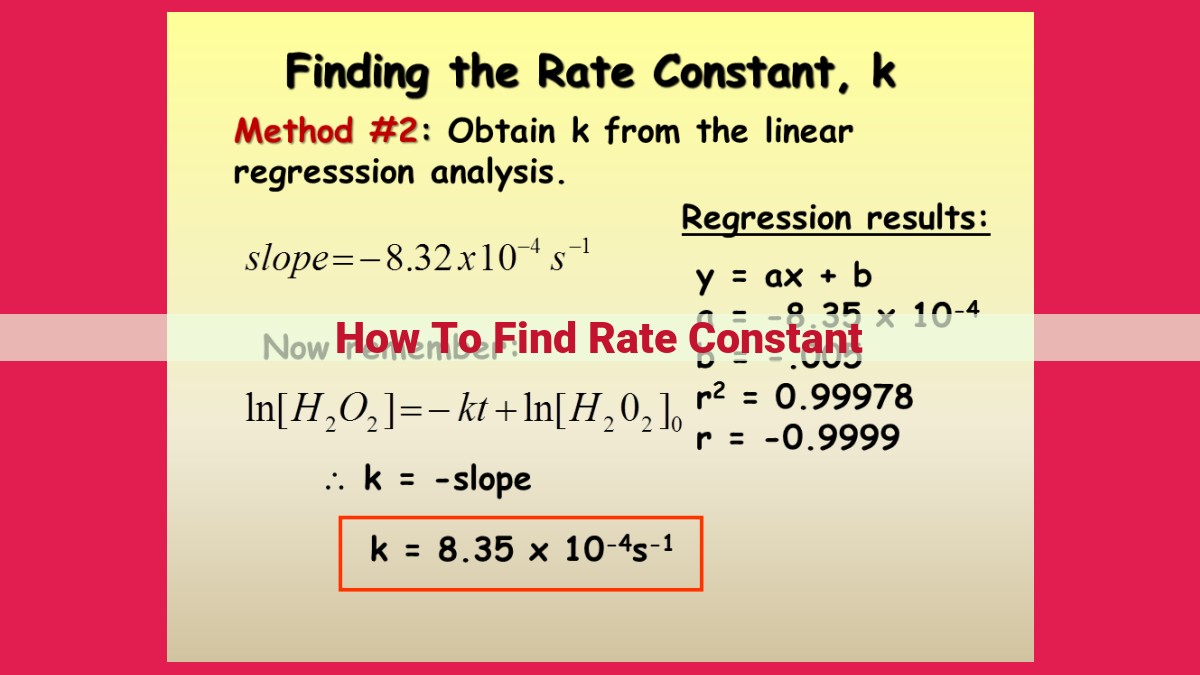
To find the rate constant, utilize the integrated rate law for the specific order of reaction. Plot the experimental data to determine the equation’s parameters, such as the slope and y-intercept. From these parameters, calculate the rate constant, which quantifies the reaction’s speed and depends on factors like temperature, activation energy, and reactant nature.

Unveiling the Secrets of Chemical Kinetics: A Journey into Reaction Dynamics

In the realm of chemistry, where atoms dance and molecules rearrange, kinetics reigns supreme as the guiding force that orchestrates the pace of these intricate transformations. Chemical kinetics, the meticulous study of reaction rates, provides invaluable insights into the complexities of chemical reactions, empowering us to understand and predict the dance of atoms.

At its core, chemical kinetics unravels the intricate interplay between reactants and products, deciphering the variables that govern their meeting, collision, and subsequent transformation. Key concepts, such as the rate constant, which quantifies the reaction’s velocity, and the half-life, which measures the time it takes for half of the reactants to vanish, lay the foundation for our kinetic understanding. Furthermore, the order of reaction illuminates the relationship between reactant concentrations and the reaction rate, unveiling the fundamental choreography of chemical transformations.

First-Order Reactions: Demystifying the Simplified Reaction Dynamics

In the realm of chemical kinetics, first-order reactions stand out for their simplicity and fundamental significance. These reactions, characterized by their direct proportionality between reaction rate and the concentration of a single reactant, provide a crucial starting point for understanding more complex reaction mechanisms.

Imagine a chemical reaction where the concentration of a reactant, let’s call it A, gradually decreases over time. In a first-order reaction, the rate at which A disappears is solely dependent on its own concentration. This means that doubling the concentration of A will double the reaction rate, and halving the concentration will halve the rate.

Half-life: A key concept in first-order reactions is the half-life, which refers to the time it takes for the concentration of the reactant to decrease by exactly half. The half-life remains constant throughout the reaction, regardless of the initial concentration of A. This remarkable property makes first-order reactions highly predictable and allows scientists to estimate the time required for a reaction to reach a specific point of completion.

Second-Order Reactions: Unraveling the Dynamics of Chemical Interactions

In the realm of chemical kinetics, reactions take on a diverse range of complexities. Second-order reactions stand out as fascinating examples where the reaction rate is proportional to the square of the concentration of one or both reactants.

Imagine a chemical dance where molecules collide and interact. In a second-order reaction, every molecule has a special partner, and the reaction occurs when these specific pairs encounter each other. Unlike its first-order counterpart, where the rate solely depends on a single reactant, here, the number of successful collisions between specific pairs of reactants governs the overall reaction rate.

Initial concentrations play a crucial role in second-order reactions. With a higher concentration of reactants, there are more chances for these specific pairs to collide, leading to a faster reaction rate. Conversely, lower concentrations result in fewer collisions and a slower reaction.

Rate constants, unique to each reaction, determine the likelihood of a successful collision leading to a reaction. These constants reflect the efficiency of the reaction and can vary significantly based on factors such as temperature, solvent, and the specific nature of the reactants.

Understanding the dynamics of second-order reactions provides insights into various chemical phenomena. For instance, in enzyme-catalyzed reactions, enzymes act as matchmakers, facilitating specific molecular collisions and accelerating reaction rates. Additionally, second-order reactions are essential in understanding autocatalytic reactions, where the product itself acts as a catalyst, leading to an exponential increase in the reaction rate over time.

Example: Consider the reaction between hydrogen (H2) and iodine (I2) to form hydrogen iodide (HI), a classic example of a second-order reaction. The reaction rate law is given as:

Rate = k[H2][I2]

This equation clearly demonstrates the quadratic dependence of the reaction rate on the concentrations of both H2 and I2.

In conclusion, second-order reactions offer a deeper understanding of complex chemical interactions, where the dance between molecules and the efficiency of their collisions dictate the overall reaction rate. By exploring their dynamics, scientists can unravel the intricate mechanisms that govern chemical processes in various fields, from industrial chemistry to biological systems.

Rate Constant: The Key to Measuring Reaction Speed

• Explain the role of the rate constant in quantifying the speed of a chemical reaction.
• Discuss factors that influence the rate constant, including temperature, activation energy, and the nature of reactants.

Rate Constant: The Key to Unlocking the Secrets of Reaction Speed

In the vast world of chemical reactions, understanding their rates is crucial for unraveling the intricate dance of molecules and predicting their outcomes. The rate constant, a fundamental parameter in chemical kinetics, holds the key to unlocking this knowledge.

The rate constant, often symbolized as k, serves as a quantitative measure of the reaction speed. It provides a precise value that describes the rate of change in the concentration of reactants over time. A higher rate constant indicates a faster reaction, while a lower rate constant signifies a slower reaction.

Several factors exert a profound influence on the rate constant. One of the most influential is temperature. As temperature rises, the energy of molecules increases, leading to more frequent and energetic collisions. This surge in collisions translates into a higher rate constant and, thus, a faster reaction.

Activation energy, an inherent property of each reaction, also plays a significant role. It represents the minimum energy barrier that reactants must overcome to transform into products. Reactions with lower activation energies have higher rate constants and proceed more swiftly than those with higher activation energies.

The nature of the reactants themselves also affects the rate constant. Larger and more complex molecules tend to have lower rate constants due to increased steric hindrance and the need for specific orientations during collisions.

Understanding the rate constant is essential for predicting the behavior of chemical reactions and optimizing industrial processes. In the realm of drug development, for example, modulating the rate of reactions can significantly impact the effectiveness and safety of medications.

In conclusion, the rate constant is an indispensable tool in chemical kinetics, providing a window into the dynamics of reactions and enabling us to unravel the intricacies of the molecular world. By comprehending the factors that govern the rate constant, we gain a deeper appreciation for the fascinating interplay of molecules and chemical transformations.

Half-Life: Monitoring Reaction Progress

In the ever-evolving world of chemical reactions, understanding the pace at which they unfold is crucial. Enter the concept of half-life, a pivotal measure that unveils the dynamics of reacting chemicals.

Definition:

Half-life is the time it takes for the concentration of a reactant to decrease by half. It represents the point at which half of the initial reactant molecules have undergone transformation. This invaluable metric allows scientists to track the progress of reactions and gain insights into their mechanisms.

Relationship to Rate Constant:

Half-life exhibits a direct relationship with the rate constant, a fundamental parameter that quantifies the speed of a reaction. For first-order reactions, where the reaction rate is proportional to the concentration of a single reactant, the half-life is inversely proportional to the rate constant.

Significance:

Monitoring half-life provides valuable information about:

• Reaction Rate: A shorter half-life indicates a faster reaction rate, while a longer half-life implies a slower rate.
• Reaction Mechanism: The half-life can help identify the order of the reaction, providing clues about the number of reactant molecules involved in the rate-determining step.
• Predicting Completion Time: By knowing the half-life, scientists can estimate the time required for a reaction to reach completion or a desired endpoint.

Formula and Calculation:

The half-life for first-order reactions can be calculated using the following formula:

t₁/₂ = (ln 2) / k

where:

• t₁/₂ is the half-life
• ln is the natural logarithm
• k is the rate constant

In conclusion, half-life plays a vital role in understanding the kinetics of chemical reactions. By monitoring half-life, scientists can unravel the intricacies of reaction progress, predict completion times, and gain insights into the fundamental mechanisms that drive these transformative processes.

Unveiling the Arrhenius Equation: Unlocking the Temperature-Reaction Rate Enigma

In the realm of chemical reactions, the Arrhenius equation stands as a beacon of understanding, illuminating the profound influence of temperature on reaction rates. This equation, formulated by the Swedish chemist Svante Arrhenius, provides a window into the intricate dance between temperature and the speed of chemical transformations.

The Arrhenius equation reads as follows:

k = Ae^(-Ea/RT)

where:

• k is the rate constant, a measure of the reaction rate
• A is the pre-exponential factor, a constant that represents the frequency of collisions between reactants
• Ea is the activation energy, the minimum energy required for a reaction to proceed
• R is the universal gas constant (8.314 J/(mol K))
• T is the absolute temperature in Kelvin

Breaking Down the Arrhenius Equation

The pre-exponential factor, A, reflects the collision frequency, the number of times reactants collide per unit time. Higher values of A indicate more frequent collisions, leading to a faster reaction rate.

The activation energy, Ea, represents an energy barrier that reactants must overcome in order to react. Reactions with higher activation energies require more energy and therefore proceed at slower rates.

Temperature’s Impact on Reaction Rates

The Arrhenius equation reveals that temperature has a profound impact on reaction rates. As temperature increases, the exponential term in the equation decreases, causing the rate constant k to increase. This means that chemical reactions tend to proceed faster at higher temperatures.

Intuitively, this makes sense. Higher temperatures provide reactants with more kinetic energy, enabling them to collide with greater force and overcome the activation energy barrier more easily.

Applications and Implications

The Arrhenius equation finds widespread use in various fields, including:

• Predicting reaction rates in chemical processes
• Optimizing industrial reactions by manipulating temperature
• Understanding enzyme activity and biological processes
• Determining the shelf life of perishable goods

By harnessing the power of the Arrhenius equation, scientists and engineers can gain valuable insights into the behavior of chemical reactions and tailor them for specific applications.

Activation Energy: The Obstacle to Overcome

In the captivating realm of chemical reactions, there exists a formidable barrier known as activation energy. It represents the minimum amount of energy required for a reaction to proceed. Imagine reactants as tiny hikers, eager to ascend the towering mountain of activation energy before they can embark on their transformative journey.

The Impact on Reaction Rates

Activation energy has a profound influence on the speed of chemical reactions. Think of it as the height of the mountain that the reactants must climb. The higher the activation energy, the more difficult it is for the reactants to reach the summit and complete the reaction. This results in slower reaction rates.

Selectivity of Reactions

But activation energy plays another crucial role: it determines the selectivity of reactions. When multiple reaction pathways are available, the reactants will favor the path with the lowest activation energy. This is because they are more likely to reach the summit and complete the reaction along that path. Thus, activation energy helps shape the outcome of chemical reactions.

Understanding Activation Energy

Activation energy arises from the need to break existing bonds and form new bonds during a chemical reaction. The stronger the bonds that need to be broken, the higher the activation energy. Additionally, the geometry and orientation of the reactants can also influence activation energy.

Overcoming the Barrier

Chemists have developed various strategies to overcome the challenge posed by activation energy. One common approach is to use catalysts. These substances provide an alternative pathway for the reaction, with a lower activation energy, making it easier for the reactants to complete the reaction.

Collision Theory: A Statistical Perspective on Reaction Rates

Delve into the Molecular Realm of Chemical Reactions

Chemical reactions, the dance of molecules, hold the key to countless transformations in our world. To understand this intricate choreography, scientists have developed theories that provide a statistical lens into the microscopic universe where reactions unfold. One such theory is collision theory, a fundamental concept that sheds light on the intricate mechanisms governing reaction rates.

The Role of Molecular Collisions

Collision theory posits that chemical reactions occur when reactant molecules collide with sufficient energy and the correct orientation. This collision provides the necessary activation energy, the energetic hurdle that must be overcome for a reaction to proceed.

Frequency and Orientation: Decisive Factors

The probability of a successful collision depends on two crucial factors: collision frequency and collision orientation. Collision frequency represents the number of collisions occurring per unit time. The more frequent the collisions, the higher the likelihood of a reaction.

Optimizing Orientation for Reaction Success

However, not all collisions lead to reactions. The orientation of the colliding molecules is also critical. Only collisions that allow the reactants to align in a way that facilitates bond formation will result in a reaction.

Collision Theory: A Simplified Perspective

While collision theory provides a foundational understanding of reaction rates, it remains a simplified model. It does not account for the complex interactions between molecules and assumes that all collisions have equal probability of leading to a reaction.

Beyond Collision Theory: Exploring More Refined Models

Despite its limitations, collision theory serves as a stepping stone in the evolution of chemical kinetics. More advanced theories, such as transition state theory, provide a more sophisticated picture of reaction mechanisms and incorporate factors like the formation of an intermediate state and the role of quantum effects.

The Interplay of Theories: A Holistic Approach

Collision theory and transition state theory, along with other kinetic models, form a complementary suite of tools that allow scientists to unravel the intricacies of chemical reactions. By combining these perspectives, we gain a deeper understanding of the molecular dance that drives chemical transformations.

Transition State Theory: Unraveling the Secrets of Chemical Reactions

In the intricate world of chemical reactions, the transition state holds the key to understanding how molecules transform from one form to another. Imagine a hiker traversing a treacherous mountain pass. The transition state represents the peak of this pass, where the hiker encounters the highest energy barrier before descending to the other side of the mountain.

Transition state theory provides a refined picture of chemical reactions by describing the unstable, high-energy intermediate formed when reactants come together. This intermediate is a fleeting glimpse of the activated complex, where bonds are breaking and forming simultaneously.

Transition state parameters, such as the transition state structure and transition state energy, offer valuable insights into reaction mechanisms and reaction rates. By studying these parameters, chemists can unravel the intricate dance of молекулы as they undergo chemical transformations.

Transition state theory not only helps us understand the dynamics of chemical reactions but also has practical implications. It enables chemists to design and optimize catalysts, which accelerate reactions by lowering the transition state energy. This knowledge is essential in various fields, from drug development to industrial chemistry.

In essence, transition state theory provides a powerful lens through which we can observe and understand the hidden workings of chemical reactions. By uncovering the secrets of the transition state, we gain a deeper appreciation for the intricate symphony of molecular interactions.

Microscopic Reversibility: Balancing the Scales

• Discuss the concept of microscopic reversibility and its implications for chemical reactions.
• Explain how collision theory and transition state theory contribute to understanding microscopic reversibility.

Microscopic Reversibility: The Balancing Act of Chemical Reactions

In the realm of chemical reactions, a fascinating concept known as microscopic reversibility plays a key role in understanding how reactions proceed and achieve equilibrium. This principle states that every forward reaction, where reactants transform into products, has a corresponding reverse reaction where products revert back to reactants. This reversibility is not just a theoretical notion but is rooted in the very laws of physics that govern chemical interactions.

Collision Theory: The Dance of Molecules

To grasp the essence of microscopic reversibility, we must delve into the microscopic world of molecules. Collision theory provides a statistical framework to describe how chemical reactions occur. According to this theory, reactions happen when molecules collide with sufficient energy and in the correct orientation. These collisions create a transition state, a high-energy intermediate where the reactants are poised to rearrange into products or revert back to reactants.

Transition State Theory: Capturing the Transition

The concept of the transition state is central to understanding microscopic reversibility. Transition state theory offers a detailed look at this fleeting intermediate. It considers the energy barrier that molecules must overcome to undergo a reaction and the probability of successful collisions.

The Balancing Act

Microscopic reversibility implies that the rates of forward and reverse reactions are equal at equilibrium. This equilibrium point represents a dynamic balance, where the number of molecules converting from reactants to products matches the number reverting back to reactants. This balance is not static but rather a constant dance of molecules, perpetually shifting between their reactant and product forms.

The Role of Thermodynamics

The principle of microscopic reversibility is deeply connected to the second law of thermodynamics, which states that entropy, a measure of disorder, always increases in isolated systems. In chemical reactions, the forward reaction typically involves a decrease in entropy, as reactants become more organized into products. The reverse reaction, on the other hand, brings the system closer to equilibrium, where entropy is maximized.

Implications for Understanding Reactions

Microscopic reversibility is not merely an abstract concept but has profound implications for our understanding of chemical reactions. It allows us to predict the direction and extent of reactions, as well as the equilibrium concentrations of reactants and products. This principle is an essential tool in fields such as chemical engineering, biochemistry, and pharmaceutical development.

The concept of microscopic reversibility adds a layer of symmetry and elegance to the study of chemical reactions. It provides a profound insight into the dynamic nature of equilibrium and the underlying principles that govern molecular interactions. By embracing this principle, we gain a deeper appreciation for the intricate dance of molecules that shapes the chemical world around us.