Degenerate Orbitals: Significance In Chemistry And Quantum Mechanics

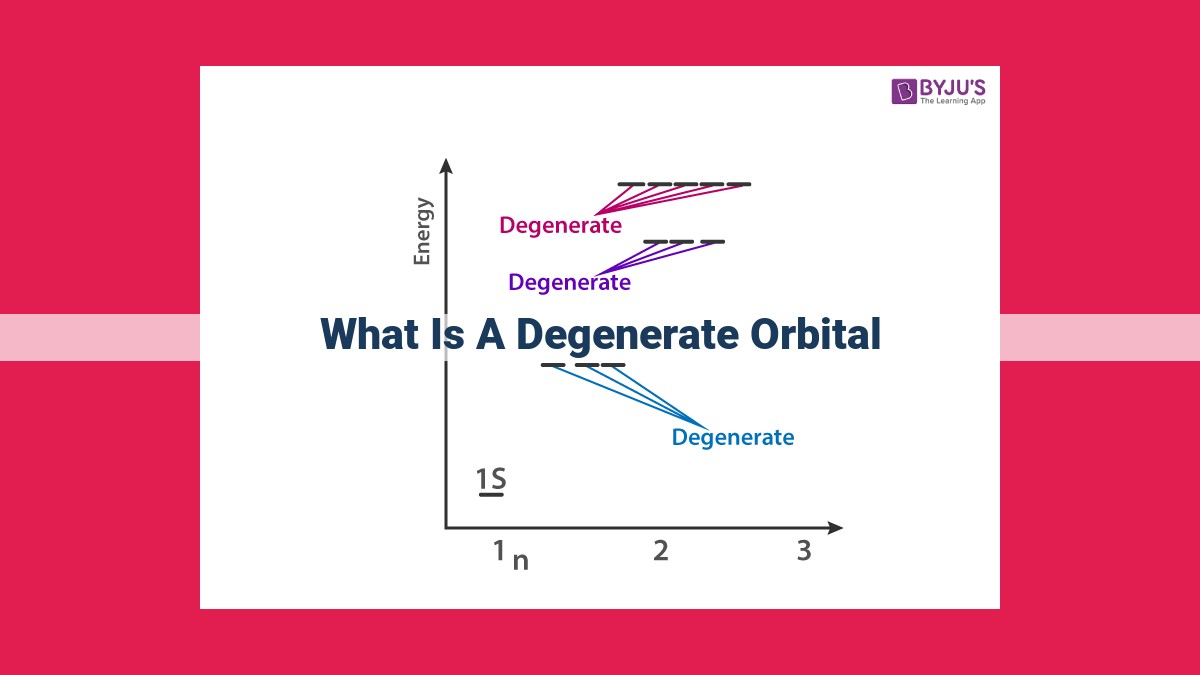
Degenerate orbitals are orbitals within an atomic shell that share the same energy level. This occurs when the orbitals have identical shapes and orientations but differ in energy quanta or magnetic quantum numbers. Hund’s rule states that electrons occupy degenerate orbitals singly before pairing, maximizing the number of unpaired electrons. The Pauli exclusion principle allows for two electrons to occupy a degenerate orbital only if their spins are opposite, leading to a unique electron configuration. Degenerate orbitals have significant implications for chemical bonding and influence the electronic properties of atoms and molecules.

Definition of Degenerate Orbitals

• Explain that degenerate orbitals have the same energy level within an atomic shell.

Unveiling the Secrets of Degenerate Orbitals: A Tale of Energy and Symmetry

Within the intricate tapestry of atomic structure, there exists a fascinating phenomenon known as degenerate orbitals. These enigmatic entities possess an uncanny ability to share the same energy level within an atomic shell, leading to a captivating dance of symmetries and interactions.

Imagine an energy level as a bustling city, where electron orbitals are the bustling apartments, each with its own unique address. In the realm of degenerate orbitals, multiple apartments share the same energy level, creating a neighborhood of electron realms that are indistinguishable in terms of their energy.

This phenomenon arises from the inherent symmetry of atomic orbitals. Take, for instance, the three p-orbitals within an electron shell. These orbitals, shaped like dumbbells, point along the x, y, and z axes, respectively. Due to their identical shapes and orientations, they all possess the same energy. This kinship forms a degenerate trio, sharing the same energy address within the atomic shell.

Degeneracy and Energy Levels in Atomic Orbitals

In the realm of quantum chemistry, electrons within an atom occupy specific energy levels. These energy levels are further divided into sublevels or orbitals. Degenerate orbitals are a fascinating concept that arises when orbitals within the same energy level possess equal energy.

Consider the case of the three p-orbitals in a given energy level. Each of these orbitals has a unique shape and orientation, but their energies are identical. This equality in energy is known as degeneracy. In other words, these three p-orbitals are degenerate with respect to each other.

Degeneracy occurs when there are multiple orbitals with equivalent energy within a specific energy level. These orbitals may differ in their spatial orientations, but they are indistinguishable in terms of their energy. The number of degenerate orbitals in a given energy level depends on the shape and symmetry of the orbital.

For instance, the p-orbitals, denoted as px, py, and pz, are all degenerate within the same energy level. They have different orientations along the x, y, and z axes, respectively, but their energies are the same. Similarly, the five d-orbitals in a given energy level are also degenerate among themselves.

Hund’s Rule and the Stability of Degenerate Orbitals

Degenerate orbitals are atomic orbitals that have the same energy level within a given shell. When electrons fill these orbitals, Hund’s rule comes into play.

Hund’s rule states that electrons will distribute themselves among degenerate orbitals in such a way as to maximize the number of unpaired electrons. This is because unpaired electrons have more energy than paired electrons, leading to a more stable configuration.

For example, consider the three p-orbitals in an energy level. Each p-orbital can hold a maximum of two electrons. According to Hund’s rule, the electrons will fill these orbitals in a way that maximizes the number of unpaired electrons. This means that the electrons will first occupy each p-orbital with one unpaired electron before pairing up.

The stability from Hund’s rule arises from the exchange interaction. When electrons are unpaired, they can exchange spins, which lowers the overall energy of the system. This exchange interaction is absent when electrons are paired, which is why paired electrons have higher energy.

Hund’s rule has important implications for the electronic properties of atoms and molecules. It helps determine the magnetic properties of atoms, as unpaired electrons contribute to the overall magnetic moment. It also affects the chemical bonding behavior of atoms, as the number of unpaired electrons can influence the formation of chemical bonds.

The Pauli Exclusion Principle and Degenerate Orbitals

In the quantum world of atoms, electrons dance around the nucleus in designated energy levels known as orbitals. These orbitals are like tiny clouds with specific shapes and orientations. When orbitals within the same energy level have the same energy, they are called degenerate orbitals.

The Pauli exclusion principle is a fundamental law of nature that governs the behavior of electrons. It states that no two electrons in an atom can have the exact same set of quantum numbers. This means that electrons can’t occupy the same orbital with the same spin, a property that describes their intrinsic angular momentum.

However, in the case of degenerate orbitals, the Pauli exclusion principle doesn’t apply entirely. Electrons can occupy the same degenerate orbital as long as they have **opposite spins. This is a fascinating exception to the rule, allowing multiple electrons to reside in the same energy space without violating the Pauli principle.

Imagine a pair of dancers performing on a stage. They occupy the same physical space but move in opposite directions. Similarly, electrons in degenerate orbitals share the same energy level but “dance” with opposite spins, effectively “avoiding” each other.

Implications of Degenerate Orbitals

The presence of degenerate orbitals within an atomic shell unlocks a realm of possibilities that profoundly impact chemical bonding and the electronic properties of atoms and molecules.

Impact on Chemical Bonding

Degenerate orbitals play a crucial role in determining the molecular geometry and bonding properties of molecules. By accommodating electrons with the same energy level, degenerate orbitals can lead to:

• Multiple bonds: The formation of double and triple bonds between atoms is made possible by the availability of degenerate orbitals. These additional bonds enhance the stability and reactivity of molecules.
• Hybridization: Degenerate orbitals can hybridize, combining to form new atomic orbitals with distinct shapes and energy levels. Hybridization enables the formation of various molecular geometries, such as linear, trigonal planar, and tetrahedral.

Influence on Electronic Properties

Furthermore, degenerate orbitals exert a significant influence on the electronic properties of atoms and molecules:

• Magnetic properties: Electrons in degenerate orbitals can have parallel spins, leading to paramagnetism. This property is essential in the study of magnetism and materials science.
• Electronic conductivity: The presence of degenerate orbitals can enhance the electronic conductivity of materials. By providing pathways for electron movement, degenerate orbitals facilitate the flow of electricity.
• Optical properties: The energy difference between degenerate orbitals can affect the absorption and emission of light by atoms and molecules. This plays a role in determining the color and optical properties of materials.

In summary, degenerate orbitals are pivotal in shaping the electronic structure and properties of atoms and molecules. Their impact extends to chemical bonding, magnetic behavior, electronic conductivity, and optical phenomena, making them essential for understanding the chemistry and physics of our world.