Definitive Guide To Measuring, Bisecting, And Calculating Angles With Proficient Angle Techniques
To measure an angle, begin by using a protractor to align its center with the angle’s vertex and the 0° mark with one side of the angle. Locate the point where the other side of the angle intersects the protractor’s scale to determine the angle’s measure. To bisect an angle, construct a protractor-based or compass-drawn perpendicular bisector, which will intersect the angle at its midpoint. Use angle addition/subtraction postulates to calculate unknown angle measures by combining or subtracting known angles. Identify special angle pairs like vertical (equal), supplementary (sum 180°), and complementary (sum 90°) angles. Finally, consider adjacent angles that share a vertex and side, with their measures always summing up.
- Explain the importance of angle measurement in everyday life and various fields.
- Define an angle and its units of measurement (degrees, minutes, seconds).
In the tapestry of our world, angles weave intricate patterns, shaping everything from the towering skyscrapers that grace our cities to the delicate petals of a blooming flower. Measuring angles is an essential skill, with applications that span countless fields, from engineering and astronomy to architecture and art.
An angle, the geometric construct that represents the divergence of two intersecting lines or rays, is measured in degrees, minutes, and seconds. These units allow us to quantify the gap between diverging lines and describe the orientations of objects in space. In everyday life, we encounter angles everywhere we turn: in the hands of a clock, the slope of a ramp, and even the trajectory of a thrown ball.
Understanding how to measure angles unlocks a wealth of possibilities. It enables us to navigate our surroundings, design functional structures, and appreciate the beauty of the world around us. Whether you’re a budding engineer, an aspiring artist, or simply someone curious about the hidden geometry that shapes our lives, mastering angle measurement is a key to unlocking the world of spatial understanding.
Tools for Angle Measurement: Unraveling the Secrets of the Protractor
In the realm of mathematics, where precision reigns supreme, the measurement of angles holds a pivotal position. And when it comes to accurately determining angles, the protractor stands as an indispensable tool.
Unveiling the Protractor’s Essence
Picture a semicircular device, adorned with intricate markings—this is the protractor. Its primary purpose lies in measuring angles, whether on paper or in real-world scenarios. The markings on a protractor are not mere decorations; they embody a wealth of information.
Deciphering the Protractor’s Markings
Each protractor is meticulously calibrated, bearing degree markings that typically range from 0° to 180°. The 0° mark aligns with the straight edge of the protractor, while the 180° mark resides on the opposite end of the semicircle.
Navigating the Protractor’s Intricacies
To harness the power of a protractor, it’s imperative to understand how to correctly position it. Align the protractor’s straight edge along one side of the angle you wish to measure, ensuring that the vertex of the angle coincides with the center of the semicircle.
With Precision, We Measure
Now, embark on the quest to ascertain the angle’s measure. Locate the point where the other side of the angle intersects the protractor’s markings. The degree marking at this point will reveal the angle’s measure.
Embracing the Protractor’s Versatility
The protractor’s unparalleled accuracy makes it the go-to tool for a multitude of tasks. From architectural blueprints to carpentry projects, from trigonometry problems to scientific experiments, the protractor empowers us with precise angle measurements, unlocking countless possibilities.
Harnessing the Protractor’s Potential
Whether you’re a seasoned mathematician or a curious explorer of the world of angles, the protractor serves as your faithful ally. Its simplicity and precision make it an indispensable tool for anyone seeking to unlock the secrets of angular measurement.
Angle Bisector and Perpendicular Bisector: Understanding Angle Bisection
In the realm of angles, there are two special lines that play a crucial role in dividing angles: the angle bisector and the perpendicular bisector. Let’s dive into their world!
Angle Bisector: The Equal-Angle Splitter
An angle bisector is a line or ray that divides an angle into two equal parts. Imagine a pie that you want to share equally with a friend. An angle bisector is like the cut that splits the pie into two identical slices.
Constructing an Angle Bisector:
- Protractor Method: Place the protractor’s center at the vertex of the angle. Align the baseline with one ray of the angle.
- Compass Method: Place the compass at the vertex and draw an arc that intersects both rays of the angle. Repeat from the other ray to create two intersecting arcs. The point where the arcs cross is the vertex of the angle bisector.
Perpendicular Bisector: The 90° Line
A perpendicular bisector is a line that intersects a line segment at its midpoint and forms a right angle (90°) with it. It’s like a special ruler that measures the perpendicular distance between a point and a line.
Properties of a Perpendicular Bisector:
- It divides the line segment into two equal parts.
- It forms right angles with both halves of the line segment.
- It passes through the midpoint of the line segment.
Understanding Angle Addition and Subtraction Postulates
In the realm of geometry, angles play a pivotal role in shaping our understanding of the world around us. From the intricate designs of architecture to the precise calculations of science, angles help us comprehend the nature of shapes, objects, and even the universe itself. Among the fundamental principles that govern angle measurements are the Angle Addition and Subtraction Postulates, which form the cornerstone of angle calculations.
The Angle Addition Postulate
The Angle Addition Postulate states that if two rays, Ray AP and Ray BP, share a common endpoint (point P), then the measure of the angle formed by these rays is equal to the sum of the measures of the two individual angles. Mathematically, we can represent this as:
m∠APB = m∠APC + m∠BPC
Consider a simple example. Suppose you have a straight line, Line AB. If you draw a ray Ray CP from point C on the line that intersects Line AB at point P, we have created two angles, ∠APC and ∠BPC. According to the Angle Addition Postulate, the measure of ∠APB is equal to the sum of the measures of ∠APC and ∠BPC.
The Angle Subtraction Postulate
The Angle Subtraction Postulate is closely related to the Angle Addition Postulate. It states that if a ray, Ray DQ, intersects two other rays, Ray DP and Ray DQ, forming two angles, then the measure of one angle is equal to the measure of the other angle minus the measure of the angle formed by the two intersecting rays. Mathematically, we can write this as:
m∠DPQ = m∠DPR - m∠PQR
To illustrate this concept, imagine a scenario where you have a line, Line RS. From point P on the line, you draw two rays, Ray DP and Ray DQ, which intersect another line, Line TS, at points D and Q, respectively. According to the Angle Subtraction Postulate, the measure of ∠DPQ is equal to the measure of ∠DPR minus the measure of ∠PQR.
Applications of the Angle Addition and Subtraction Postulates
The Angle Addition and Subtraction Postulates are essential tools for solving a wide range of angle measurement problems. They allow us to determine the measures of unknown angles by combining or subtracting known angles. These postulates are particularly useful in fields such as trigonometry, surveying, architecture, and engineering, where accurate angle measurements are crucial. By understanding and applying these postulates, we can confidently navigate the world of angles and gain a deeper appreciation for the intricate geometry that surrounds us.
Special Pairs of Angles
- Define vertical angles and their characteristics of equal measures.
- Define supplementary angles and their property of summing up to 180°.
- Define complementary angles and their property of summing up to 90°.
Special Pairs of Angles
Angles play a crucial role in our understanding of geometry and spatial relationships. Special pairs of angles emerge when specific conditions are met, providing valuable insights into angle measurement.
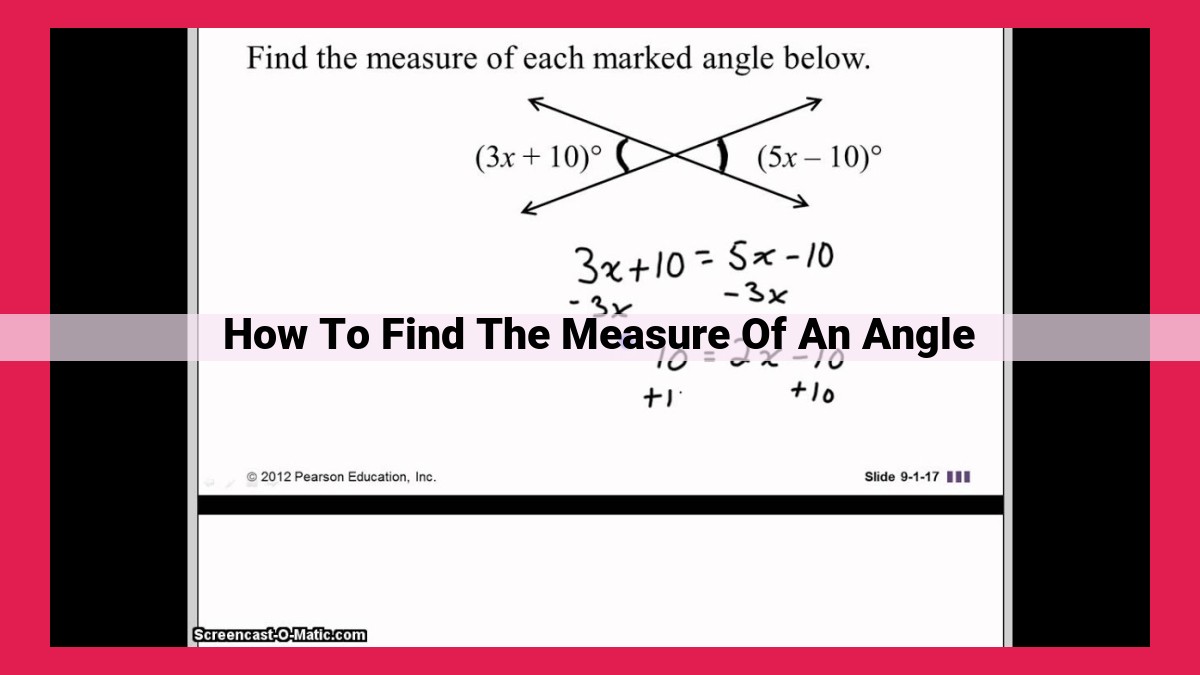
Vertical Angles
Imagine a straight line intersecting another straight line to form an “X” shape. The angles formed opposite each other at the intersection point are called vertical angles. These angles are always equal in measure. This is because the sum of the angles around a point is always 360 degrees, and the vertical angles divide this sum evenly between them.
Supplementary Angles
When two adjacent angles add up to 180 degrees, they are referred to as supplementary angles. Picture yourself standing at a corner of a room. The angle between the wall and one side of the room is adjacent to the angle between the wall and the other side of the room. Together, these angles make a total of 180 degrees, allowing you to turn around and face the opposite direction.
Complementary Angles
Finally, we have complementary angles. These are angles that add up to 90 degrees. They are often found in perpendicular lines, where one line meets another at a right angle. Imagine a T-junction in a road. The two angles formed by the main road and the intersecting road are complementary, allowing for smooth turns without collisions.
Adjacent Angles: Unveiling the Relationship Between Intertwined Angles
In the realm of geometry, angles play a pivotal role, not only in theoretical calculations but also in everyday life. Whether you’re designing a building, navigating a road, or measuring the trajectory of a ball in a game, angles serve as indispensable tools. Among the many types of angles, adjacent angles hold a unique significance due to their intertwined nature.
Defining Adjacent Angles
Adjacent angles are those that share a common vertex and a side. Imagine two intersecting lines forming four angles around the point where they meet. The angles that lie side-by-side, sharing the same vertex and side, are known as adjacent angles.
Relationship Between Adjacent Angles
The relationship between adjacent angles is beautifully characterized by their sum. If you take any two adjacent angles, you’ll find that their sum is always 180 degrees. This property is known as the Adjacent Angle Theorem.
Simplified Navigation of Angle Calculations
Understanding this theorem greatly simplifies the navigation of angle calculations. If you know the measure of one adjacent angle, you can easily find the measure of the other by simply subtracting it from 180 degrees. For example, if one adjacent angle measures 60 degrees, its adjacent angle would measure 180 – 60 = 120 degrees.
Real-World Applications
The concept of adjacent angles has numerous practical applications. In architecture, understanding adjacent angles helps determine the shape and dimensions of buildings, ensuring structural stability and aesthetic appeal. In surveying, measuring adjacent angles allows for accurate land measurement and property demarcation. Even in sports, the trajectory of a ball or the angle at which a player strikes it can be determined using the principles of adjacent angles.
Adjacent angles, with their intrinsic relationship and interconnected measures, form a cornerstone of geometry. Their practical applications extend far beyond the classroom, demonstrating the power of mathematical concepts in shaping our world. By understanding the sum of adjacent angles, we not only strengthen our mathematical foundation but also gain a valuable tool for problem-solving and everyday calculations.