Calculating The Critical Angle For Total Internal Reflection

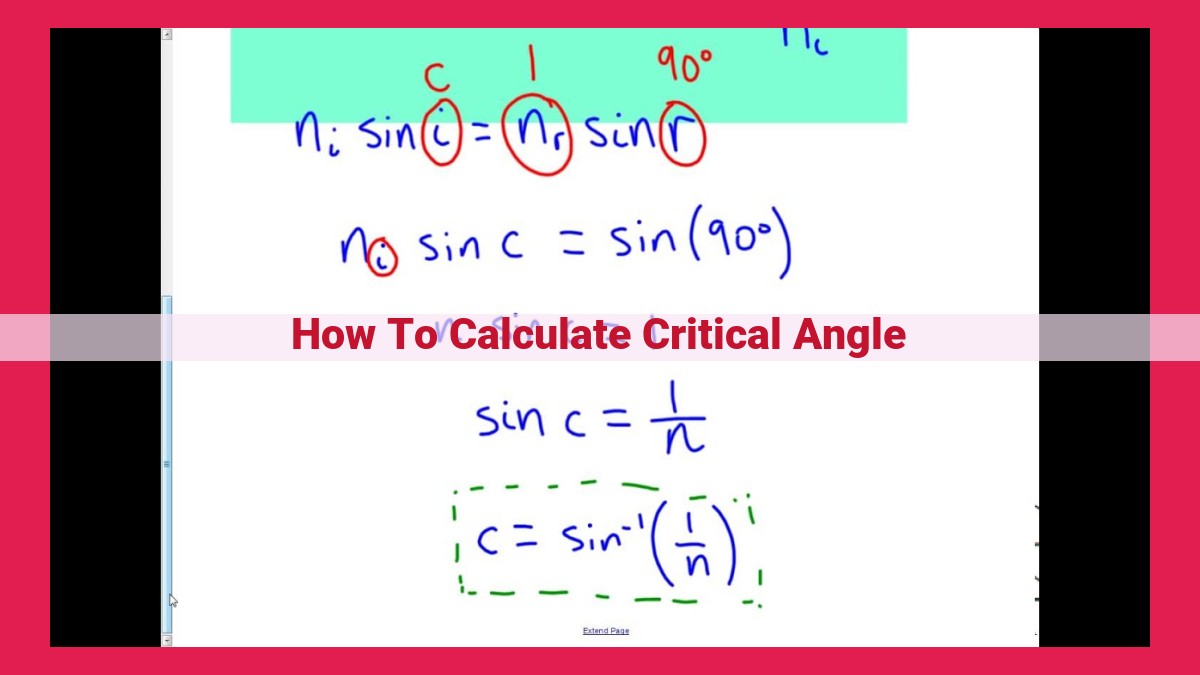
To calculate the critical angle, start with Snell’s Law: sin(i)/sin(r) = n2/n1, where i is the angle of incidence, r is the angle of refraction, n1 is the index of refraction of the denser medium, and n2 is the index of refraction of the less dense medium. At the critical angle (θc), total internal reflection occurs, and r = 90°. Substituting this into Snell’s Law gives sin(θc) = n2/n1. Therefore, the critical angle is calculated as θc = sin^-1(n2/n1).

• Explanation of what the critical angle is and its significance in optics.

Understanding the Critical Angle: A Journey into Light’s Bending Odyssey

In the realm of optics, there exists a fascinating concept known as the critical angle. It plays a pivotal role in determining the behavior of light as it interacts with different materials, paving the way for a myriad of optical phenomena and applications.

Unveiling the Critical Angle: A Threshold for Light’s Passage

The critical angle is a specific angle of incidence, measured from the normal to the interface between two materials, beyond which light undergoes a remarkable transformation known as total internal reflection. This phenomenon occurs when light, traveling from a denser medium to a less dense medium, encounters a critical angle at which it is completely reflected back into the denser medium.

Snell’s Law: The Guiding Principle for Light’s Bending

Understanding the critical angle requires a deeper exploration of Snell’s Law. This fundamental law describes the relationship between the angles of incidence and refraction of light as it crosses the boundary between two materials with different refractive indices.

Refractive index is a measure of how much light bends when passing through a material. The greater the refractive index, the more the light bends. According to Snell’s Law, the ratio of the sine of the incident angle (angle of light entering the material) to the sine of the refracted angle (angle of light exiting the material) is equal to the ratio of the refractive indices of the two materials.

Total Internal Reflection: When Light Bounces Back

As light encounters an interface between two materials with different refractive indices, its behavior is governed by Snell’s Law. However, when the angle of incidence exceeds the critical angle, a unique phenomenon occurs. Instead of being refracted into the less dense medium, the light is completely reflected back into the denser medium.

Total internal reflection finds its applications in diverse fields, including fiber optic cables, prisms, and microscopy. It’s a fundamental principle upon which many optical instruments and technologies are built.

Snell’s Law: Understanding the Relationship Between Angles and Indices of Refraction

• Description of Snell’s Law equation and its role in calculating angles of incidence and refraction.
• Explanation of how indices of refraction affect light bending.

Snell’s Law: Unlocking the Secrets of Light Bending

In the world of optics, the mystery of light bending is a fundamental concept. Snell’s Law plays a pivotal role in deciphering this phenomenon, offering a mathematical formula that enables us to calculate the angles at which light changes direction when passing from one material to another.

The Equation of Enlightenment

Snell’s Law, first discovered by the Dutch mathematician Willebrord Snellius, is expressed by the equation:

sin θi / sin θr = n2 / n1

where:

• θi is the angle of incidence (the angle at which light strikes a surface)
• θr is the angle of refraction (the angle at which light bends after passing through the surface)
• n1 is the index of refraction of the first material (the material from which light originates)
• n2 is the index of refraction of the second material (the material into which light enters)

Indices of Refraction: Gatekeepers of Light Bending

The index of refraction is a crucial parameter that determines the extent to which light bends. It represents the ratio of the speed of light in a vacuum to the speed of light in the material. A higher index of refraction implies that light travels slower in that material, resulting in a greater bending angle.

Light’s Journey Through Snell’s Equation

Snell’s Law serves as a guidebook for light’s journey across material boundaries. By understanding the interplay between angles and indices of refraction, we can predict how light will behave when transitioning from, say, air into water or glass. Additionally, this law helps us comprehend the principles behind optical devices, such as lenses and prisms, which rely on light bending to produce images and perform complex optical transformations.

Index of Refraction: A Measure of Light Bending

In the realm of optics, the index of refraction plays a pivotal role in dictating how light interacts with different materials. This numerical value quantifies the bending of light as it crosses the boundary between two mediums.

The index of refraction is defined as the ratio of the speed of light in a vacuum to its speed in the material. In simpler terms, it measures how much the material slows down light. The higher the index of refraction, the slower light travels through that material.

This phenomenon affects the direction of light as it passes from one medium to another. Light bends towards the normal (a perpendicular line to the surface) when it enters a material with a higher index of refraction, such as from air into water. Conversely, it bends away from the normal when it enters a material with a lower index of refraction, like from water into air.

The index of refraction is a crucial factor in determining the critical angle, beyond which light undergoes total internal reflection. This phenomenon occurs when light is incident on a boundary at an angle greater than the critical angle. Instead of refracting into the second medium, all the light is reflected back into the first medium.

Total Internal Reflection: Beyond the Critical Angle

Imagine you’re peeking into an underwater world. As you look through the glass of your aquarium, you notice something peculiar. Some light rays seem to bounce right off the water’s surface and reflect back into the aquarium, while others appear to vanish. This perplexing phenomenon is known as total internal reflection.

Total internal reflection occurs when light travels from a denser medium (like glass) to a less dense medium (like air) at an angle called the critical angle. At this critical angle, the light ray undergoes a complete reflection, meaning it doesn’t escape into the air but instead bounces back into the denser medium.

To understand why this happens, we need to delve into the concept of the index of refraction. Every material has a unique index of refraction, which is a measure of how much light bends when it passes through that material. When light moves from a medium with a higher index of refraction (denser) to a medium with a lower index of refraction (less dense), it bends away from the normal (imaginary line perpendicular to the surface).

The critical angle is the angle of incidence (the angle at which the light ray strikes the surface) that produces an angle of refraction of 90 degrees. In other words, the light ray is reflected back parallel to the surface. If the angle of incidence is greater than the critical angle, the light undergoes total internal reflection.

Total internal reflection has fascinating applications in the world of optics. It’s the principle behind fiber optic cables, which transmit light signals over long distances with minimal loss. It’s also used in prisms and lenses to bend and focus light rays. And it’s even employed in total internal reflection microscopy to visualize structures inside cells that would otherwise be invisible.

For instance, diamonds sparkle so brilliantly because their high index of refraction allows for total internal reflection of light to create multiple reflections within the gemstone. This phenomenon is also crucial in the cutting and shaping of diamonds, ensuring their radiant brilliance.

Calculating the Critical Angle: A Step-by-Step Guide

When light crosses a boundary between two materials with different indices of refraction, it bends, or refracts. The amount of bending depends on the angle of incidence, the angle at which the light strikes the boundary, and the indices of refraction of the two materials.

The critical angle is the angle of incidence at which the refracted light is bent so that it travels parallel to the boundary between the two materials. Beyond the critical angle, a phenomenon called total internal reflection occurs, where all the light is reflected back into the first material.

How to Compute the Critical Angle:

For a light wave traveling from a medium with a higher index of refraction (n1) to a medium with a lower index of refraction (n2), the critical angle (θc) can be calculated using the formula:

sin(θc) = n2 / n1

Understanding the Variables:

• θc: Critical angle (in degrees)
• n1: Index of refraction of the denser medium (higher index)
• n2: Index of refraction of the less dense medium (lower index)

Once you have these values, you can use the formula to calculate the critical angle.

For example, if light is traveling from water (n1 = 1.33) to air (n2 = 1.00), the critical angle would be:

sin(θc) = 1.00 / 1.33

θc = 48.75°

This means that if the angle of incidence is greater than 48.75°, the light will undergo total internal reflection and will not pass through the air-water boundary.

Applications of the Critical Angle

• Examples of real-world applications where the critical angle plays a crucial role, such as:
  • Fiber optic cables
  • Prisms and lenses
  • Total internal reflection microscopy
  • Diamond cutting

Applications of the Critical Angle: Guiding Light and Beyond

The critical angle, a pivotal concept in optics, wields its influence across a multitude of real-world applications, transforming the way we perceive and manipulate light. Its ability to confine light within specific paths has revolutionized fields as diverse as telecommunications, imaging, and even the sparkling allure of diamonds.

Fiber Optic Cables: The Information Superhighway

At the heart of our global communication networks lies fiber optic cables, where hair-thin strands of glass or plastic carry vast amounts of data. The critical angle ensures that light remains trapped within these fibers, allowing signals to travel long distances without significant loss.

Prisms and Lenses: Bending Light to Our Will

Prisms and lenses, ubiquitous in optical systems, harness the critical angle to bend and focus light. By carefully controlling the angles of incidence and refraction, these devices enable us to magnify objects, decompose light into its constituent colors, and correct for visual impairments.

Total Internal Reflection Microscopy: Illuminating the Microscopic World

In the realm of microscopy, total internal reflection microscopy (TIRM) utilizes the critical angle to illuminate biological specimens from below. This technique eliminates reflections from the specimen’s surface, providing exceptionally clear images of delicate structures.

Diamond Cutting: Unlocking the Sparkle

The critical angle also plays a crucial role in the artistry of diamond cutting. By meticulously aligning planes of cut with the diamond’s internal structure, cutters exploit the critical angle to maximize the gemstone’s brilliance and fire.

These are just a few examples of the many applications where the critical angle holds sway. Its ability to manipulate light empowers us to communicate seamlessly, explore the unseen, and adorn ourselves with the radiant beauty of gems.