Calculating Cosine In Triangles: Methods And Applications In Trigonometry
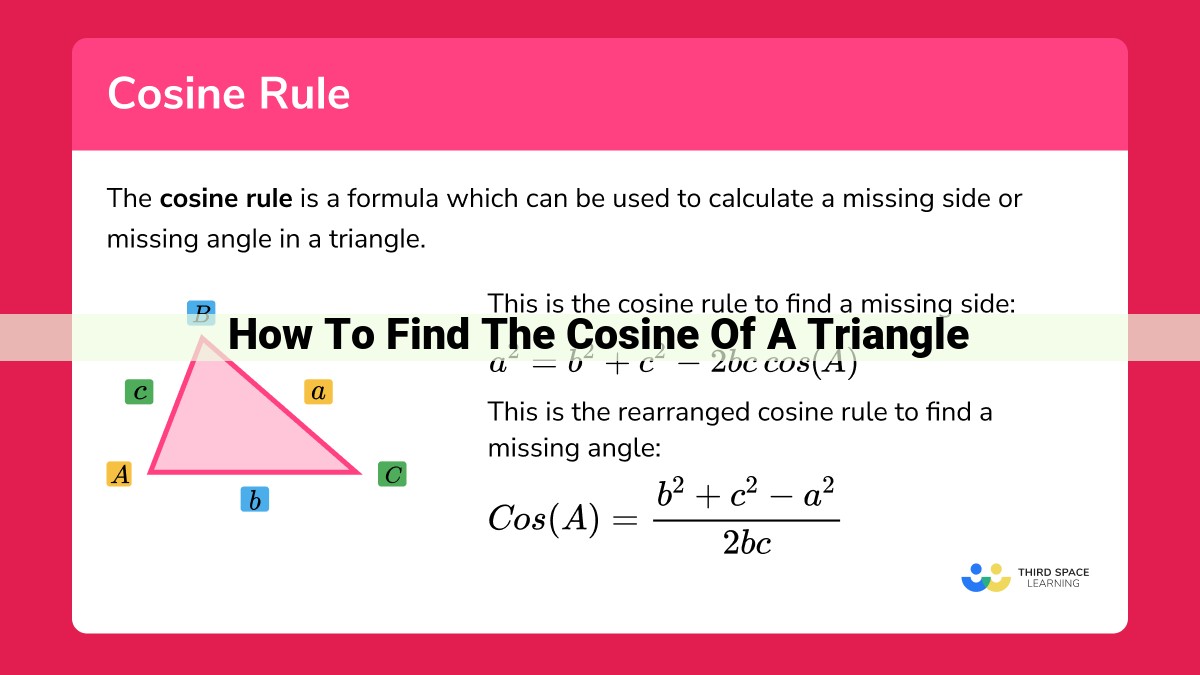
To find the cosine of a triangle, establish the triangle’s type (right-angled or general). For right-angled triangles, use the Pythagorean theorem to determine the lengths of its sides. For general triangles, employ the cosine theorem or law of cosines, which involves the lengths of its three sides. Additionally, trigonometric identities, the half-angle formula, and the angle bisector theorem offer alternative methods for finding the cosine. These concepts play a crucial role in solving triangle problems, determining angles and distances, and analyzing periodic functions, making the cosine a versatile function in trigonometry.
Cosine: The Key to Unlocking Triangles
In the realm of trigonometry, one indispensable function stands supreme: the cosine. Cosine represents the ratio of the adjacent side to the hypotenuse in a right-angled triangle, and its significance cannot be overstated. It serves as a cornerstone for understanding triangle relationships, solving complex trigonometric problems, and unlocking a myriad of real-world applications.
This comprehensive guide will equip you with the ultimate knowledge and techniques for finding the cosine of any triangle. Delve into the depths of trigonometry as we embark on a compelling journey through the Pythagorean theorem, Law of Cosines, angle bisector theorem, and a myriad of other essential concepts. Along the way, we will explore the fascinating connections between these concepts and their practical implications in our everyday lives.
Pythagorean Theorem: The Cornerstone of Cosine in Right-Angled Triangles
In the realm of trigonometry, the cosine function holds a pivotal position. It unveils the secrets of triangles, allowing us to decipher their hidden angles and lengths. When it comes to right-angled triangles, a special geometric formula plays a central role in our quest to find the cosine: The Pythagorean Theorem.
Imagine this: You’re standing on a grassy field, surrounded by three poles. Two of them form a right angle, like two perpendicular walls of a room. The third pole, like a curious wanderer, stands opposite the right angle. The Pythagorean Theorem tells us that the square of the distance between you and the tallest pole (the hypotenuse) is equal to the sum of the squares of the distances to the other two poles (the legs).
In the language of mathematics, this translates to:
a² + b² = c²
where a and b represent the lengths of the legs, and c is the hypotenuse.
How does this help us find the cosine?
Let’s say we know the lengths of the two legs, a and b. We want to find the cosine of the angle opposite the leg with length a. The cosine is defined as the ratio of the adjacent leg (in this case, b) to the hypotenuse (which we can find using the Pythagorean Theorem).
cos θ = b/c
So, to find the cosine, we can plug in our values for b and c from the Pythagorean Theorem:
cos θ = b/(√(a² + b²))
Example:
Let’s say we have a right-angled triangle with legs of length 3 and 4. Using the Pythagorean Theorem, we find that the hypotenuse is √(3² + 4²) = 5. Now, we can calculate the cosine of the angle opposite the leg of length 3:
cos θ = 4/5
Unlocking the Cosine: A Comprehensive Guide
In the realm of trigonometry, the cosine function reigns supreme, playing a pivotal role in unraveling the secrets of triangles. This blog post embarks on a journey to empower you with a comprehensive understanding of finding the cosine of a triangle. From the depths of the Pythagorean theorem to the intricacies of trigonometric identities, we will navigate the fascinating world of cosine, unveiling its significance and unlocking its applications.
The Pythagorean Theorem: A Gateway to Right Triangles
When it comes to right triangles, the Pythagorean theorem reigns supreme. It’s a legendary formula that connects the lengths of the triangle’s sides, allowing us to calculate the missing side. By understanding the Pythagorean theorem, you’ll gain the power to unlock the cosine of any right-angled triangle.
Cosine Theorem (Law of Cosines): A Guiding Light for Any Triangle
Beyond right triangles, the cosine theorem, also known as the Law of Cosines, unveils its power. This versatile formula transcends the limitations of right triangles, enabling us to find the cosine of any angle in any triangle. It’s a beacon of knowledge, guiding our path through even the most enigmatic triangles.
The cosine theorem is a majestic equation that connects the cosine of an angle to the lengths of the triangle’s sides. It provides a comprehensive framework for understanding the geometric relationships within any triangle, empowering us to unravel its mysteries.
Unveiling the Related Concepts: Law of Cosines and Dot Product
As we delve deeper into the cosine theorem, we encounter two intertwined concepts: the Law of Cosines and the dot product. These concepts complement the cosine theorem, providing additional perspectives and insights into its workings.
The Law of Cosines serves as a powerful tool in its own right, connecting the triangle’s sides and angles in a harmonious equation. It’s a versatile formula that complements the cosine theorem, offering a different perspective on the same underlying geometric principles.
Meanwhile, the dot product, a cousin of the cosine theorem, weaves its way into the realm of vector algebra. It measures the similarity between two vectors and, remarkably, its essence echoes within the cosine theorem. By understanding these related concepts, you’ll gain a deeper appreciation for the cosine theorem’s elegance and versatility.
Finding the Cosine of an Angle: A Comprehensive Guide
In the realm of trigonometry, the cosine function reigns supreme, providing a crucial link between the sides and angles of triangles. This comprehensive guide will unveil the secrets of finding the cosine of any triangle with ease.
Pythagorean Theorem
When dealing with right-angled triangles, the Pythagorean Theorem shines as a beacon of simplicity:
a² + b² = c²
where a and b are the lengths of the legs, and c is the length of the hypotenuse. Using this theorem, we can determine the cosine of an acute angle θ as:
cos θ = adjacent side / hypotenuse
Cosine Theorem (Law of Cosines)
Venturing beyond right-angled triangles, the Cosine Theorem (or Law of Cosines) emerges as a powerful tool:
c² = a² + b² - 2ab * cos θ
This formula allows us to calculate the cosine of any angle θ in a triangle, regardless of its type.
Half-Angle Formula
For those angles that are not easily halved, the Half-Angle Formula comes into play:
cos (θ/2) = ±√((1 + cos θ) / 2)
This formula helps us find the cosine of half an angle θ/2, given the cosine of the original angle θ.
Connections to the Angle Bisector Theorem and Double-Angle Formula further enrich our understanding of this formula.
Mastering the art of finding the cosine is a cornerstone of trigonometry. Through the Pythagorean Theorem, Cosine Theorem, Half-Angle Formula, and other techniques, we have equipped you with a comprehensive arsenal to solve triangle problems, determine angles and distances, and analyze periodic functions with confidence. May this guide serve as your constant companion in your trigonometrical adventures.
Trigonometric Identities: Unlocking the Power of Cosine
In the realm of trigonometry, cosine reigns supreme as a versatile trigonometric function. But finding its value can sometimes be a daunting task. Trigonometric identities come to our rescue, providing a lifeline for converting between different trigonometric functions and simplifying complex expressions.
The Magic of Identities
Trigonometric identities are like secret codes that allow us to transform one trigonometric function into another. They’re like translators that make it easier to communicate with the language of trigonometry. By using these identities, we can find the cosine of an angle even when it’s not immediately obvious.
Useful Identities for Finding Cosine
Several trigonometric identities are particularly handy for finding the cosine:
- Pythagorean Identity: sin²θ + cos²θ = 1
- Cosine of Complementary Angles: cos(90°-θ) = sinθ
- Cosine of Double Angles: cos(2θ) = cos²θ – sin²θ = 2cos²θ – 1
- Cosine of Half Angles: cos(θ/2) = ±√((1 + cosθ) / 2)
Harnessing the Power of Identities
Let’s say we want to find the cosine of 30 degrees. Using the Pythagorean Identity, we have:
cos²30° + sin²30° = 1
cos²30° = 1 - sin²30°
cos²30° = 1 - (1/2)²
cos²30° = 3/4
cos30° = √3/2
Similarly, we can use the Cosine of Half Angles Identity to find the cosine of 15 degrees:
cos(15°) = ±√((1 + cos30°) / 2)
cos(15°) = ±√((1 + √3/2) / 2)
cos(15°) = ±√((2 + √3) / 4)
cos(15°) = ±(√6 + √2) / 4
Trigonometric identities are invaluable tools for finding the cosine of an angle. They enable us to convert between different trigonometric functions, simplifying complex expressions and unlocking the full power of trigonometry. By embracing these identities, we can navigate the trigonometric landscape with ease and precision.
Angle Bisector Theorem: A Bridge to Cosine Exploration
In the realm of trigonometry, the angle bisector theorem emerges as a beacon guiding us toward a profound understanding of cosine. This theorem establishes an intricate connection between the cosine of an angle and its bisector.
The angle bisector theorem states that if a line bisects an angle of a triangle, the ratio of the lengths of the segments of the opposite side created by the bisector is equal to the ratio of the lengths of the adjacent sides. In mathematical terms:
AC/CB = AB/BC
where AC and CB are the lengths of the segments of the opposite side, and AB and BC are the lengths of the adjacent sides.
This theorem serves as a stepping stone in our quest to comprehend the cosine. By employing the angle bisector theorem, we can derive the half-angle formula, which allows us to determine the cosine of half an angle.
The half-angle formula states that:
cos(θ/2) = ±√((1 + cos θ) / 2)
where θ is the original angle. This formula provides a powerful tool for unraveling trigonometric relationships, as it reveals the cosine of an angle in terms of the cosine of its half.
Furthermore, the angle bisector theorem is intertwined with the perpendicular bisector theorem. This theorem asserts that a line perpendicular to a line segment at its midpoint bisects the line segment. By combining these theorems, we can establish deeper connections between the cosine of an angle and the lengths of the sides of the triangle.
In summary, the angle bisector theorem acts as a conduit, linking the cosine of an angle to the lengths of the sides of its triangle. This theorem, coupled with the half-angle formula, empowers us to delve into a comprehensive understanding of cosine and its multifaceted applications in trigonometry.
Applications in Trigonometry:
- Discuss practical applications of finding the cosine in trigonometry, such as:
- Solving triangle problems
- Determining angles and distances
- Analyzing periodic functions
Applications of Cosine in Trigonometry
In the realm of trigonometry, the cosine function plays a crucial role in solving triangle problems and determining angles and distances. Its applications extend beyond theoretical calculations into practical scenarios that impact various fields.
Solving Triangle Problems
Whether you’re an architect designing a building or a surveyor mapping a plot of land, the ability to solve triangle problems is essential. The cosine law, also known as the law of cosines, provides a powerful tool for finding missing sides or angles in oblique triangles, where no side is perpendicular to another. By applying the cosine law, you can uncover the hidden dimensions and relationships within triangles.
Determining Angles and Distances
In navigation, astronomy, and many other disciplines, accurately measuring angles and distances is paramount. The cosine law enables us to solve for angles in non-right triangles, a valuable skill when using triangulation techniques for determining the position of objects or the distance to celestial bodies.
Analyzing Periodic Functions
Cosine plays a vital role in the analysis of periodic functions, such as waves and oscillations. The trigonometric cosine function describes the vertical displacement of a point moving in a circular motion, giving rise to the familiar sinusoidal form that underlies many natural and engineering phenomena. By understanding how the cosine varies over time, we can gain insights into periodic systems and make predictions about their behavior.
The cosine function is an indispensable tool for understanding and solving problems in trigonometry. It extends beyond classroom calculations to find practical applications in engineering, navigation, and the analysis of periodic functions. Whether you’re a student eager to master the art of trigonometry or a professional seeking to apply it in the real world, understanding the cosine is essential for success.