Converting Polar To Rectangular Equations For Enhanced Geometric Precision
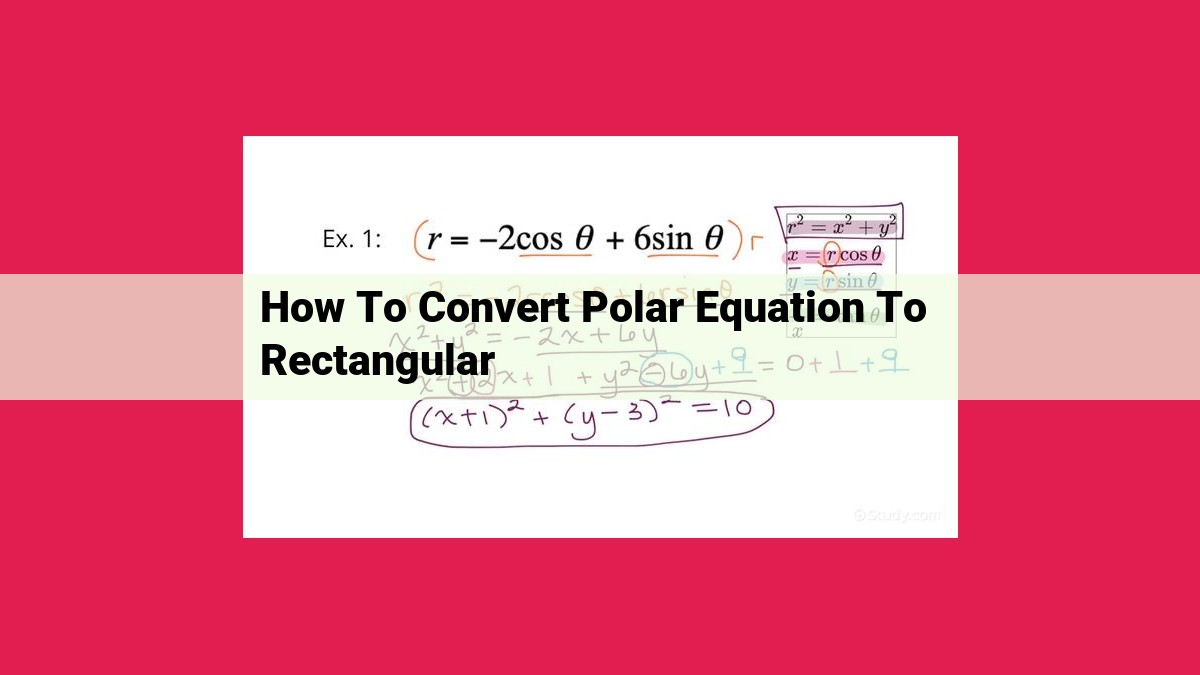
To convert polar equations (distance and angle) to rectangular equations (x and y), use the formulas: x = r cos(θ) and y = r sin(θ). Determine the quadrant of the point using the signs of x and y. Find the reference angle (acute angle with positive x-axis) using inverse trigonometric functions. Convert the polar angle to a quadrantal angle based on the quadrant and reference angle. Finally, derive the rectangular equation by substituting the quadrantal angle and distance (r) into the conversion formulas.
Navigating the Coordinate Maze: Unveiling Polar and Rectangular Coordinates
In the realm of mathematics, where numbers dance and shapes take form, we encounter two fundamental coordinate systems: polar coordinates and rectangular coordinates. These systems provide a language for describing locations in a plane, empowering us to map the world around us.
Polar Coordinates: A Radial Perspective
Imagine yourself at the center of a circle. Any point on the circle’s circumference can be pinpointed by two attributes: its distance from the center (called the radius) and the angle it forms with the positive x-axis (known as the polar angle). This duo of parameters defines polar coordinates.
Rectangular Coordinates: A Cartesian Grid
In contrast, rectangular coordinates employ two perpendicular lines called the x-axis and y-axis, creating a grid known as the Cartesian plane. Every point on the plane is specified by its horizontal distance from the y-axis (the x-coordinate) and its vertical distance from the x-axis (the y-coordinate).
Bridging the Coordinate Divide: Conversion from Polar to Rectangular
As we navigate these coordinate systems, we often encounter the need to convert from polar to rectangular coordinates. This conversion involves a few key equations:
- x = r cos(θ): The x-coordinate is the radius multiplied by the cosine of the polar angle.
- y = r sin(θ): The y-coordinate is the radius multiplied by the sine of the polar angle.
Inverse Trigonometric Functions: Angles in Disguise
To complete the conversion, we sometimes need to determine the polar angle from trigonometric ratios. Here, inverse trigonometric functions come to our aid:
- arcsine (sin⁻¹): Given the sine ratio, it finds the angle.
- arccosine (cos⁻¹): Given the cosine ratio, it finds the angle.
- arctangent (tan⁻¹): Given the tangent ratio, it finds the angle.
Quadrant Quandary: Determining the Point’s Domain
To pinpoint the point’s location accurately, we need to determine the quadrant in which it resides. This is done by examining the signs of the x- and y-coordinates:
- Quadrant I: (+) x, (+) y
- Quadrant II: (-) x, (+) y
- Quadrant III: (-) x, (-) y
- Quadrant IV: (+) x, (-) y
Reference Angle: The Guiding Light
In certain cases, we may need to find the reference angle. This is the positive acute angle between the terminal arm (the line connecting the origin to the point) and the nearest coordinate axis. It helps us determine the quadrant and simplify calculations.
The Conversion Saga: A Summary
In summary, converting polar equations to rectangular equations entails the following steps:
- Use the given equations to find x and y coordinates.
- Determine the quadrant of the point.
- Calculate the reference angle if necessary.
- Use inverse trigonometric functions to find the polar angle if needed.
By mastering these techniques, we unlock the power of these coordinate systems, enabling us to unravel the mysteries of the mathematical world and navigate the coordinates of our surroundings.
Mastering the Art of Polar to Rectangular Coordinates: Demystifying the Conversion
Embark on an exciting adventure into the world of coordinates, where we’ll unravel the secrets of converting polar coordinates into their rectangular counterparts. Polar coordinates, represented by the familiar duo of distance (r) and angle (θ), describe a point’s location on a plane from the origin. Rectangular coordinates, on the other hand, use the trusty x and y axes to pinpoint a point’s position.
To transform polar coordinates into rectangular coordinates, we rely on a magical formula that unlocks the conversion:
x = r * cos(θ)
y = r * sin(θ)
These equations translate the distance (r) and angle (θ) into their rectangular equivalents (x and y). Imagine r as the radius of a circle, and θ as the angle formed between the radius and the positive x-axis. The coordinates x and y represent the projection of the point onto the x and y axes, respectively.
Understanding the concept of reference angle is crucial in this conversion process. It’s the positive acute angle formed between the terminal arm of the angle θ and the positive x-axis. To determine the reference angle, we use inverse trigonometric functions, such as arcsine, arccosine, and arctangent, which help us find the angle given a trigonometric ratio.
To determine the quadrant in which our rectangular coordinate resides, we pay attention to the signs of x and y. Positive x values indicate the first or fourth quadrant, negative x values suggest the second or third, while positive y values mark the first or second quadrant, and negative y values point towards the third or fourth.
In essence, converting polar coordinates to rectangular coordinates involves a series of steps:
- Determine the reference angle using inverse trigonometric functions.
- Identify the quadrant based on the signs of x and y.
- Apply the formulas: x = r * cos(θ) and y = r * sin(θ).
These steps empower us to seamlessly navigate between the polar and rectangular coordinate systems, unlocking a deeper comprehension of geometry, trigonometry, and their applications in various fields.
Understanding Inverse Trigonometric Functions for Coordinate Conversion
In the realm of mathematics, where numbers dance and equations intertwine, we embark on a journey to unravel inverse trigonometric functions—tools that bridge the gap between angles and trigonometric ratios. These indispensable functions unlock the secrets of converting polar coordinates to rectangular coordinates, enabling us to paint a clearer picture of the world around us.
Introducing the Inverse Trigonometric Trio:
At the heart of this conversion process lie three pivotal functions:
- Arcsine (sin⁻¹): Reveals the angle whose sine equals a given value.
- Arccosine (cos⁻¹): Uncovers the angle whose cosine matches a specified ratio.
- Arctangent (tan⁻¹): Unmasks the angle whose tangent corresponds to a predetermined value.
The Concept of Reference Angle:
When dealing with angles, it’s crucial to recognize the reference angle—the positive acute angle formed between the terminal arm (pointing to our target angle) and the coordinate axis. This fundamental concept forms the cornerstone of our conversion endeavors.
Visualizing the Quadrants:
Before delving into our conversion wizardry, let’s establish a mental framework—the Cartesian plane and its four quadrants. Each quadrant is defined by the signs of its x and y coordinates, providing a roadmap for locating points.
Determining the Quadrant
In the world of coordinates, the Cartesian plane reigns supreme. This plane is like a grand chessboard with two perpendicular axes: the x-axis (horizontal line) and the y-axis (vertical line). The point where these axes meet is the origin, like the center of the chessboard.
Imagine you have a point on this chessboard, like a knight on square E4. To determine which quadrant this knight resides in, you simply need to check the signs of its x-coordinate (the number before the letter) and its y-coordinate (the number after the letter).
Like the four seasons, the Cartesian plane is divided into four quadrants, each with its unique personality:
- Quadrant I (The Northeastern Knight): Both x and y are positive, a happy knight basking in the sunlight.
- Quadrant II (The Northwestern Knight): x is negative, while y is positive, a knight facing west but still basking in the sunlight.
- Quadrant III (The Southwestern Knight): Both x and y are negative, a knight in a gloomy corner.
- Quadrant IV (The Southeastern Knight): x is positive and y is negative, a knight facing east but cast in shadow.
Remember, these rules are as steadfast as the magnetic compasses guiding ships across the seas. By unraveling the signs of the x and y coordinates, you can pinpoint the quadrant of any point with ease, just like a seasoned sailor navigating the vast Cartesian ocean.
Calculating the Reference Angle: Unlocking the Secrets of Polar Coordinates
In the realm of mathematics, where shapes and numbers intertwine, there exists a fascinating conversion process between two coordinate systems: polar and rectangular. To delve into the depths of this conversion, we must first master the art of calculating the reference angle.
Picture yourself standing at the origin of a coordinate plane, with a compass in hand. Imagine a ray extending from the origin, like an arrow pointing in a specific direction. The angle between this ray and the positive x-axis is known as the polar angle.
Now, let’s introduce another concept: the reference angle. This is the acute angle formed between the ray and the positive x-axis, measured clockwise from the x-axis. To determine the reference angle:
- Determine the quadrant of the point where the ray intersects the coordinate plane.
- Subtract the polar angle from 360° if the point lies in the second or third quadrant.
This simple calculation gives us the reference angle, which allows us to unlock the secrets of converting polar to rectangular coordinates.
Distinguishing Polar Angle from Reference Angle
It’s crucial to differentiate between the polar angle and the reference angle. The polar angle is a measure of the direction of the ray from the origin, while the reference angle is the acute angle formed with the positive x-axis. Understanding this distinction is key to successfully converting between polar and rectangular coordinates.
Remember, this conversion process is like a bridge between two worlds, enabling us to navigate the complexities of different coordinate systems with ease.