Discover The Total Area Of Composite Figures: A Comprehensive Guide

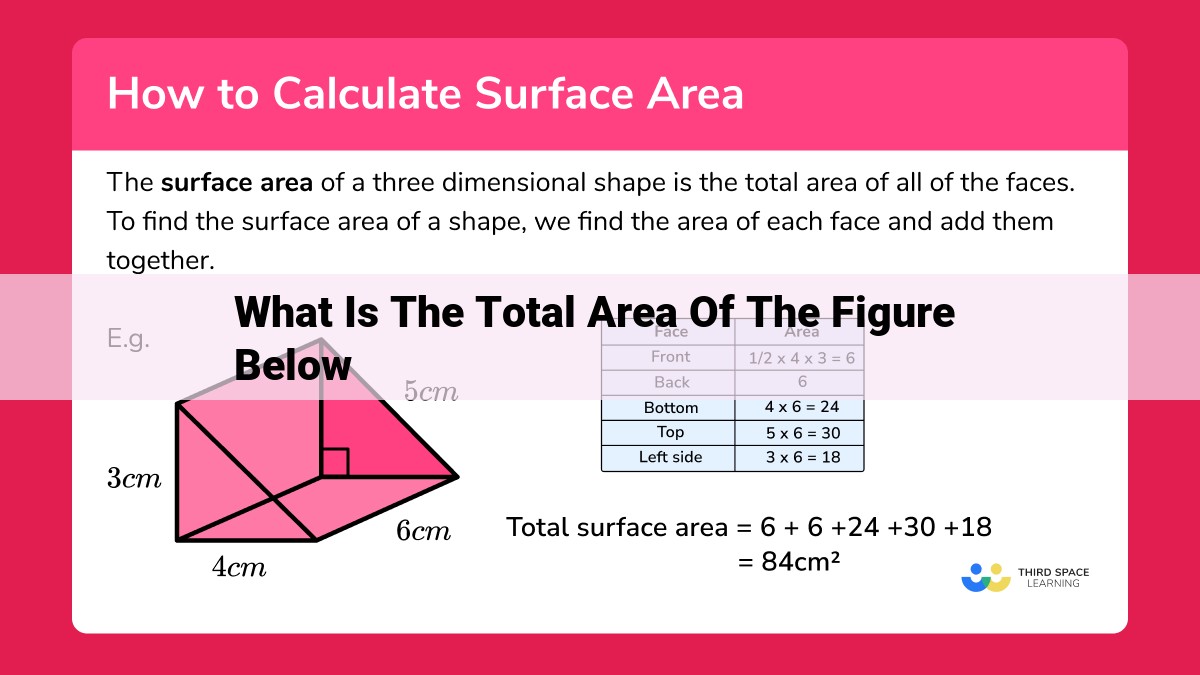
To find the total area of the given figure, first identify the individual shapes that compose it. Calculate the area of each shape using relevant formulas, such as A = L x W for rectangles or (1/2) x base x height for triangles. If applicable, use the Pythagorean theorem to determine the height of triangles. If the shapes overlap, subtract the overlapping area to avoid double counting. Finally, add the areas of all the individual shapes together to obtain the total area of the composite figure.

In the realm of geometry, the concept of area reigns supreme as a fundamental measure of surface. It quantifies the extent of a two-dimensional shape, revealing its size and dimensions. From the smallest postage stamp to the vast expanse of a continent, area plays a pivotal role in our comprehension of the world around us.

Area bears a profound relationship with length and width. Imagine a rectangular garden stretching out before you. Its length dictates how far the garden extends from one end to the other, while its width determines its breadth. The area of this garden, calculated by multiplying the length and width, provides an accurate representation of the space it occupies.

Calculating Area: Exploring Geometrical Dimensions

In the realm of geometry, area reigns supreme as a measure of surface. Comprehending its significance unlocks the door to a plethora of real-world applications.

Area of a Rectangle: A Simple Formula

A rectangle, with its straight sides and right angles, epitomizes simplicity. Its area, the amount of two-dimensional space it occupies, is effortlessly determined by multiplying its length (L) by its width (W).

For instance, a rectangle with a length of 5 units and a width of 3 units possesses an area of 15 square units.

Area of a Triangle: Beyond the Rectangle

Venturing beyond rectangles, we encounter triangles, shapes with three sides and three angles. Unlike rectangles, triangles require a different approach to calculating their area. The formula hinges on two key elements: the base (b) and the height (h).

The base represents the triangle’s side that rests flat on the ground. The height, perpendicular to the base, extends from the base to the opposite vertex.

Calculating the height of a triangle can involve the Pythagorean theorem, a mathematical tool that relates the lengths of the sides of a right triangle. Once the height is known, the area of the triangle is found by multiplying half the base by the height.

Sum of Areas: Combining Shapes

The world around us often presents us with composite figures, shapes composed of multiple simpler shapes. Determining the total area of such figures requires a nuanced understanding of overlapping and disjoint areas.

Overlapping areas occur when two shapes share a common space. To calculate the combined area, subtract the area of the overlap from the sum of the individual areas.

Disjoint areas, on the other hand, stand apart without any overlap. In such cases, the combined area is simply the sum of the individual areas.

Applying Area Concepts in the Real World

In the realm of architecture, area calculations are indispensable for designing buildings that suit our needs. Architects meticulously determine the area of rooms, hallways, and outdoor spaces to optimize functionality and ensure comfortable living conditions. From sprawling mansions to compact apartments, area measurements guide every design decision.

Land surveying relies heavily on area calculations to accurately determine the size and boundaries of properties. Surveyors use this information to create detailed maps, establish property lines, and resolve land disputes. Their precise area calculations ensure that every inch of land is accounted for, safeguarding landowners’ rights.

In the world of interior design, area calculations are crucial for planning and optimizing space. Designers calculate the area of rooms and furniture to create harmonious and functional layouts. They determine the appropriate size of rugs, sofas, and other furnishings to maximize comfort and aesthetic appeal. By carefully considering area measurements, designers transform living spaces into inviting and stylish havens.

Common Area Measurement Units and Conversions

When working with area measurements, it’s essential to understand the commonly used units. The square foot (sq ft) and square meter (sq m) are the most prevalent units for measuring area.

To convert between units, simply multiply by the appropriate conversion factor:

• 1 sq m = 10.764 sq ft
• 1 sq ft = 0.0929 sq m

By understanding these conversion techniques, you can seamlessly navigate different area measurements and ensure accuracy in your calculations.

Solving Area Problems: Strategies and Examples

In the realm of geometry, area calculations play a vital role in determining the surface coverage of various shapes. From architects designing homes to land surveyors marking boundaries, the concept of area finds applications in diverse fields. To master the art of solving area problems, let’s delve into some effective strategies and explore practical examples.

Using Formulas and Dividing Figures

At the heart of area calculations lie formulas, tailor-made for specific shapes. For instance, to find the area of a rectangle, simply multiply its length by its width. For a triangle, the height and base measurements come into play. If you encounter a composite figure, break it down into simpler shapes and calculate their areas individually.

Practice Makes Perfect

To solidify your understanding, let’s tackle some practice examples. Imagine you need to determine the area of a rectangular garden measuring 10 feet long and 6 feet wide. Simply multiply these values, 10 x 6, to get the total area: 60 square feet.

Now, let’s consider a triangular plot with a base of 8 meters and a height of 5 meters. Recall the formula for a triangle’s area: 1/2 x base x height. Plugging in the values, we get: 1/2 x 8 x 5 = 20 square meters.

Bringing It All Together

The key to solving area problems lies in understanding the relevant formulas and applying them accurately. By dissecting composite shapes into simpler ones, you can extend these formulas to calculate the total area. With practice, you’ll develop a solid foundation in area calculations, equipping you for success in various endeavors.