Mastering Composite Figure Area Calculations For Practical Applications

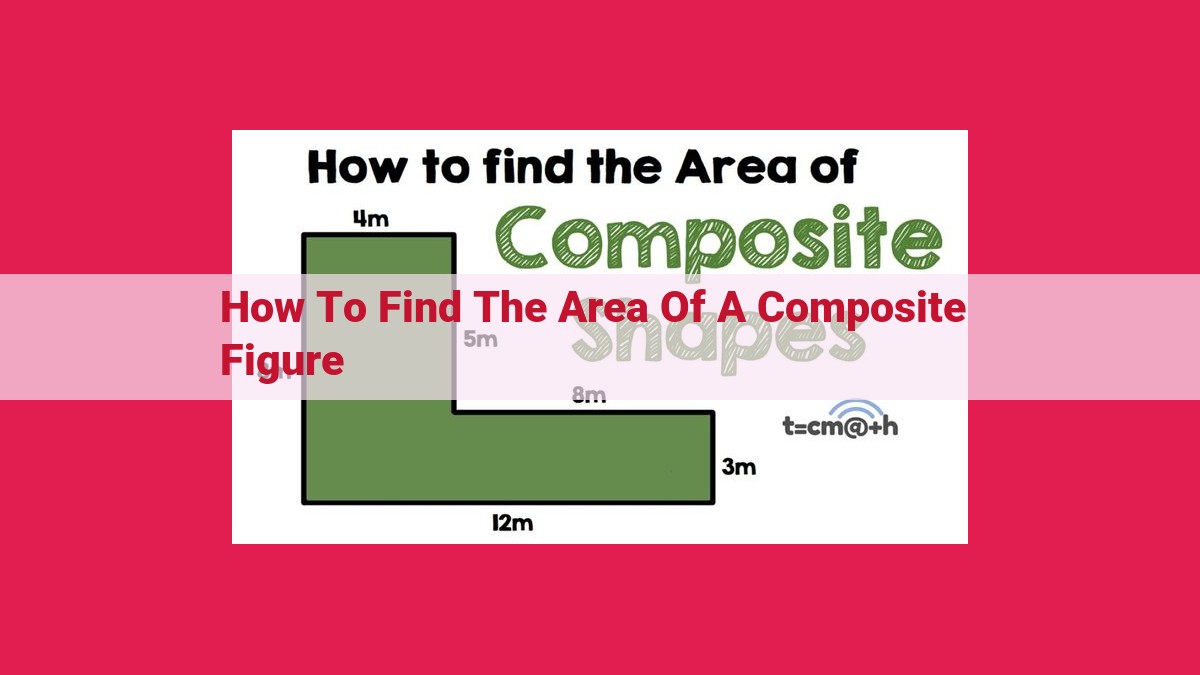
To find the area of composite figures, divide the figure into smaller, non-overlapping shapes. Calculate the area of each individual shape using relevant formulas, then combine their areas to get the total area. Alternatively, use geometry software to draw and measure the composite figure directly. Understanding these strategies is crucial for various disciplines that involve geometric shapes.

• Define composite figures and their significance.
• State the purpose of the guide: to provide comprehensive strategies for finding the area of composite figures.

Finding the Area of Composite Figures: A Comprehensive Guide

In the fascinating world of geometry, we often encounter figures that are not as straightforward as simple shapes like squares or circles. Composite figures are those that are made up of two or more smaller figures combined together. Understanding the area of these composite figures is crucial in various disciplines such as architecture, engineering, and design.

This guide will provide you with a comprehensive set of strategies to master the art of finding the area of composite figures. Whether you’re a student navigating the complexities of geometry or a professional seeking to optimize your spatial understanding, this guide has got you covered.

What are Composite Figures?

Composite figures are essentially shapes that are formed by combining multiple smaller shapes. These individual shapes can be anything from triangles and rectangles to circles and even more complex polygonal shapes. The key characteristic of composite figures is that they are composed of non-overlapping shapes, making it possible to dissect them into smaller units.

Why is it Important to Find the Area of Composite Figures?

The area of a composite figure represents the total surface enclosed within its boundaries. This measurement is critical for a wide range of applications, such as:

• Architecture: Determining the area of a room or building helps in planning efficient space utilization and estimating material requirements.
• Engineering: Engineers need to calculate the area of complex structures to ensure stability, functionality, and optimal resource allocation.
• Design: In fields like interior design and landscaping, finding the area of composite shapes is essential for creating visually appealing and practical spaces.

Unraveling the Enigma of Composite Figures: Types and Decoding Their Composition

Venture into the intriguing realm of composite figures, where shapes dance in harmonious union to form captivating masterpieces. These complex creations, unlike their simpler counterparts, are composed of a medley of smaller shapes, each with its own distinct identity.

Delving into the diverse world of composite figures, we encounter a kaleidoscope of shapes that seamlessly intertwine to create harmonious wholes. Quadrilaterals, with their four straight sides, form the foundation of many composite figures. Their versatility allows them to morph into rectangles, squares, parallelograms, and trapezoids, each with its unique set of properties.

Triangles, with their inherent stability, are another fundamental building block. They grace composite figures with their equilateral, isosceles, or scalene configurations. Circles, the embodiment of perfect curvature, lend an air of elegance to composite figures, adding a touch of organic beauty to their geometric tapestry.

But what truly sets composite figures apart is their boundless creativity. They defy categorization, blending various shapes in countless combinations. Intricate patterns emerge, where triangles nestle within circles, and quadrilaterals dance around curves. Each composite figure tells a captivating tale of geometric harmony, inviting us to unravel its secrets.

Strategy 1: Deconstructing Composite Figures into Smaller Shapes

Imagine you’re faced with a puzzle—a complex shape that seems daunting to measure. But what if you could break it down into smaller, more manageable pieces? That’s precisely the essence of Strategy 1.

This strategy involves carefully dividing the composite figure into non-overlapping smaller shapes. Like a master puzzle-solver, you identify the individual pieces that make up the whole. Recognizing these individual shapes is crucial, as they hold the key to unlocking the figure’s total area.

Think of it as a game of Tetris: You need to fit each shape perfectly into its allotted space. By skillfully dividing the composite figure, you create a collection of smaller, simpler shapes. Now, you’re ready to conquer each shape individually, paving the way for determining the total area of the composite figure.

Strategy 2: Harmonizing the Areas of Individual Shapes

In the realm of composite figures, mastering the art of decomposing them into their constituent shapes is just half the journey. The next crucial step is combining the areas of these individual shapes to unveil the overall area of the composite figure.

Just like a mosaic composed of countless tiles, each individual shape within a composite figure holds its own unique area. To calculate this area, we employ relevant formulas tailored to each shape. For instance, rectangles and squares surrender their area when we multiply their length by their width. Triangles unveil their area when we embrace half the product of their base and height. And circles, those enigmatic shapes, reveal their area when we seek the camaraderie of pi and their radius.

Once we have lovingly coaxed the area of each individual shape from their geometric depths, the final chapter of our adventure unfolds. We add these individual areas together, harmonizing them into a grand total, which represents the total area of our composite figure. It’s like a puzzle coming together, each piece contributing to the majestic whole.

By embracing this strategy, we become architects of our own geometric understanding, equipped to unravel the mysteries of composite figures with ease and precision.

Strategy 3: Using Formulas for Specific Shapes

In this realm of composite shapes, there comes a point where you need to calculate the area of each individual shape lurking within the intricate whole. Here, let’s journey into the world of formulas, our trusty companions for unlocking these geometric secrets.

For a triangle, its area is half its base multiplied by its height. Imagine a triangle as a slice of cake. Its base is the straight edge, like the bottom of the cake, and its height is the distance from the base to the peak, like the frosting on top. So, if your triangle’s base is 5 cm and its height is 3 cm, its area would be 7.5 square cm.

Next, let’s conquer rectangles. These are like perfect squares turned on their side. Their area is length multiplied by width. Think of a rectangle as a napkin. Its length is the longer side, and its width is the shorter side. If your napkin measures 10 cm by 5 cm, its area is 50 square cm.

Now, let’s tackle the circle, a shape as round as the moon. Its area is calculated using the formula πr², where π is a constant equal to approximately 3.14, and r is the radius of the circle. Imagine a pizza. Its radius is the distance from the center to the edge, and its area is the total amount of pizza you can devour.

When you encounter a composite shape, simply identify the individual shapes within it and apply the appropriate formulas. For instance, if you have a shape that’s a combination of a triangle and a rectangle, you’d calculate the area of the triangle and the rectangle separately and then add them together to get the total area.

So, there you have it, the power of formulas for unlocking the areas of composite shapes. May your geometric adventures be filled with precision and triumphant calculations!

Using Technology to Simplify Area Calculations: Geometry Software for Composite Figures

When dealing with complex shapes, finding areas can become challenging. However, geometry software emerges as a powerful tool that can simplify this process, making it more manageable and accurate.

Imagine yourself as an architect tasked with calculating the floor space of a building with intricate layouts. Instead of manually measuring each room and adding them up, geometry software allows you to draw the floor plan digitally, dividing it into individual shapes. With the help of pre-defined formulas embedded in the software, it can effortlessly calculate the area of each shape and sum them up to give you the total area of the building.

Not only does geometry software streamline the process, but it also minimizes errors that may arise from manual calculations. Its precision ensures that you obtain accurate results, allowing you to focus on other aspects of your project with confidence. The software’s ability to create scale drawings also enables you to visualize the layout, making it easier to plan and design your project.

Moreover, geometry software offers a wide range of features that can enhance your workflow. You can easily edit and modify shapes, allowing you to explore different design options effortlessly. The software also provides measurements and calculations for angles, distances, and perimeters, giving you a comprehensive understanding of your project’s geometry.

In conclusion, geometry software has revolutionized the way we measure and analyze shapes. Its user-friendly interface and powerful tools make it an invaluable asset for professionals in architecture, engineering, design, and beyond. By leveraging geometry software, you can streamline your workflow, ensure accuracy, and explore design options with ease.