Charging Capacitors: A Comprehensive Guide To Voltage, Current, And Time

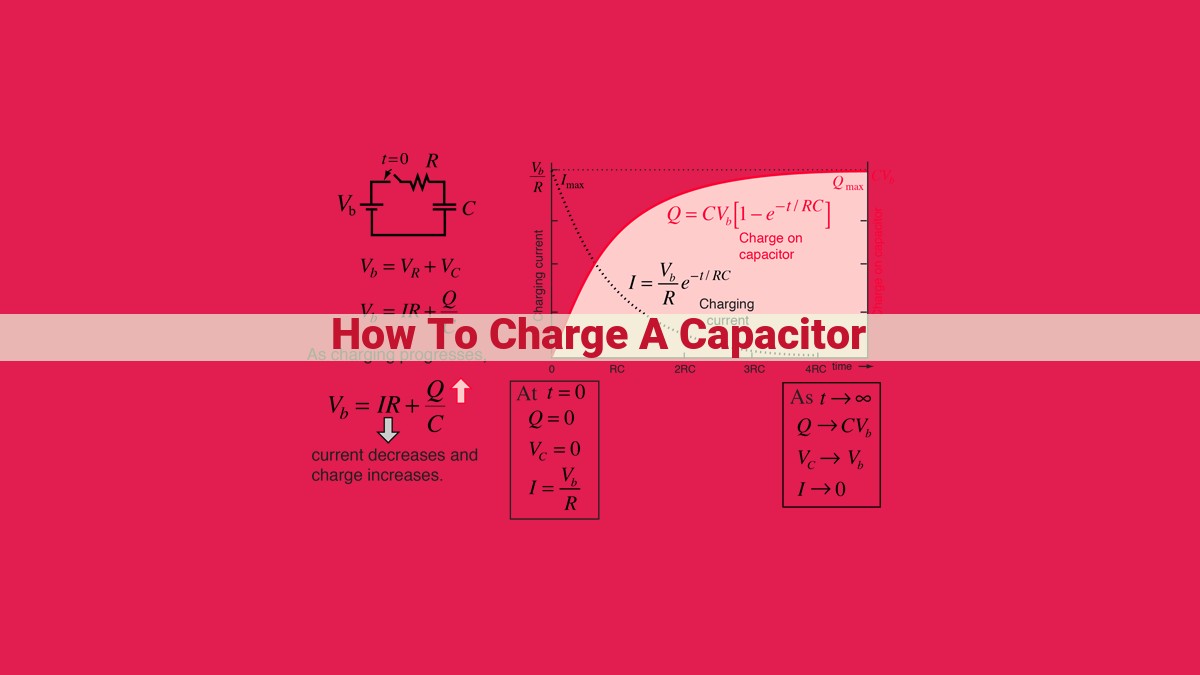
To charge a capacitor, connect it to a voltage source. The charging current flows into the capacitor, positively charging one plate and negatively charging the other. The voltage across the capacitor increases exponentially over time, following the equation Vc = V * (1 – e^(-t/RC)). Here, V is the voltage source, R is the resistance in the circuit, C is the capacitance, and t is time. The time constant (RC) represents the time taken for the capacitor to reach 63.2% of its maximum charge. As the voltage across the capacitor approaches the voltage source, the charging current decreases. Capacitance determines the amount of charge stored for a given voltage. The charging current is inversely proportional to the resistance and directly proportional to the time constant.

Understanding Charging a Capacitor

Voltage, Capacitance, and Charging

In the realm of electronics, capacitors reign supreme in storing electrical energy. They’re simple yet powerful devices that consist of two conductive plates separated by a non-conductive material. When you connect a capacitor to a voltage source, it embarks on a journey of charging.

Key Terms:

• Voltage: The electrical force that drives current through a circuit. Measured in volts.
• Capacitance: The ability of a capacitor to store electrical energy. Measured in farads.
• Charging Current: The flow of electrons from the voltage source that charges the capacitor.

The Role of Time Constant

The charging process is characterized by a time constant, tau. This value, measured in seconds, determines how quickly a capacitor charges or discharges. A smaller time constant means faster charging.

Charging Equation

The voltage across a charging capacitor follows an exponential curve, described by the charging equation:
Vc = V_max * (1 – e^(-t / tau))
– Vc is the voltage across the capacitor at time t.
– V_max is the maximum voltage of the voltage source.
– e is the mathematical constant approximately equal to 2.71828.
– t is the time elapsed since the start of charging.

The Process of Charging a Capacitor

• Explain the flow of charging current and the role of voltage.

The Intriguing Dance of Charging a Capacitor

As a capacitor embarks on its journey of accumulating charge, an enchanting dance unfolds. Imagine a tiny stream of electrons, eager to fill the capacitor’s plates. This surge of charging current marks the commencement of the capacitor’s transformation from an empty vessel to a reservoir of electrical energy.

The role of voltage in this dance is paramount. It acts as the driving force, propelling the electrons towards the capacitor’s plates. As the electrons accumulate, the capacitor’s plates acquire a potential difference, or voltage. Like a pendulum, the charging current oscillates, gradually diminishing as the voltage approaches its maximum value.

During this captivating process, the electrons form an orderly queue, their flow precisely governed by the capacitor’s capacitance. This capacitance dictates the capacitor’s ability to store electrical energy. The higher the capacitance, the greater the storage capacity.

With each passing moment, the capacitor’s plates grow increasingly charged, their attraction for electrons diminishing. This leads to a gradual decrease in the charging current, as fewer electrons are needed to maintain the voltage.

Finally, the charging current dwindles to zero, signaling that the capacitor has reached its maximum voltage. The dance of charging concludes, leaving the capacitor poised to release its stored electrical energy upon discharge.

Voltage During Charging

As the capacitor charges, its voltage steadily increases. This increase in voltage is governed by the charging equation, which describes the relationship between voltage, time, and the circuit’s resistance and capacitance.

The charging equation is:

$$V(t) = V_{max} (1 – e^{-t/RC})$$

where:

• V(t) is the voltage across the capacitor at time t
• V_{max} is the maximum voltage applied to the capacitor
• t is the time since charging began
• R is the resistance in the circuit
• C is the capacitance of the capacitor

This equation reveals that the voltage across the capacitor increases exponentially over time. Initially, the voltage rises rapidly, but as t increases, the rate of increase slows down. Eventually, the voltage approaches V_{max} asymptotically.

The time constant RC plays a crucial role in determining the rate of charging. A larger time constant means a slower rate of charging, while a smaller time constant results in a faster charging rate.

Capacitance and Charging: A Story of Electrical Storage

In the realm of electricity, capacitors stand out as masters of charge storage. Imagine a capacitor as a tiny vault where electrical charge resides, waiting to be released when needed. Just like a bank account holds money, a capacitor stores electrical charge.

The ability of a capacitor to hold charge is measured by its capacitance. The higher the capacitance, the more charge it can store. Think of it as the size of the vault; the bigger the vault, the more money it can hold.

Now, let’s talk about charging current. When a capacitor is connected to a power source, like a battery, a flow of electrons, known as charging current, rushes into the capacitor. The charging current determines how quickly the capacitor fills up with charge. It’s like a water faucet filling a glass; a faster-flowing faucet fills the glass quicker.

Finally, time constant comes into play. It’s the time it takes for the capacitor to reach approximately 63% of its maximum charge. Think of it as the time it takes for the glass to fill up most of the way. A shorter time constant means the capacitor charges up faster.

These three factors – capacitance, charging current, and time constant – work together to govern the charging process of a capacitor. It’s like a balancing act; adjusting one factor affects the others, ultimately determining how much charge the capacitor can store and at what rate it charges. Understanding this dynamic is essential for harnessing the power of capacitors in electrical circuits.

**Charging Current and Time Constant: Unlocking the Mysteries of Capacitor Dynamics**

In the realm of electrical circuits, understanding the behavior of capacitors is crucial. When a capacitor is connected to a voltage source, it embarks on a fascinating journey, storing charge and releasing it. Charging current plays a pivotal role in this process, and its relationship with time constant provides valuable insights into the dynamics of capacitor charging.

Defining Charging Current

Charging current is the current that flows through a capacitor as it accumulates charge. It represents the rate at which charge is being transferred from the voltage source to the capacitor. As the capacitor accumulates more charge, the charging current gradually decreases, ultimately reaching zero when the capacitor is fully charged.

Time Constant: A Crucial Indicator

The time constant is an important concept in capacitor charging. It represents the amount of time it takes for the capacitor to reach approximately 63.2% of its final charge. The time constant is directly proportional to both the capacitance of the capacitor and the resistance of the circuit it’s connected to. A larger capacitance or a higher resistance results in a longer time constant.

Interplay of Charging Current and Time Constant

The charging current is inversely proportional to the time constant. As the time constant increases, the charging current decreases. This relationship highlights that a capacitor with a small time constant rapidly accumulates charge, while a capacitor with a large time constant takes more time to reach its final charge.

Practical Implications

Understanding charging current and time constant is essential for designing and analyzing circuits involving capacitors. For example, in a camera flash, a capacitor is charged quickly using a high charging current to ensure a bright flash. Conversely, in a timing circuit, a capacitor is charged slowly using a low charging current to achieve accurate time delays.

The charging current and time constant are two key parameters that govern the dynamics of capacitor charging. By comprehending their relationship, we gain valuable insights into the behavior of capacitors and can effectively harness their power in various electrical applications.

Discharging a Capacitor: The Art of Releasing Stored Energy

In the realm of electronics, capacitors play a crucial role in storing and releasing electrical energy. When a capacitor is charged, it accumulates electrons on one plate and an equal number of positive charges on the other. But what happens when we want to release this stored energy? That’s where discharging comes in.

The Process of Discharge

Discharging a capacitor is the process of removing the accumulated charge from its plates. Unlike charging, which requires an external source of voltage, discharging occurs naturally when the capacitor is connected to a closed circuit. The direction of the current flow reverses, and electrons flow from the negatively charged plate to the positively charged plate.

The Role of Resistance

The rate at which a capacitor discharges is determined by the resistance in the circuit. Resistance acts as a barrier to the flow of current, slowing down the process of electron transfer. The higher the resistance, the slower the discharge rate. In a circuit with low resistance, the capacitor discharges rapidly, while in a circuit with high resistance, it discharges more gradually.

Mathematical Insights

The discharge of a capacitor can be described mathematically by the equation:

V(t) = V0 * e^(-t/RC)

where:

• V(t) is the voltage across the capacitor at time t
• V0 is the initial voltage across the capacitor
• R is the resistance in the circuit
• C is the capacitance of the capacitor

This equation shows that the voltage across the capacitor decays exponentially over time. The time constant of the circuit, represented by the term RC, determines how quickly the voltage decays. A shorter time constant indicates a faster discharge rate.

Applications of Discharge

Discharging capacitors finds numerous applications in electronic circuits. For instance, in timing circuits, discharging capacitors provides a precise time delay. In flash photography, discharging a large capacitor generates a burst of high-intensity light. Additionally, discharging capacitors can be used to power electronic devices, such as defibrillators and pacemakers.

Understanding the process of discharging a capacitor is essential for designing and analyzing electronic circuits. By controlling the resistance and capacitance in the circuit, engineers can tailor the discharge rate to meet specific requirements, paving the way for efficient and reliable electronic systems.

Energy Stored in a Capacitor

Just as a battery stores electrical energy, a capacitor also has the remarkable ability to accumulate and release energy. Imagine a capacitor as a miniature energy reservoir, capable of storing electrical charge like a sponge soaks up water.

Capacitance and Stored Energy:

The energy stored in a capacitor is directly proportional to its capacitance and the square of the voltage across it. Mathematically, it can be expressed as:

E = ½ * C * V²

where:

• E is the energy stored (in joules)
• C is the capacitance (in farads)
• V is the voltage across the capacitor (in volts)

Storing Charge:

Capacitors store electrical energy in the form of separated charge. When a voltage is applied across a capacitor, positive charges accumulate on one plate, while an equal number of negative charges gather on the opposite plate. This separation of charge creates an electric field within the capacitor, which stores the energy.

Capacitor as a Temporary Reservoir:

Unlike batteries, capacitors cannot generate energy continuously. They act as temporary reservoirs, storing energy for a limited time. Once the voltage is removed, the charges recombine, and the stored energy is released.

Applications of Stored Energy:

The ability of capacitors to store energy finds applications in various electronic devices. They can act as:

• Power sources in electronic circuits during brief power outages
• Energy buffers to smooth out fluctuations in power supply
• Timing elements in circuits that require a controlled delay

Capacitors are versatile energy storage devices that play a crucial role in electronics. Their ability to store and release energy is a fundamental property that makes them indispensable components in a wide range of applications, from simple circuits to sophisticated electronic systems.

Capacitor Charging and Discharging Circuits: Exploring the Electrical Playground

In the world of electronics, capacitors play a vital role in storing and releasing electrical energy. Understanding how capacitors charge and discharge is essential for anyone who wants to delve into the fascinating realm of circuits.

Charging a Capacitor

Imagine a capacitor as a tiny electrical reservoir. When you connect it to a battery, electrons start to flow into its plates, creating a positive charge on one plate and a negative charge on the other. This flow of charge is known as charging current. The rate at which a capacitor charges depends on its capacitance and the voltage applied to it.

Discharging a Capacitor

The fun doesn’t stop when you disconnect the capacitor from the battery. Electrons now have a chance to flow back from the negative plate to the positive plate, releasing the stored energy. This process is called discharging. Just like charging, discharging has its own rate, determined by the capacitor’s capacitance and the resistance in the circuit.

Circuit Configurations

Charging Circuits:
– Series RC Circuit: The capacitor is connected in series with a resistor, which limits the charging current and determines the time constant (the time it takes for the capacitor to reach approximately 63% of its maximum charge).

Discharging Circuits:
– Parallel RC Circuit: The capacitor is connected in parallel with a resistor, allowing the charge to flow freely and discharge the capacitor quickly.
– Series RL Circuit: The capacitor is connected in series with an inductor, which opposes changes in current and slows down the discharge process.

Applications

Capacitor charging and discharging circuits find numerous applications in electronics, including:
– Energy storage: Capacitors can store electrical energy for later use in applications like camera flashes and portable devices.
– Filtering: They can remove unwanted frequency components from signals, such as in audio filters.
– Timing: Capacitors play a crucial role in timers and oscillators, controlling the timing of electronic circuits.
– Energy recovery: In regenerative braking systems, capacitors store energy during braking and release it during acceleration.

Capacitor charging and discharging circuits are the building blocks of many electronic systems. By understanding how capacitors work, you can create circuits that store, release, or manipulate electrical energy with precision. So, whether you’re a hobbyist or an engineer, delve into the world of capacitor circuits and unleash your creativity!