Determining Charge Stored On A Capacitor: Q = Cv

To determine the charge stored on a capacitor, utilize the formula Q = CV, where Q represents the charge in coulombs (C), C is the capacitance in farads (F), and V is the voltage across the capacitor in volts (V). This formula demonstrates the direct proportionality between charge, capacitance, and voltage, allowing you to calculate the charge stored on a capacitor provided you know its capacitance and the voltage applied to it.

Capacitance: The Foundation of Charge Storage

In the realm of electricity, capacitance stands as the cornerstone of charge storage, enabling devices to accumulate and release electric charge at will. This remarkable ability, measured in farads (F), determines the capacitance of a capacitor, which in turn dictates the amount of charge it can hold.

Think of a capacitor as an electrical reservoir, akin to a water tank. Just as the tank’s capacity determines the volume of water it can store, the capacitance of a capacitor governs the quantity of charge it can accumulate. The larger the capacitance, the more charge it can hold.

Charge: The Accumulated Electricity

In the realm of electrical components, capacitors reign supreme as maestros of charge storage. Envision a capacitor as a tiny energy reservoir, a sponge capable of absorbing and releasing electric charge at the blink of an eye. This remarkable attribute is quantified by a fundamental parameter known as charge, measured in the unit of coulombs (abbreviated as C).

Charge embodies the essence of an electric field, representing the collective buildup of electrical energy within a capacitor. It’s akin to a tiny army of electrons, each carrying a negative charge, and a matching battalion of positive charges congregating on the opposite side of the capacitor’s plates. This dance of charges creates an electrical tug-of-war, giving rise to capacitance, the capacitor’s ability to store charge.

Now, let’s delve into a crucial relationship that governs charge: its direct proportionality to capacitance and voltage. Imagine a capacitor as a flexible rubber sheet. The larger the sheet (higher capacitance), the more charge it can accommodate. Similarly, the greater the voltage applied across the capacitor’s plates (higher voltage), the more charge it can store. This captivating interplay is succinctly captured by the formula:

Charge (Q) = Capacitance (C) x Voltage (V)

This elegant equation underscores the intricate connection between these three electrical parameters, showcasing how they dance together to orchestrate charge storage. By deftly manipulating capacitance and voltage, we can tailor a capacitor’s ability to accumulate and release charge, shaping its electrical behavior to suit our needs.

Voltage: The Driving Force Behind Charge

Imagine a capacitor as a reservoir of electrical charge, ready to store and release electricity at your command. Voltage is akin to a dam that controls the flow of water into and out of the reservoir. The greater the voltage, the higher the pressure, and the more charge the capacitor can hold.

Just as the height difference between a waterfall and a pool determines the water’s potential energy, the potential difference between the plates of a capacitor determines the voltage. This difference in electrical potential drives the accumulation of charge on the plates.

As the voltage increases, the potential energy stored in the capacitor rises. This energy attracts positive charges to one plate and negative charges to the other, creating an unbalanced distribution of electrical energy. The higher the voltage, the greater the imbalance, and the more charge that flows onto the capacitor plates.

The relationship between voltage and charge is directly proportional. This means that as the voltage increases, so does the amount of charge that the capacitor can store. This relationship is essential for understanding how capacitors work as energy storage devices.

Energy: The Stored Potential within a Capacitor

In the realm of electrical components, capacitors hold a unique ability to store electrical energy, much akin to a reservoir retaining water. This stored potential, measured in joules (J), is the hidden power that makes capacitors indispensable in a myriad of electronic circuits.

Unveiling the Relationship

The energy (E) stored in a capacitor forms an intriguing connection with its capacitance (C) and the charge (Q) it accumulates. This relationship is elegantly captured by the equation:

E = 1/2 * Q^2 * C

Exploring the Interdependence

This equation unveils a profound interdependence between these electrical properties. The stored energy is directly proportional to the square of the charge, indicating that the more charge a capacitor can hold, the greater its potential for storing energy. Moreover, the energy is positively correlated with capacitance, meaning that capacitors with larger capacitance can store more energy for a given charge.

Harnessing the Power of Capacitors

This stored energy is not merely a passive byproduct; it serves a crucial role in many electronic applications. For instance, capacitors in power supplies smooth voltage fluctuations, ensuring a steady flow of electricity. In electronic circuits, they act as temporary power reservoirs, releasing their stored energy during voltage dips or sudden current demands.

The stored energy within a capacitor is a fundamental aspect of its operation. Understanding the relationship between energy, charge, and capacitance empowers us to harness the full potential of these versatile components in various electronic systems. Whether it’s storing energy for future use or smoothing out voltage fluctuations, capacitors continue to play a vital role in the realm of electronics.

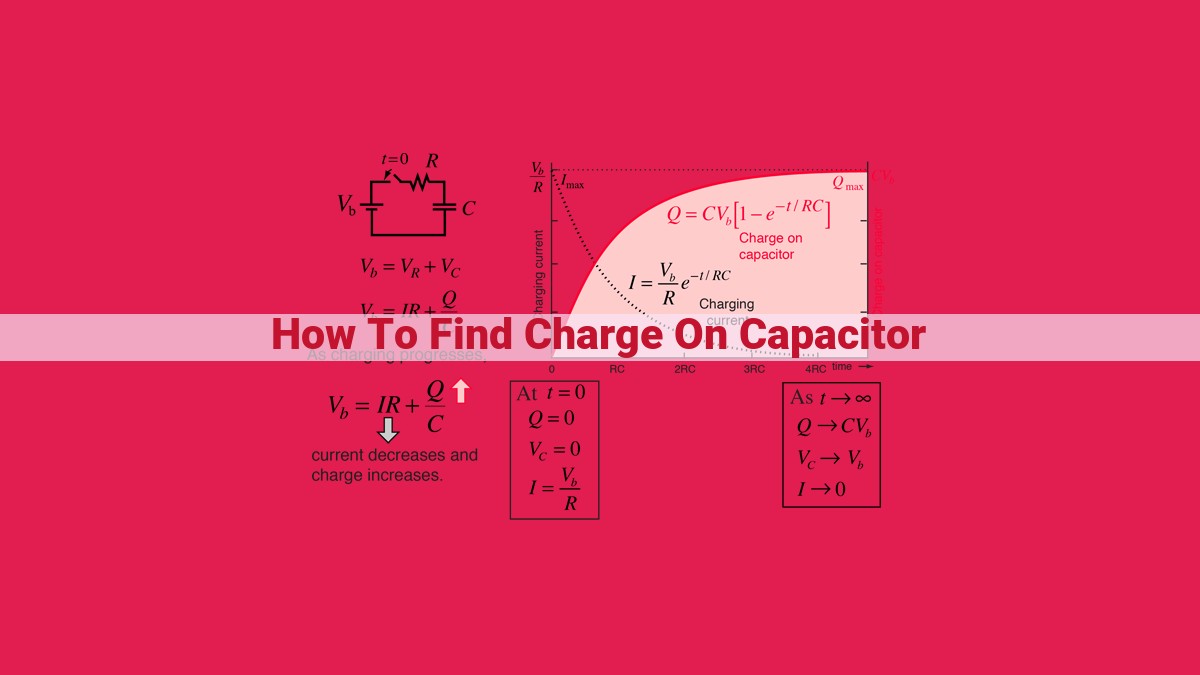
The Charge-Capacitance-Voltage Relationship

In the realm of electronics, capacitors play a pivotal role in storing electric charge. Capacitance (measured in farads, F) represents a capacitor’s ability to hoard this charge, determining the amount it can hold. Voltage (measured in volts, V), on the other hand, acts as the driving force behind this charge accumulation.

The relationship between these three fundamental parameters is elegantly expressed by the formula:

Q = CV

where:

• Q is the charge stored on the capacitor (measured in coulombs, C)
• C is the capacitance of the capacitor (measured in farads, F)
• V is the voltage across the capacitor (measured in volts, V)

This formula serves as a powerful tool for predicting the charge that a capacitor will store. By manipulating the capacitance or voltage, engineers can tailor capacitors to specific applications. For instance, a capacitor with a high capacitance can store more charge at a given voltage, making it ideal for energy storage.

In essence, the charge-capacitance-voltage relationship is the cornerstone of understanding capacitor behavior. It empowers engineers to harness the power of capacitors in a myriad of electronic devices, from smartphones to industrial machinery.

Capacitance: The Foundation of Charge Storage

Capacitance, measured in farads (F), is like a sponge that soaks up electric charge. Just as a larger sponge holds more water, a capacitor with a higher capacitance can store more charge. Understanding capacitance is crucial to grasping the workings of electronic circuits.

Charge: The Accumulated Electricity

Electric charge, measured in coulombs (C), represents the amount of electricity stored on a capacitor. Charge and capacitance go hand in hand. A capacitor with greater capacitance can hold a larger charge, just like a bigger bucket can hold more liquid.

Voltage: The Driving Force Behind Charge

Voltage, measured in volts (V), is the potential difference between the two plates of a capacitor. It’s like the pressure that pushes water into a sponge. Higher voltage drives more charge to accumulate on the capacitor plates.

Energy: The Stored Potential

Capacitors store energy, measured in joules (J), like a compressed spring. The energy stored is proportional to the square of both charge and capacitance. Think of it as a rubber band stretched taut; the more it’s stretched or the thicker it is, the more energy it stores.

The Charge-Capacitance-Voltage Relationship

The formula Q = CV is the cornerstone of capacitor understanding. It relates charge (Q), capacitance (C), and voltage (V). This equation predicts the amount of charge that can be stored on a capacitor, just like a recipe predicts how much batter to mix for a cake.

Measuring Units: Quantifying Electrical Properties

Measuring electrical properties requires specific units:
– Coulombs (C): Measures the amount of charge, like counting electrons.
– Farads (F): Quantifies capacitance, like the capacity of a water bucket.
– Volts (V): Measures the potential difference, like the voltage in a battery.

Using these units ensures consistent and accurate communication in the realm of electronics.

Capacitance, Charge, Voltage: A Unified Understanding

In the realm of electronics, capacitors play a pivotal role, serving as the gatekeepers of electrical energy. Their ability to store and release charge makes them essential components in a wide range of devices. To comprehend their operation, let’s embark on a journey into the world of capacitance, charge, and voltage.

Capacitance: The Conduit of Charge

Capacitance, measured in farads (F), embodies a capacitor’s capacity to store charge. Just as a bucket can hold water, a capacitor can hold electric charge. The more capacitance, the greater the amount of charge it can accommodate.

Charge: The Electrical Essence

Charge, expressed in coulombs (C), represents the amount of accumulated electricity on a capacitor. It’s like the volume of water in a bucket. A higher charge indicates a greater concentration of electrical particles.

Voltage: The Catalyst of Charge

Voltage, measured in volts (V), is the potential difference between the capacitor’s plates. Think of it as the water pressure in a hose. The higher the voltage, the greater the force driving the accumulation of charge on the plates.

Energy: The Stored Potential

Like a coiled spring, a charged capacitor possesses stored energy, measured in joules (J). This energy is proportional to the square of the charge and the capacitance. The more charge and capacitance, the greater the stored energy.

The Charge-Capacitance-Voltage Nexus

These three quantities are intertwined by the fundamental equation:

Q = CV

Where:

• Q is charge (in coulombs)
• C is capacitance (in farads)
• V is voltage (in volts)

This equation reveals that charge is directly proportional to both capacitance and voltage.

Measuring Electrical Properties

To quantify these electrical properties, we use specific units:

• Coulombs (C): Unit of charge
• Farads (F): Unit of capacitance
• Volts (V): Unit of voltage

By understanding these units, we can precisely describe the characteristics of capacitors.

Calculating Charge on a Capacitor

To determine the charge stored on a capacitor, we simply use the equation:

Q = C x V

For example, if a capacitor with a capacitance of 2 farads is charged with a voltage of 5 volts, the charge stored on the capacitor would be:

Q = 2 F x 5 V = 10 C

This calculation provides a clear understanding of the relationship between capacitance, voltage, and charge, empowering us to design and analyze circuits effectively.