Calculating Angle X: Comprehensive Guide To Measurement And Properties
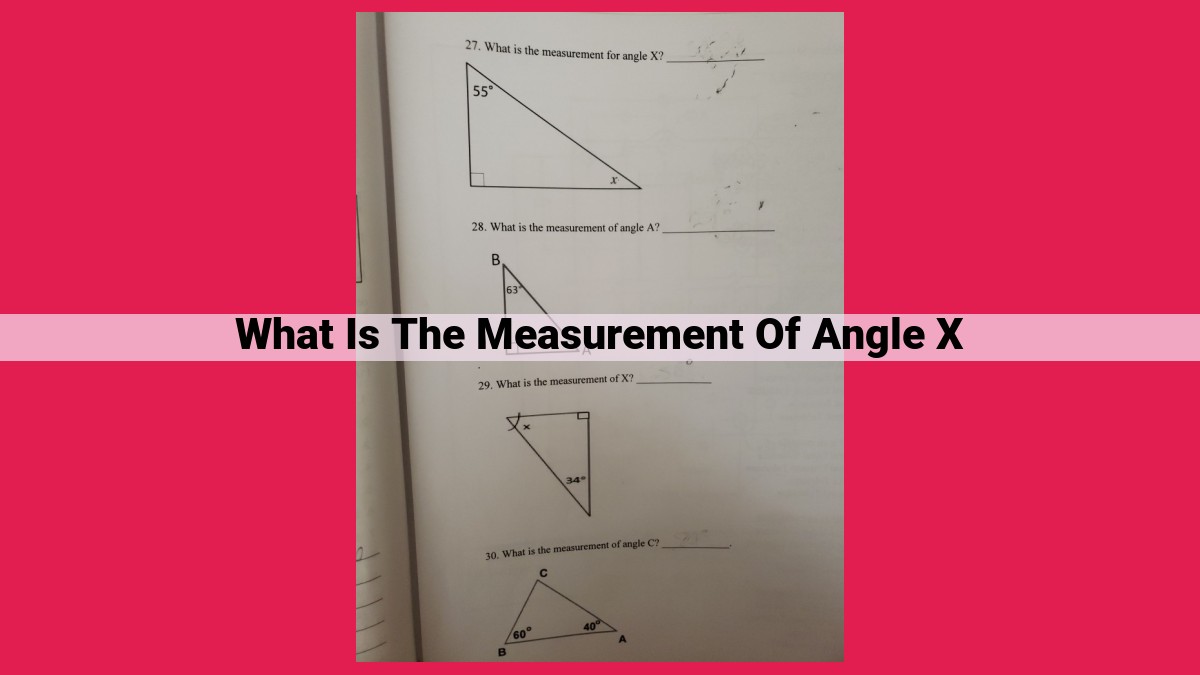
Determining the Measurement of Angle X: Angle X, mentioned in the article outline, is likely discussed within one of the sections on angle types, measurement, or relationships. To find its specific measurement, readers would need to refer to the corresponding section, where the angle’s characteristics and any relevant equations or theorems would be presented to calculate its value accurately.
Types of Angles: Unraveling the Geometric Puzzle
In the realm of geometry, angles play a pivotal role, serving as the cornerstone of various calculations and applications. To unravel the intricacies of this geometrical concept, let’s explore the diverse types of angles.
Acute Angles: The Sharp Edge of Geometry
Imagine a sharp corner in your room or the pointed tip of a pencil. These are examples of acute angles. Acute angles measure less than 90 degrees, characterized by their narrow and pointed appearance.
Obtuse Angles: The Expansive Embrace
On the other end of the spectrum, we have obtuse angles. Think of a wide-open door or the broad edge of a table. Obtuse angles measure more than 90 degrees but less than 180 degrees. Their expansive nature sets them apart from their acute counterparts.
Right Angles: The Precise 90-Degree Standard
The precise 90-degree mark defines a right angle. You’ll find right angles in the corners of squares and rectangles, forming perfect 90-degree intersections. In geometry, right angles are often represented by a square with four equal sides.
Straight Angles: The Unbroken Line
Finally, a straight angle measures exactly 180 degrees. It can be visualized as a straight line or a half-turn. Straight angles are commonly found in the edges of doors and the straight edges of tables, representing the complete 180-degree span.
Understanding the different types of angles is essential for navigating the world of geometry. Whether you’re measuring a corner in your home or calculating the angle of a roof, these classifications provide a solid foundation for exploring the fascinating world of angles.
Units of Angle Measurement: Navigating the Realm of Degrees, Radians, and Gradients
In the vast world of angle measurement, we encounter a trio of units that serve as our guiding stars: degrees, radians, and gradients. Each with its unique characteristics and applications, these units provide the language through which we quantify the world of angles.
Degrees: A Familiar Constant
The most familiar unit of angle measurement, the degree, has been with us for centuries. Imagine a circle, a perfect embodiment of completeness and unity. Divide this circle into 360 equal parts, and each slice represents one degree. This ubiquitous unit finds its home in countless applications, from measuring the tilt of the Earth’s axis to navigating the stars in the night sky.
Radians: The Mathematician’s Delight
Radians, on the other hand, are the darling of the mathematical world. They are defined as the ratio of the length of an arc to the radius of the circle from which it is drawn. This elegant and natural unit simplifies trigonometric calculations, making it the preferred choice for advanced mathematics and engineering.
Gradients: A Subtle Shift
Rounding out our trio is the gradient, a unit that is essentially a refinement of the degree. One gradient equals 1/100th of a right angle, or 0.9 degrees. This precision-oriented unit is particularly useful in fields like surveying and cartography, where even the smallest angular deviations can have significant implications.
Choosing the Right Unit
The choice of which unit to use depends on the context and the field of application. Degrees are widely accepted and intuitive for everyday use and basic geometry. Radians, with their mathematical elegance, excel in trigonometry and calculus. Gradients, with their enhanced precision, are particularly valuable in specialized domains.
Mastering Angle Measurement
Whether you’re a budding mathematician, an aspiring engineer, or simply someone who wants to understand the world around you, mastering angle measurement is an invaluable skill. By embracing these three units and their interrelationships, you can unlock a deeper understanding of the world of angles and all that it entails.
Using Protractors for Accurate Angle Measurement
Unlocking the Secrets of Angle Measurement
Measuring angles is not just a skill for mathematicians; it’s a valuable tool in countless real-world applications. Whether you’re an architect designing a building or an artist sketching a masterpiece, understanding angle measurement is crucial.
In geometry, angles represent the amount of turn between two intersecting lines. Protractors, our trusty companions, help us accurately measure these angles. Imagine a protractor as a half-circle with a scale marked in degrees or radians.
Step-by-Step Protractor Precision
Using a protractor is a simple yet effective technique. First, place the protractor’s center point on the vertex of the angle. Align the protractor’s zero mark with one of the angle’s rays. Then, read the value on the scale where the other ray intersects the protractor.
For acute angles, which are less than 90 degrees, the measurement will be within the first quadrant of the protractor. For obtuse angles, greater than 90 degrees, you’ll find the measurement in the adjacent quadrant.
Mastering the Art of Angle Measurement
To ensure accuracy, always position the protractor precisely and read the measurement perpendicular to the scale. If your angle extends beyond the protractor’s range, use an additional protractor or the complementary angle concept to complete the measurement.
With a little practice, using protractors becomes second nature. This skill will empower you to explore the world of geometry and solve a multitude of problems. So, let’s embark on this angle-measuring adventure together!
Angle Bisector: Dividing Angles with Precision
In the realm of geometry, the angle bisector emerges as a pivotal tool for dividing angles with utmost accuracy. It’s the gatekeeper of equal angles, ensuring fairness and harmony in the geometric world.
An angle bisector is defined as a line or ray that divides an angle into two **congruent angles. Picture this: you have a delicious pizza, and you want to share it equally with your friend. The angle bisector is like the pizza cutter, slicing that angle in half, ensuring each of you gets a fair share.
Constructing Angle Bisectors
Constructing angle bisectors is a task that requires precision and finesse. You’ll need a trusty compass and a straightedge to perform this geometric magic. Here’s a step-by-step guide:
- Center the compass on the vertex of the angle.
- Adjust the compass to a radius greater than half the length of the angle’s sides.
- Draw two arcs that intersect inside the angle.
- Connect the intersection points with a straight line.
Voilà! You’ve successfully constructed the angle bisector, the mediator of angles.
Angle Trisection: The Elusive Challenge
While angle bisection is a straightforward affair, its close cousin, angle trisection, presents a different level of complexity. Trisecting an angle, or dividing it into three equal parts, is a geometric conundrum that has puzzled mathematicians for centuries.
Unlike angle bisection, there is no known foolproof method to trisect an angle using only a compass and straightedge. It’s one of those mathematical mysteries that continues to tantalize the curious and fuel the quest for knowledge.
Constructing Angle Bisectors: Unlocking the Secrets of Angle Division
In the realm of geometry, understanding how to construct angle bisectors is a fundamental skill. An angle bisector is a line or ray that divides an angle into two equal parts. It serves as a powerful tool for mathematicians and students alike, enabling them to solve complex geometric problems with ease.
To embark on the journey of constructing angle bisectors, we shall arm ourselves with two essential tools: a trusty compass and a precise straightedge. With these trusty companions by our side, we can conquer the challenge of bisecting any angle with accuracy.
Step 1: Center the Compass on the Vertex
The first step is to position the compass at the vertex of the angle, where the two rays meet. Adjust the compass to have the same radius on both sides. This will help us create two equal arcs in the next step.
Step 2: Draw Intersecting Arcs
Once the compass is in place, gently draw two arcs that intersect outside the angle. These arcs should cross each other at two distinct points.
Step 3: Connect the Intersection Points
Using a straightedge, carefully connect the two intersection points created in the previous step. This line or ray is the angle bisector. It will divide the angle into two equal parts, each measuring exactly half of the original angle.
A Note on Angle Trisection
While constructing angle bisectors is a straightforward process, the task of trisecting an angle, or dividing it into three equal parts, is much more challenging. Mathematicians have proven that trisecting an angle using only a compass and straightedge is impossible, making it a fascinating mathematical enigma.
The challenge of angle trisection
The Elusive Angle Trisection: A Mathematical Enigma
In the realm of geometry, angle trisection presents a tantalizing challenge that has intrigued mathematicians for centuries. Unlike angle bisection, which involves dividing an angle into two equal parts, trisection demands the division of an angle into three equal parts. This deceptively simple task has proven to be remarkably complex, leaving mathematicians grappling with its enigma.
The Quest for Trisection
The ancient Greeks, renowned for their geometric prowess, were among the first to attempt angle trisection. Yet, despite their ingenuity, they encountered a fundamental obstacle. It turned out that trisection, using only a compass and straightedge, is impossible for certain angles. This astounding discovery shattered the belief that all geometric constructions could be achieved with these basic tools.
Mathematical Proof
The impossibility of angle trisection was eventually proven in the 19th century by the brilliant mathematician Pierre Wantzel. He demonstrated that trisecting an angle using only a compass and straightedge would require solving a cubic equation, which is beyond the scope of tools allowed in classical geometry.
Special Cases and Approximations
While general angle trisection remains an elusive goal, certain special cases do admit solutions. For example, trisecting a 60-degree angle is possible because it can be divided into three equal parts of 20 degrees each. However, for most angles, only approximations are available.
Practical Implications
Despite the mathematical challenges, angle trisection has practical implications in various fields. Engineers rely on accurate angle measurement in design and construction. Artists use angles to create perspective and depth in their work. Even in everyday life, we encounter angles in architecture, carpentry, and even knitting.
The challenge of angle trisection serves as a testament to the intricate nature of mathematics. It shows that even seemingly simple problems can harbor hidden complexities. Yet, the pursuit of trisection has led to new insights into the fundamentals of geometry and the power of mathematical reasoning. And so, the enigma of angle trisection continues to inspire and challenge mathematicians to this day.
Supplementary Angles: The Balancing Act
Supplementary angles are a fascinating concept in the realm of geometry. They are pairs of angles that, when added together, measure exactly 180 degrees. This unique partnership between angles creates a harmonious balance that plays a pivotal role in many geometric constructions.
One of the most common examples of supplementary angles is a straight line. When two lines intersect at a point, they form four angles. The opposite angles created by these intersecting lines are always supplementary, forming a straight line that measures 180 degrees.
Another example of supplementary angles is a linear pair. When two rays share a common endpoint, they form two adjacent angles. These adjacent angles form a linear pair, and their sum is always 180 degrees. This property of linear pairs is essential for understanding and solving various geometric problems.
In addition to these basic examples, supplementary angles also appear in more complex geometric figures. For instance, in a parallelogram, opposite angles are supplementary, ensuring the parallel sides remain parallel. Understanding these relationships between angles is crucial for analyzing and constructing various geometric shapes.
Vertical Angles and Linear Pairs: Supplementary Angles in Action
In the realm of angles, supplementary angles hold a special place. These angles, when combined, form a straight line or 180 degrees. They play a crucial role in understanding the relationships between lines and angles.
One intriguing example of supplementary angles is vertical angles. These are angles that are formed when two lines intersect. Imagine a crossroad where two roads intersect. The angles at each corner are vertical angles. They are always equal in measure, like two peas in a pod.
Another notable manifestation of supplementary angles is linear pairs. These are adjacent angles that form a straight line. Think of a seesaw or a teeter-totter. When the seesaw is balanced, the angles on either side create a linear pair. These angles are like inseparable twins, always equaling 180 degrees.
In the world of shapes, vertical angles and linear pairs contribute to the stability and symmetry we observe. They ensure that triangles have equal base angles, rectangles have four right angles, and parallelograms have opposite angles that are supplementary.
Understanding these relationships is like unlocking a secret code to unravel the mysteries of geometry. Architects use these principles to design buildings with balanced facades, engineers rely on them for structural stability, and artists employ them to create pleasing compositions.
So, next time you encounter vertical angles or linear pairs, remember their special relationship. They are the guardians of symmetry and stability, the invisible forces that shape our geometric world.
Relationships between Adjacent Angles and Supplementary Angles
In the realm of angles, two intriguing relationships emerge: adjacent angles and supplementary angles. Understanding these relationships is crucial for navigating the intricate world of geometry.
Adjacent Angles:
Imagine two angles that share a common side. These are known as adjacent angles. Their sides form a straight line, creating a linear pair. The sum of adjacent angles always equals 180 degrees. In other words, they complement each other to form a straight line.
Supplementary Angles:
Now, let’s introduce supplementary angles. These are two angles that, when added together, form a straight line, totaling 180 degrees. Unlike adjacent angles, supplementary angles do not necessarily share a common side. They can be separated but their combined angle measures always equate to a straight line.
The Connection:
The connection between adjacent angles and supplementary angles lies in their mathematical relationship. If two adjacent angles form a linear pair, they are also supplementary. Conversely, if two angles are supplementary, they can be divided into adjacent angles that form a linear pair.
Real-Life Applications:
Understanding adjacent and supplementary angles is not just a theoretical exercise. It has practical applications in various fields. In architecture, for instance, architects use these principles to design buildings with symmetrical facades and optimal sunlight exposure. In navigation, mariners rely on angle measurement to determine their direction and avoid collisions.
Remember, angles hold the key to understanding geometry and its real-world applications. By exploring relationships like adjacency and supplementation, we unlock the secrets to measuring and manipulating angles with precision, paving the way for innovative designs and accurate navigation.
Angle Measurement: A Journey to Understanding Angles
Angles, those elusive geometric concepts, play a pivotal role in shaping our world. From the towering skyscrapers that pierce the heavens to the intricate patterns found in nature, angles are the angles are the silent orchestrators behind the beauty and functionality we see around us. In this comprehensive guide, we embark on an angle-faring adventure to demystify these alluring geometric constructs.
Complementary Angles: A Harmonious Pair
Definition: Complementary angles are two angles that, when combined, form a right angle (90 degrees). Picture a T-shaped intersection, where the vertical and horizontal lines meet at a 90-degree angle. The angles formed by the vertical line and each of the horizontal lines are complementary pairs.
These harmonious angles possess a unique relationship. When you add the measure of two complementary angles, the result is always 90 degrees. It’s like a perfectly balanced dance routine, where the addition of one angle seamlessly complements the other.
Properties of Complementary Angles
- Sum of measures is 90 degrees: The cornerstone of complementary angles is their constant sum of 90 degrees.
- Adjacent angles: Complementary angles are often adjacent, meaning they share a common side and vertex.
- Acute and obtuse angles: Complementary angles come in pairs, with one acute angle (less than 90 degrees) and one obtuse angle (greater than 90 degrees).
- Vertical angles: A pair of vertically opposite angles formed by intersecting lines are always complementary.
Recognizing Complementary Angles in the Real World
Complementary angles find practical applications in various domains. In architecture, they help create balanced and aesthetically pleasing structures. Engineering utilizes complementary angles to ensure optimal load distribution and stability in bridges and buildings. Even everyday life offers instances of complementary angles, such as the hands of a clock indicating 3:00 and 9:00.
By mastering the concept of complementary angles, you unlock a key to unlocking the secrets of the geometric world and beyond. They are the harmonious partners of the angle family, contributing to the beauty and functionality that surrounds us.
Complementary Angles: A 90-Degree Partnership
Unveiling the Complementary Story
In the realm of angles, we often encounter pairs that form a special bond, known as complementary angles. These angles, when combined, create a perfect right angle, measuring a precise 90 degrees. It’s as if they’re two pieces of a puzzle, fitting together snugly to form a complete and harmonious whole.
The Essence of Complementary
Complementary angles are characterized by their distinct characteristics. One angle is acute, meaning it measures less than 90 degrees. Its counterpart is obtuse, measuring greater than 90 degrees. Together, they form a perfect 90-degree angle, symbolizing balance and harmony.
Real-Life Examples: A World of Complementary
The world around us is teeming with examples of complementary angles. Observe the corners of a rectangular picture frame or the intersecting lines of a crossroad. In architecture, arches and domes rely on the precise alignment of complementary angles to ensure stability and aesthetics.
Practical Applications: Beyond Geometry
Beyond geometry, complementary angles find practical applications in various fields. Engineers use them to design bridges and structures that withstand forces and maintain stability. Navigators rely on complementary angles to calculate ship trajectories and pinpoint their locations on the open seas.
Complementary angles serve as a cornerstone in the world of angles. They represent the perfect partnership, where two distinct measurements come together to create a harmonious whole. From the elegance of geometry to the practicality of engineering, complementary angles showcase the power of mathematical principles in our everyday lives. By understanding and utilizing these concepts, we gain a deeper appreciation for the intricate tapestry of our world.
The Role of Right Angles in Complementary Angles
In the world of geometry, angles play a pivotal role in shaping our understanding of shapes and their relationships. Complementary angles are a fascinating pair that work in harmony to create a special bond.
When two angles sum up to 90 degrees, they form a right angle. A right angle is often represented by a square corner or a perpendicular line. In the case of complementary angles, one of the angles will be a right angle, while the other will be an acute angle, which is less than 90 degrees.
Imagine two walls meeting at a corner. The angle formed by the walls is a right angle. Now, let’s take a look at the space above one of the walls. The angle formed by the wall and the ceiling is an acute angle. These two angles are complementary, as they add up to 90 degrees.
The significance of right angles in complementary angles lies in the fact that they provide a stable and predictable relationship. Unlike supplementary angles, which can vary in size, complementary angles are always bound by the right angle. This fixed relationship makes complementary angles incredibly useful in geometry and everyday applications.
For example, architects use complementary angles to design buildings that are both aesthetically pleasing and structurally sound. By ensuring that certain angles are right angles, they create a balance and stability that is essential for a safe and functional structure.
In summary, the role of right angles in complementary angles is to provide a fixed and predictable relationship that is essential for geometry and practical applications. Understanding the relationship between right angles and complementary angles helps us navigate the world of shapes and angles with confidence and precision.
Relationships Between Adjacent Angles and Complementary Angles
In the world of angles, relationships play a pivotal role. Among these relationships, the interplay between adjacent angles and complementary angles stands out.
Adjacent Angles:
Adjacent angles are two angles that share a common side and do not overlap. They can be visualized as adjacent rooms in a house, separated by a shared wall.
Complementary Angles:
Complementary angles are two angles whose sum equals 90 degrees. They can be thought of as puzzle pieces that fit together perfectly to form a right angle.
Connection Between Adjacent and Complementary Angles:
The relationship between adjacent angles and complementary angles is like a dance. When two adjacent angles add up to 90 degrees, they form a complementary angle pair. It’s as if the adjacent angles are two dancers, each taking half of the stage, creating a harmonious balance.
For example, imagine an isosceles right triangle. The two angles opposite the hypotenuse (the longest side) are both 45 degrees. These angles are adjacent and complementary, fitting together perfectly to form a right angle of 90 degrees.
Significance of This Relationship:
Understanding the connection between adjacent and complementary angles is crucial in geometry and its applications. Architects use this knowledge to design buildings with precise angles, while engineers rely on it for stability and balance in structures. Even in everyday life, we encounter angles, such as when parking a car at a 90-degree angle to the curb.
By grasping this relationship, we unlock the secrets of angle measurement, enabling us to navigate the world of shapes, angles, and their countless applications.
Angle Measurement: A Cornerstone in Our World
Angle measurement, a fundamental concept in mathematics, plays a pivotal role in our daily lives. It’s a key tool in architecture, engineering, design, and even everyday tasks like measuring the height of a tree or gauging the distance of a parked car.
Real-World Applications of Angle Measurement:
Imagine architects meticulously calculating the angles of a triangular roof to ensure its stability. Engineers use angle measurements to design bridges that can withstand the weight of heavy traffic. In the realm of construction, builders rely on accurate angle measurements to align walls, install windows, and ensure level floors.
Angles are also essential in navigation. Sailors use sextants to measure the angle between the horizon and the sun or stars, determining their latitude and longitude. Pilots rely on angle measurements to guide their planes along predetermined flight paths.
In the field of medicine, radiologists use computed tomography (CT) scans to create cross-sectional images of the body. These images rely on precise angle measurements to provide detailed anatomical views for accurate diagnosis.
Even in our homes, we encounter angle measurements. Interior designers use angles to arrange furniture, creating visually appealing and functional spaces. Landscapers rely on angle measurements to design sloping gardens and arrange plants in aesthetically pleasing ways.
So, whether we’re navigating the vast seas, exploring the depths of our own bodies, or simply decorating our homes, angle measurement is an underlying force that shapes our world and makes our lives more precise and efficient.
Angle Measurement in Practice: Applications and Context
The world around us is filled with angles. From the walls of buildings to the curves of bridges, angles define and shape our environment. Understanding angle measurement is not only essential for architects and engineers, but also for anyone who wants to navigate the world around them.
Architecture and Design
In architecture, angles play a crucial role in creating aesthetically pleasing and functional structures. The angles of the roof determine how the building will withstand wind and rain, while the angles of the windows and doors impact the amount of natural light that enters the building.
Engineering and Construction
In engineering, precise angle measurement is essential for structural integrity. The angles of support beams and trusses determine how much weight they can carry, while the angles of gears and pulleys affect the efficiency of machines.
Everyday Applications
Angle measurement is not limited to professional fields. In everyday life, we encounter angles in countless situations. The angle of a car’s windshield affects visibility, while the angle of a cutting board helps us slice food with precision.
By understanding angle measurement, we can not only grasp the geometric concepts that underlie the world around us but also appreciate the practical applications of this fundamental mathematical tool.
Common Mistakes in Angle Measurement and Tips for Accuracy
Measuring angles accurately is crucial in various fields, from engineering to everyday tasks. However, common mistakes can lead to inaccuracies and incorrect results. Here’s a guide to help you avoid these pitfalls and achieve precise angle measurements:
Placing the Protractor Incorrectly
A common mistake is misaligning the protractor’s center with the vertex of the angle. Always ensure that the center point of the protractor is directly over the angle’s vertex. The base of the protractor should rest flush against one of the angle’s rays.
Using the Wrong Scale
Some protractors have multiple scales (e.g., degrees, radians). Choose the correct scale for your measurements and read the angle accurately. Double-check the scale before and after taking the measurement to avoid confusion.
Estimating the Angle
Avoid approximating or eyeballing the angle. Use the protractor’s markings to determine the precise angle. Align the protractor carefully and read the angle to the nearest degree or the desired unit of measurement.
Rounding Errors
When converting angles from one unit to another (e.g., degrees to radians), round the result to an appropriate number of decimal places. Avoid excessive rounding, as it can introduce inaccuracies.
Measuring Angles Incorrectly
Ensure that the protractor’s straight edge touches both rays of the angle. Avoid measuring angles that are too large or too small to fit on the protractor. If necessary, use a larger protractor or divide the angle into smaller parts for accurate measurements.
By following these tips, you can minimize errors and achieve accurate angle measurements. Remember, precise angle measurements are essential for various applications, from architectural designs to scientific experiments. Invest in a high-quality protractor, practice accurate measurement techniques, and strive for excellence in angle measurement.
Delving into Angle Measurement: Unlocking the Secrets of Angles
Angle measurement, the cornerstone of geometry and countless real-world applications, can often seem like an arcane subject. But fear not, for this comprehensive guide will demystify the world of angles, transforming you into a confident angle master.
Our journey begins with understanding the types of angles. Acute angles are shy and less than 90 degrees, while obtuse angles are bold and greater than 90 degrees. Then there are right angles, the epitome of perpendicularity, and straight angles, which stretch out to a full 180 degrees.
Next, we venture into the realm of angle measurement. Degrees, radians, and gradients are the units of choice, each with its unique strengths. Protractors, the trusty tools of angle measurement, help us pinpoint angles with accuracy.
Angle bisectors, the fair-minded mediators, divide angles into two equal parts. Constructing them with compasses and straightedges is a skill every angle enthusiast must possess. While angle trisection remains an elusive challenge, angle bisectors provide a satisfying compromise.
Supplementary angles are perfect companions, adding up to a neat 180 degrees. Vertical angles and linear pairs are prime examples of this harmonious partnership. Complementary angles, on the other hand, are like Yin and Yang, complementing each other to form a right angle of 90 degrees.
Finally, we explore the practical applications of angle measurement. From architecture to engineering, everyday life is filled with examples where angles play a crucial role. Common mistakes in angle measurement are highlighted, along with tips for optimizing accuracy.
So, embrace the power of angle measurement, unlocking the secrets of geometry and beyond. Let angles be your guide in navigating the world, from designing buildings to understanding the trajectory of a thrown ball. With the knowledge gained from this comprehensive guide, you will become an angle expert, ready to conquer any angle-related challenge that comes your way!
Emphasize their importance in geometry and related fields
Delving into Angle Measurement: Unraveling the Secrets of Angles
From the towering skyscrapers that grace our cities to the intricate designs of nature, angles play an indispensable role in shaping our world. Understanding angle measurement empowers us to appreciate the beauty and precision of our surroundings.
The Cornerstone of Geometry and Beyond
Angles form the foundation of geometry, the language of shapes and space. They serve as the building blocks for constructing triangles, quadrilaterals, and all manner of geometric figures. In fact, angle measurement is at the heart of every geometric theorem and proof, allowing us to deduce properties and relationships between shapes.
Moreover, angles extend their influence beyond geometry. They find crucial applications in fields such as architecture, engineering, and physics. Architects rely on precise angle measurements to design buildings that are both visually appealing and structurally sound. Engineers use angles to calculate the forces acting on bridges, while physicists employ them to describe the trajectories of projectiles and the behavior of light.
Unlocking the Secrets of Angles
To delve into the world of angles, we begin by exploring their different types. From acute and obtuse angles to right and straight angles, each type has its unique characteristics and properties. We then embark on a journey to measure angles using protractors, the trusty tools that help us determine the size of any angle with accuracy.
Next, we encounter the concept of the angle bisector, a line that divides an angle into two equal parts. Constructing angle bisectors is a fundamental skill that lays the groundwork for angle trisection, the more challenging task of dividing an angle into three equal parts.
Supplementary and Complementary: A Balancing Act
As we continue our exploration, we encounter the fascinating worlds of supplementary and complementary angles. Supplementary angles are a pair of angles that add up to 180 degrees, forming a straight line. They often appear in situations involving parallel lines and transversals. On the other hand, complementary angles are a pair that add up to 90 degrees, creating a right angle. They play a crucial role in trigonometry, the study of triangles and their properties.
The Power of Angle Measurement in Practice
The realm of angles extends far beyond the confines of a textbook. In the real world, angle measurement has countless applications. From the angles of a bicycle’s handlebars to the elevation angles of a telescope, understanding angles helps us make sense of our surroundings and solve practical problems.
By unraveling the secrets of angle measurement, we gain a deeper appreciation for the wonders of mathematics and its power to describe and interpret our world. Whether we are architects designing a masterpiece or students exploring the beauty of geometry, angle measurement serves as a fundamental tool that empowers us to unlock the mysteries of shapes and space
Reflect on the practical significance of angle measurement in various domains
Angle Measurement: A Powerful Tool in Shaping Our World
Angle measurement is not merely a concept confined to textbooks but a vital tool that finds practical applications in countless domains, shaping the world around us in subtle yet significant ways.
From designing breathtaking architectural marvels to constructing towering bridges that defy gravity, angle measurement plays a pivotal role. Architects and engineers rely on precise angle measurements to ensure structural integrity, stability, and aesthetics. Every truss, arch, and column is carefully angled to withstand forces and create visually appealing structures.
Angle measurement extends its reach into everyday objects as well. Whether it’s the angled handles of a coffee mug, the tapered body of a car, or the curved blades of a lawnmower, angles contribute to functionality, ergonomics, and design.
In the realm of science and technology, angle measurement enables us to probe the mysteries of the universe. From navigating celestial bodies using star angles to designing optical instruments that capture stunning cosmic images, angles serve as a crucial language for understanding and manipulating light and motion.
Moreover, angle measurement is essential for daily tasks, often without us even realizing it. Driving a car involves constant angle adjustments as we steer, playing sports requires precise coordination of limb angles, and even tying a knot relies on the correct alignment of angles.
Angle measurement has also been instrumental in advancing fields such as medicine, agriculture, and manufacturing. Surgeons utilize angle measurements for precise incisions and bone alignments, agronomists employ it for analyzing plant growth patterns, and manufacturers depend on it for accurate assembly and quality control.
In conclusion, angle measurement is a versatile and indispensable tool that has transformed the way we build, design, explore, and interact with the world around us. Its practical applications extend far beyond the classroom, shaping everything from our homes and cities to scientific discoveries and everyday tasks. Truly, angle measurement is a testament to the power of geometry in shaping our world.