Calculate The Y-Intercept Using Two Points: A Quick Guide With Examples

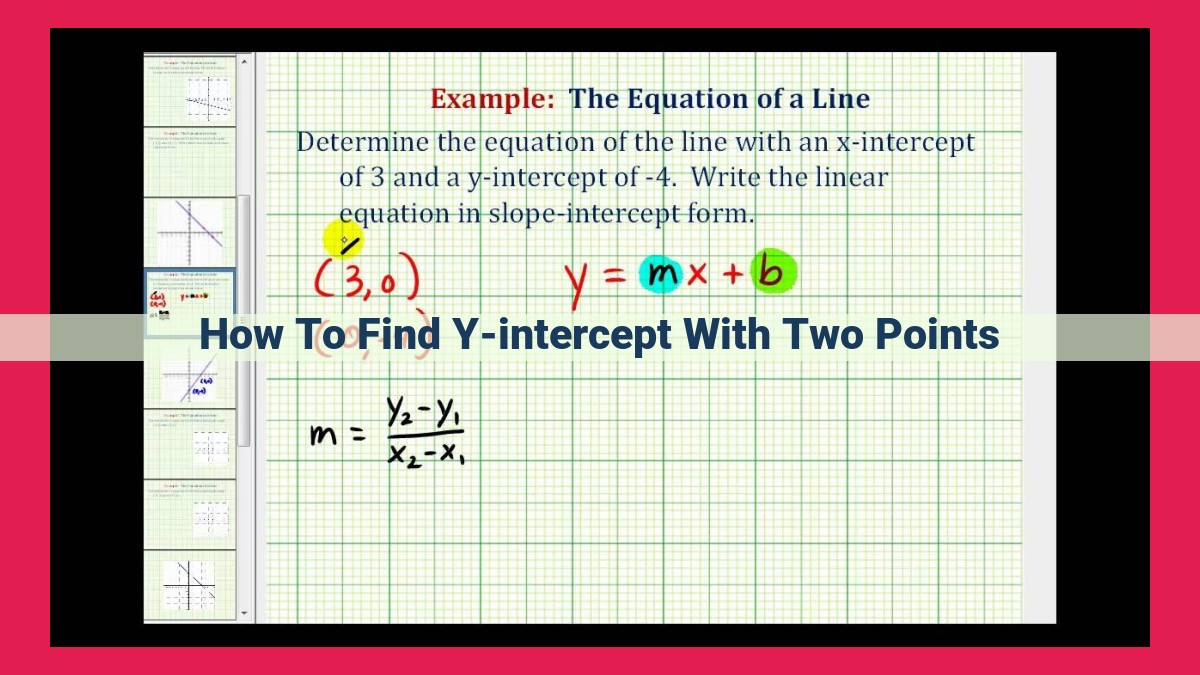
To find the y-intercept using two points, first calculate the slope (rise/run) between the points. Then, substitute the slope and one point into the slope-intercept form (y = mx + b), where m is the slope and b is the y-intercept. Solve for b to find the y-intercept. For example, given points (1, 3) and (3, 9), the slope is 2 and using the point (1, 3), the equation becomes 3 = 2(1) + b, which yields a y-intercept of 1.

Understanding the Secrets of Linear Equations

Let’s begin our journey by unraveling the mysteries of linear equations. To fully grasp these concepts, we’ll start with the basics:

Y-Intercept: The Equation’s Starting Point

Imagine a straight line gracefully crossing the y-axis. The point where this line kisses the y-axis is called the y-intercept. It tells us the value of y when x is zero.

Slope-Intercept Form: A Handy Equation Format

The slope-intercept form is a convenient way to write a linear equation. It looks like this: y = mx + b, where m represents the slope and b represents the y-intercept.

Two Points: Guiding Us to the Slope

To determine the slope of a line, we need two points through which it passes. The rise is the change in y-values between these points, while the run is the change in x-values. Dividing the rise by the run gives us the mighty slope!

Y-Coordinate: A Point’s Vertical Position

In a coordinate plane, the y-coordinate of a point tells us how high or low it is on the vertical axis (y-axis). It’s essentially the second number in an ordered pair like (x, y).

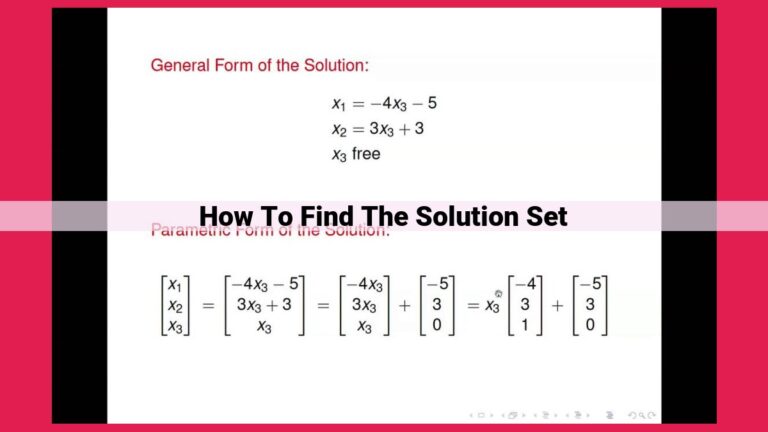
Finding the Y-Intercept Using Two Points

Imagine yourself as a detective unraveling the secrets of a mysterious line. The Y-intercept holds the key to understanding the line’s position on the coordinate plane. To uncover this secret, you need the tools of two points. Let’s embark on this detective journey to uncover the Y-intercept’s hidden value.

Step 1: Calculate the Slope

The slope, often symbolized by “m,” measures the line’s steepness. To find it, you’ll use the rise and run between the two given points. The rise is the difference in Y-coordinates, and the run is the difference in X-coordinates.

**Slope (m) = (Change in Y) / (Change in X)**

Step 2: Substitute Slope and Point

Once you have the slope, it’s time to introduce the slope-intercept form:

**y = mx + b**

where “b” represents the Y-intercept.

Plug the previously calculated slope (m) and one of the given points (x1, y1) into this equation.

Step 3: Solve for Y-Intercept

Now comes the grand finale. With the slope and point substituted, it’s time to solve for the Y-intercept (b). Isolate “b” on one side of the equation by subtracting “mx” from both sides.

**b = y - mx**

Voila! You have unmasked the secret identity of the Y-intercept using a pair of points. Now go forth, detective, and uncover the mysteries hidden within other lines.

Finding the Y-Intercept Using Two Points: A Comprehensive Guide

In the realm of mathematics, the concept of the y-intercept holds immense importance. It serves as a critical parameter in determining the behavior and position of a linear equation. This comprehensive blog post will delve into the intricacies of finding the y-intercept using two points. Along the way, we’ll unravel the fundamental concepts of slope-intercept form and equip you with practical steps to navigate this mathematical landscape confidently. So, fasten your seatbelts and let’s embark on this enlightening journey!

Understanding the Key Terms

Before we dive into the y-intercept, let’s establish a solid foundation by clarifying some key terms:

• Y-intercept: The point where a line crosses the y-axis when the x-coordinate is zero.
• Slope-intercept form: An equation of a line written in the format: y = mx + b, where m is the slope, and b is the y-intercept.
• Two points: Any two distinct points lying on the line.
• Rise: The vertical distance between the two points.
• Run: The horizontal distance between the two points.
• Y-coordinate: The vertical position of a point.

Finding the Y-Intercept Using Two Points

Equipped with these fundamental concepts, let’s unravel the steps involved in finding the y-intercept using two points:

1. Calculate the Slope: Determine the slope (m) of the line using the rise and run between the two points: m = (y2 – y1) / (x2 – x1).
2. Substitute Slope and One Point: Insert the calculated slope and one of the points (x1, y1) into the slope-intercept form: y = mx + b.
3. Solve for Y-Intercept: Solve the equation from Step 2 for b, which represents the y-intercept.

Example

Let’s solidify our understanding with a practical example. Consider two points on a line: (2, 5) and (4, 9).

1. Calculate the Slope: m = (9 – 5) / (4 – 2) = 2.
2. Substitute Slope and One Point: (5) = 2(2) + b.
3. Solve for Y-Intercept: Solving for b, we get b = 1.

Therefore, the y-intercept of the line passing through (2, 5) and (4, 9) is 1.

Practice Problems

To reinforce your understanding, here are a few practice problems:

1. Find the y-intercept of the line passing through points (3, 4) and (1, 2).
2. Calculate the y-intercept of the line whose slope is -1 and passes through the point (0, 5).

Mastering the art of finding the y-intercept using two points empowers you to solve a wide range of mathematical problems with precision. Whether you’re dealing with linear equations, graphs, or other mathematical applications, this technique will prove invaluable. So, embrace the concepts outlined in this post, practice regularly, and conquer the world of mathematics with confidence!

Finding the Y-Intercept: A Guide for Slope-Intercept Form Mastery

Understanding the Basics

Before we dive into the intricacies of y-intercepts, let’s establish the building blocks:

• Y-intercept: The point where a line crosses the y-axis, indicating the value of y when x is 0.
• Slope-intercept form: The equation of a line in the form y = mx + b, where m is the slope and b is the y-intercept.
• Two points: Any two distinct points that lie on the line.
• Rise: The change in y-coordinate between two points.
• Run: The change in x-coordinate between two points.

Finding the Y-Intercept with Two Points

To determine the y-intercept using two points:

1. Calculate the slope (m): Divide the rise by the run.
2. Substitute into slope-intercept form: Choose one point and plug in its coordinates and the calculated slope.
3. Solve for b (y-intercept): Isolate b by simplifying the equation.

Example:

Suppose we have two points on a line: (2, 5) and (4, 9). To find its y-intercept:

1. Slope (m): (9-5) / (4-2) = 2
2. Slope-intercept form: y = 2x + b
3. Substituting (2, 5): 5 = 2(2) + b
4. Solving for b: b = 1

Therefore, the y-intercept of the line is 1.

Practice Problems to Sharpen Your Skills

1. Find the y-intercept of the line passing through (1, 4) and (-2, 2).

2. A line has a slope of -3. If it passes through the point (2, -5), determine its y-intercept.

Understanding the y-intercept and its role in the slope-intercept form equips you with a powerful tool for analyzing linear relationships. By grasping the concepts and practicing with these examples, you can confidently solve real-world problems involving y-intercepts.