How To Calculate Radial Acceleration: A Step-By-Step Guide
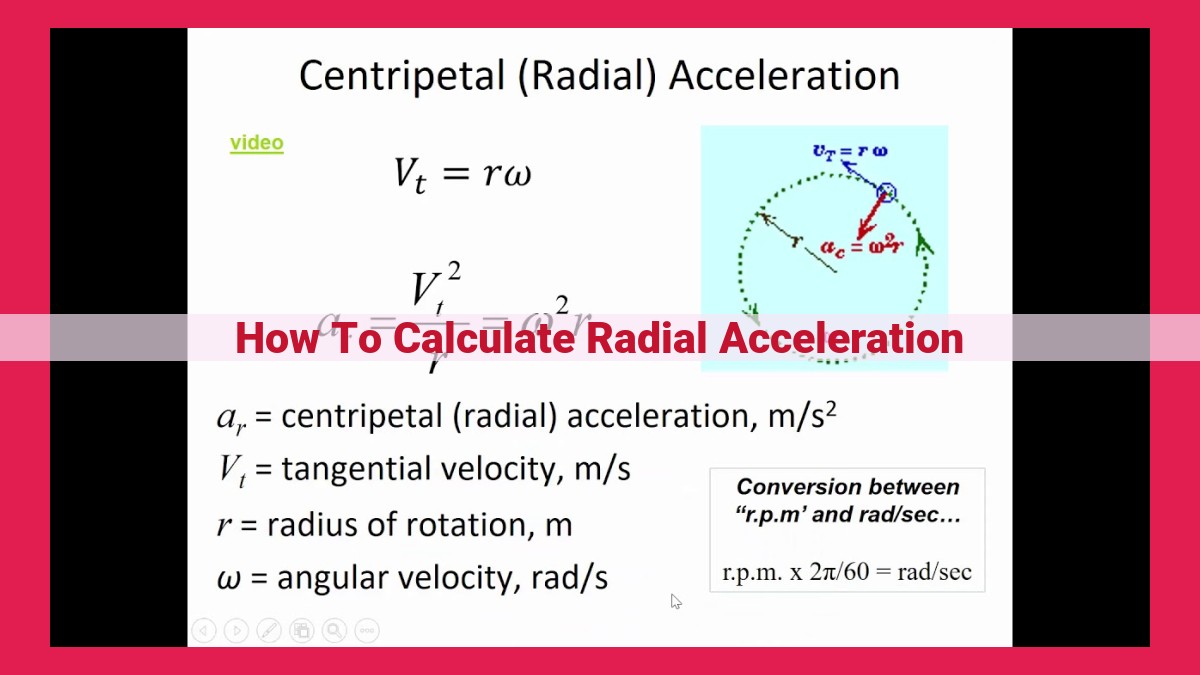
To calculate radial acceleration, determine the centripetal force maintaining circular motion. Then, use the equation a = v^2/r, where ‘a’ is the radial acceleration, ‘v’ is the linear velocity, and ‘r’ is the radius of curvature. Find the radius of curvature using R = (v^2) / a or relate it to angular displacement, linear velocity, and angular velocity. Calculate angular velocity using ω = 2πf / T, where ‘ω’ is angular velocity, ‘f’ is frequency, and ‘T’ is time. Determine the time using formulae relating time, velocity, acceleration, and distance.
Understanding Centripetal Force:
- Definition of centripetal force
- Its role in maintaining circular motion
- Describe its relationship with Newton’s Laws of Motion
- Explain how it counterbalances centrifugal force (if applicable)
Grasping the Essence of Centripetal Force: A Journey Through Circular Motion
Picture yourself whirling around on a merry-go-round, captivated by the mesmerizing dance of centripetal force. It’s the invisible hand that keeps you tethered to the whirling platform, preventing you from flying off into oblivion. But what exactly is this mysterious force, and how does it work its magic?
Centripetal force is the force that acts towards the center of a circular path, keeping an object moving along that path. It’s the opposite of centrifugal force, which is the outward force that an object experiences when it’s moving in a circle.
Newton’s Laws of Motion play a crucial role in understanding centripetal force. According to Newton’s second law, the force acting on an object is equal to its mass multiplied by its acceleration. In the case of circular motion, the centripetal force is what provides the necessary acceleration towards the center of the circle.
The relationship between force, velocity, and acceleration is depicted by Newton’s second law: F = ma. In circular motion, the acceleration is directed towards the center of the circle, so we call it centripetal acceleration.
To delve deeper, let’s consider an object moving in a circle with radius r and velocity v. The centripetal force required to keep the object in this circular path is given by:
Fc = mv^2 / r
This equation highlights the direct relationship between force, velocity, and radius. The faster the object moves or the smaller the radius of the circle, the greater the centripetal force required to keep it on track.
Interestingly, for every centripetal force, there’s a corresponding centrifugal force that appears to act outward from the center of the circle. However, centrifugal force is not a real force but rather a consequence of our rotating frame of reference. In essence, centripetal force counterbalances centrifugal force, ensuring the object’s stable circular motion.
Unveiling the Secrets of Circular Motion: Exploring the Radius of Curvature
Imagine yourself driving on a winding road, your car smoothly navigating the curves. Behind the scenes, a fascinating force is at play, keeping you securely on the road – centripetal force. It’s this force that pulls your car towards the center of the curve, preventing you from flying off into the distance.
Radius of Curvature: A Measure of Circularity
The radius of curvature is a crucial concept in understanding circular motion. Imagine a circle – the radius of curvature is the distance from any point on the circle to its center. In the case of your car on the winding road, the radius of curvature is the distance from your car to the center of the curve you’re driving around.
The Formula for Radius of Curvature
Scientists have devised a formula to calculate the radius of curvature:
R = (v^2) / a
where:
- R is the radius of curvature
- v is the linear velocity, or the speed at which an object is moving in a circular path
- a is the centripetal acceleration, or the acceleration towards the center of the circle
Linear Velocity and Angular Velocity
Linear velocity measures how fast an object is moving along a straight line, while angular velocity measures how quickly an object is rotating or moving in a circular path. These two quantities are intimately related to the radius of curvature. As the linear velocity of an object increases, the radius of curvature must also increase to maintain a constant centripetal acceleration.
Angular Displacement and Period
Angular displacement is the angle through which an object rotates, while period is the time it takes for an object to complete one full rotation. Angular velocity, linear velocity, and radius of curvature are interconnected through the following formula:
ω = 2πf / T
where:
- ω is the angular velocity
- f is the frequency of rotation
- T is the period of rotation
Velocity: The Key to Understanding Motion
Imagine driving your car around a circular track. As you navigate the curves, you feel a force pulling you towards the center of the track. This force is called centripetal force. It keeps you from flying off the track and keeps you moving in a circular path.
The radius of curvature, or the tightness of the curve, plays a crucial role in determining the centripetal force required. The formula R = (v^2) / a shows that as speed increases or the curve becomes tighter, the centripetal force must increase to maintain the circular motion.
Velocity is a fundamental concept in physics. It’s defined as the rate of change of displacement over time. It’s different from speed, which is the rate at which distance is covered. Velocity also has two components: speed and acceleration. Speed is the magnitude of velocity, while acceleration is the rate at which velocity changes.
Understanding velocity is essential in circular motion. For instance, when a cyclist pedals around a circular track, their linear velocity is constantly changing, even though their speed may remain relatively constant. This change in velocity is due to the cyclist’s angular velocity, or the rate at which they turn around the center of the track.
Calculating angular velocity is important for understanding rotational motion. The formula ω = 2πf / T shows that angular velocity is directly proportional to frequency (the number of rotations per second) and inversely proportional to period (the time it takes to complete one rotation).
Time plays a crucial role in circular motion. It can be calculated using various formulae involving velocity, acceleration, and distance. For example, if you know the initial and final velocities of an object moving in a circle, you can use the formula t = (v_f – v_i) / a to determine the time it takes to complete a rotation.
In summary, understanding velocity and its significance is essential for comprehending circular motion and the forces involved. It allows us to analyze how an object moves along a circular path, calculate time, and comprehend the role of centripetal force in maintaining the object’s circular trajectory.
Calculating Angular Velocity: A Guide to Unveiling Rotational Motion
In the mesmerizing world of physics, understanding motion in a circle is essential. One key component of this is angular velocity, which measures how quickly an object rotates around a fixed point. Let’s delve into the fascinating realm of angular velocity and discover how it’s calculated.
Defining Angular Velocity: The Rate of Rotation
Imagine a spinning top or a merry-go-round whirling around. Angular velocity is a measure of how fast these objects rotate. It’s defined as the rate of change of angular displacement, which is the angle through which an object turns. This measure is typically expressed in radians per second (rad/s).
Formula for Angular Velocity: Combining Frequency and Period
To calculate angular velocity, a simple formula comes to our aid: (ω = 2πf / T). Here, ω represents the angular velocity, f denotes the frequency of rotation (in revolutions per second), and T stands for the period of rotation (in seconds). The relationship between frequency and period is inversely proportional, meaning a higher frequency corresponds to a shorter period and vice versa.
Interplay with Rotational Motion and Period: The Dance of Time and Rotation
Angular velocity plays a crucial role in rotational motion. It quantifies the rate at which an object turns. The period of rotation, on the other hand, measures the time it takes for one complete revolution. These two quantities are closely intertwined, with a higher angular velocity resulting in a shorter period of rotation.
Determining Time in Circular Motion: A Journey Through Time and Space
Time, an abstract and versatile concept, plays a crucial role in unraveling the mysteries of circular motion. In this realm of whirling objects, time weaves its intricate tapestry, connecting velocity, acceleration, and distance in a dance of motion.
To embark on this time-traveling adventure, let us first consider velocity, a measure of how swiftly an object traverses a path. In circular motion, velocity manifests as a combination of two components: speed, the rate at which distance is covered, and direction, which constantly changes as the object navigates its circular trajectory.
Next, we encounter acceleration, the rate of change in velocity. Acceleration manifests as a force that either propels the object toward the center of the circle or draws it away. In circular motion, acceleration is centripetal, meaning it relentlessly pulls the object inward, ensuring it remains on its curved path.
Distance, the length of the path traversed, joins the trio of time, velocity, and acceleration. Time acts as the conductor, orchestrating the interplay between these elements. By manipulating time, we can unravel the secrets of circular motion, determining the duration it takes for an object to complete a revolution or traverse a specific arc.
Time’s influence extends to a myriad of scenarios in circular motion. Suppose a skater glides gracefully across an icy rink, completing graceful pirouettes. Time can reveal the precise moment when their angular velocity reaches its peak, capturing the fleeting beauty of their performance. Or consider a rotating wheel, its spokes blurring as it spins faster and faster. Time provides the key to unlocking the wheel’s rotational period, the time it takes to complete a single revolution.
Understanding time’s role in circular motion is a transformative experience, empowering us to decode the intricate movements of the celestial bodies, from the rhythmic dance of planets orbiting their stars to the mesmerizing spin of galaxies. Time becomes a window into the dynamic symphony of our cosmos, a tool for unraveling the mysteries that lie hidden within the ebb and flow of circular motion.