Unveiling The Maclaurin Series: Essential Tool For Approximating Complex Functions
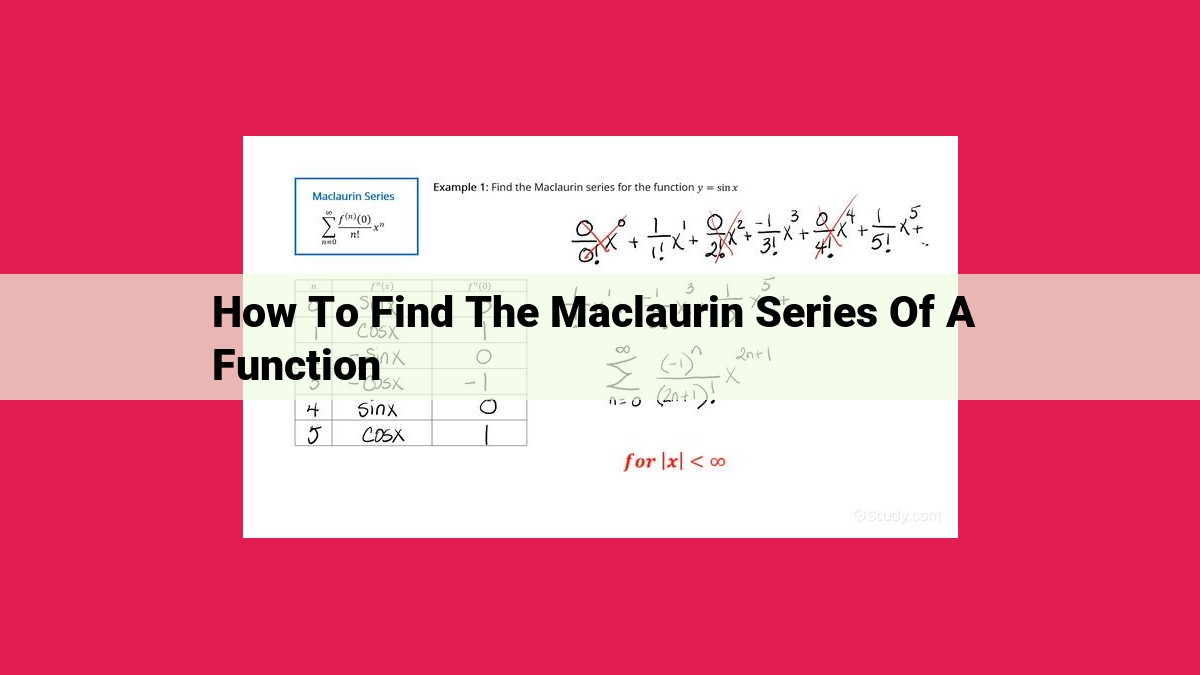
The Maclaurin series is a representation of a function as an infinite sum of terms involving its derivatives. It enables approximating complex functions as polynomials, facilitating their analysis and applications in various fields such as mathematics, physics, finance, and economics. To find the Maclaurin series of a function, one can use mathematical tools like the formula for the nth derivative, which provides a recursive relationship to compute coefficients in the series. Calculators and mathematical software simplify this process, but understanding the concept thoroughly allows for manual calculations and deeper insights.
Unveiling the Maclaurin Series: A Gateway to Understanding Functions
In the realm of mathematics, the Maclaurin series stands as a cornerstone, providing a profound understanding of the nature of functions. Let’s embark on a journey to unravel its secrets, making it an accessible ally for unlocking the mysteries of the mathematical world.
The Essence of the Maclaurin Series
The Maclaurin series is an infinite sum that approximates a function by representing it as a polynomial. It is a special case of the Taylor series, where the expansion takes place around the point (x=0). The Maclaurin series acts as a powerful tool for understanding the behavior of functions, as it allows us to approximate them locally using familiar polynomial functions.
Relationship to the Taylor Series
The Maclaurin series is an intimate relative of the Taylor series. Both series represent functions as infinite sums of polynomial terms. However, the Taylor series expands the function around any point, while the Maclaurin series is specifically centered at the origin. This means that the Maclaurin series is a particularly convenient choice when examining functions near (x=0).
By comprehending the Maclaurin series, we gain a deep appreciation for the behavior of functions near specific points. It empowers us to make informed approximations, paving the way for problem-solving in various fields. From physics to engineering and beyond, the Maclaurin series is a vital tool in the arsenal of those seeking a deeper understanding of the mathematical world.
Key Concepts for Understanding Functions
In the realm of mathematics, functions are ubiquitous, serving as building blocks for describing a wide array of phenomena. Understanding their core concepts is crucial for deciphering the language of the universe.
The Automobile: A Symphony of Motion
Imagine a sleek automobile gliding effortlessly through the streets. Its movement is a testament to the power of functions. Each component, from the engine’s combustion to the transmission’s gear ratios, contributes to the vehicle’s motion. By meticulously defining the relationship between the input variables (e.g., gas pedal position) and the output (e.g., velocity), functions provide the blueprint for the automobile’s performance.
The Computer: A Gateway to Knowledge
The modern computer is an embodiment of function theory. It processes information by executing a series of instructions, transforming input data into meaningful output. At the heart of this process lie functions that describe the operations performed on the data. These functions enable us to effortlessly create documents, surf the web, and unlock countless realms of knowledge.
The Book: A Tapestry of Ideas
A book is a vessel of wisdom, where the written word weaves a tapestry of ideas. Each page contains functions that map the author’s thoughts to a reader’s understanding. The chapters, sections, and paragraphs are carefully structured, allowing the reader to navigate effortlessly through the complexities of the subject matter. Without functions, the book would be a mere collection of incoherent words, devoid of meaning.
Food: The Elixir of Life
Nourishment sustains our bodies and minds, and functions play a vital role in this process. The nutritional value of food is determined by a complex interplay of chemical reactions described by functions. These functions help us determine the energy content of our meals and the optimal proportions of macronutrients for a balanced diet.
Music: The Universal Language of Emotion
Music transcends linguistic barriers, evoking emotions and connecting hearts. The intricate patterns of sound waves are functions that map time to the perception of harmony and melody. These functions orchestrate the musical tapestry, creating a symphony that resonates deep within our souls.
In summary, the concepts of functions provide the foundation for understanding the world around us. From the practical applications in automobiles and computers to the expressive realm of art and literature, functions are the language through which we decipher the complexities of our universe. By mastering these concepts, we unlock a door to a world of knowledge and wonder.
Applications of the Maclaurin Series: Empowering Real-World Solutions
The Maclaurin series, a potent mathematical tool, unveils the hidden patterns within complex functions. It opens up a realm of possibilities, transforming abstract equations into practical solutions for everyday challenges. Let’s explore some fascinating applications that demonstrate its versatility.
Engineering Marvels: From Bridges to Rockets
In the world of engineering, the Maclaurin series plays a crucial role in designing structures that can withstand immense forces. Engineers use it to calculate the deformation of complex shapes like bridges and aircraft wings. By approximating these shapes using polynomials, they can predict their behavior under various loads, ensuring safety and structural integrity.
Unveiling the Secrets of Cosmology
The vastness of the cosmos presents mysteries that the Maclaurin series helps unravel. Astronomers use it to approximate the temperature distribution within stars, allowing them to understand their evolution and energy sources. Moreover, it aids in the analysis of planetary orbits and the intricate dance of celestial bodies.
Optimizing Business Strategies
In the realm of business, the Maclaurin series empowers companies to forecast demand and optimize inventory levels. By modeling demand patterns as polynomial functions, they can minimize waste and maximize profits. Additionally, it enables predictive analytics, allowing businesses to anticipate market trends and make informed decisions.
Enhancing Medical Diagnostics
Within the medical field, the Maclaurin series contributes to improving diagnostic techniques. It aids in the analysis of medical images and the development of prediction models. By approximating complex biological processes using polynomials, healthcare professionals can diagnose diseases early and prescribe targeted treatments.
Harnessing the Power of Computing
The world of computing also embraces the Maclaurin series to solve complex numerical problems. It enables the approximation of functions and the derivation of mathematical expressions. Engineers use it to optimize signal processing algorithms and enhance the performance of computer graphics.
These are just a glimpse of the countless applications of the Maclaurin series. From engineering marvels to groundbreaking scientific discoveries, it empowers us to understand and shape our world in remarkable ways. As we delve deeper into its potential, we unlock a treasure trove of solutions that transform the abstract into the extraordinary.
Step-by-Step Guide to Conquer the Maclaurin Series Manually
Are you ready to embark on a mathematical adventure without the safety net of a calculator? Fear not! This step-by-step guide will empower you to conquer the Maclaurin series manually, like a true mathematical warrior.
Unveiling the Maclaurin Mystery
At its core, the Maclaurin series is a powerful technique that allows us to represent complex functions as an infinite sum of simpler terms. This series is named after Colin Maclaurin, a brilliant mathematician who lived in the 18th century.
Step 1: Prepare Your Toolkit
To delve into the Maclaurin series, we’ll need the indispensable Taylor’s theorem. This theorem provides the foundation for expressing functions as power series around a specific point. Remember, the Maclaurin series is a special case of Taylor’s theorem where we expand the function around x = 0.
Step 2: Craft Your Series
The Maclaurin series takes the form:
f(x) = f(0) + f'(0)x + (f''(0)/2!)x^2 + (f'''(0)/3!)x^3 + ...
where:
- f(x) is the function we want to represent as a power series
- f(0) is the function evaluated at x = 0
- f'(0) is the first derivative of f(x) evaluated at x = 0
- f”(0) is the second derivative of f(x) evaluated at x = 0
- … and so on
Step 3: Dive into Derivatives
To calculate the Maclaurin series, we need to evaluate the function and its derivatives at x = 0. This can be a bit tricky, especially for complex functions. Don’t worry; we’ll tackle strategies for handling these challenges later.
Step 4: Conquer Complex Functions
For functions with intricate derivatives, we can employ clever tricks to simplify the process. One technique involves using recursion, where we express higher-order derivatives in terms of lower-order ones. Another approach is using generating functions, which offer an elegant way to represent a sequence of coefficients in the series.
With perseverance and a touch of ingenuity, you’ll master the art of calculating the Maclaurin series manually. This skill will not only deepen your mathematical understanding but also equip you with a valuable tool for tackling real-world problems.
Using Calculators and Software for Maclaurin Series
While manual calculation provides a deep understanding, calculators and mathematical software offer convenience and efficiency when finding Maclaurin series.
Calculators
Scientific calculators include built-in functions for Maclaurin series calculation. Simply input the function and specify the order of the series. The calculator will display the coefficients and the remainder term.
Mathematical Software
Specialized mathematical software, such as Mathematica, Maple, and MATLAB, provides robust tools for Maclaurin series manipulation. These programs allow you to:
- Generate series for complex functions
- Manipulate coefficients and terms
- Graph the series and its approximations
- Explore convergence and error estimation
Limitations and Best Practices
Using calculators and software has its limitations:
- Accuracy: Results may be approximate, especially for complex functions.
- Convergence: Software may not always correctly determine whether the series converges.
- Interpretation: It’s essential to interpret the results carefully and understand the limitations of the approximation.
Calculators and software can streamline the process of finding Maclaurin series, making it more accessible to a wider range of users. However, it’s important to be aware of the limitations and use these tools cautiously, always verifying the accuracy of the results.
Advanced Concepts in the Maclaurin Series
Convergence and Error Estimation
The Maclaurin series is an infinite series representation of a function. It’s crucial to determine when the series converges to the original function. Convergence tests, such as the ratio and root tests, help establish the range of values for which the series approximates the function accurately.
Error estimation techniques, like Lagrange’s remainder, quantify the discrepancy between the series and the function within a given interval. This knowledge aids in determining the number of terms required for a desired level of precision.
Technical Applications
The Maclaurin series finds wide-ranging applications in scientific and engineering fields. For instance, it’s employed in:
- Numerical analysis: Approximating integrals, derivatives, and solutions to differential equations.
- Differential equations: Finding power series solutions to differential equations, enabling the analysis of complex systems.
- Numerical optimization: Identifying critical points and minima or maxima of functions.
- Probability theory: Calculating probabilities and quantiles in complex distributions.
By understanding these advanced concepts, readers gain a deeper appreciation for the power and versatility of the Maclaurin series. It empowers them to tackle more complex problems and make informed decisions about the series’ applicability and accuracy.



