Antiderivative Techniques: Rule Of Quotients And Partial Fractions For Rational Functions

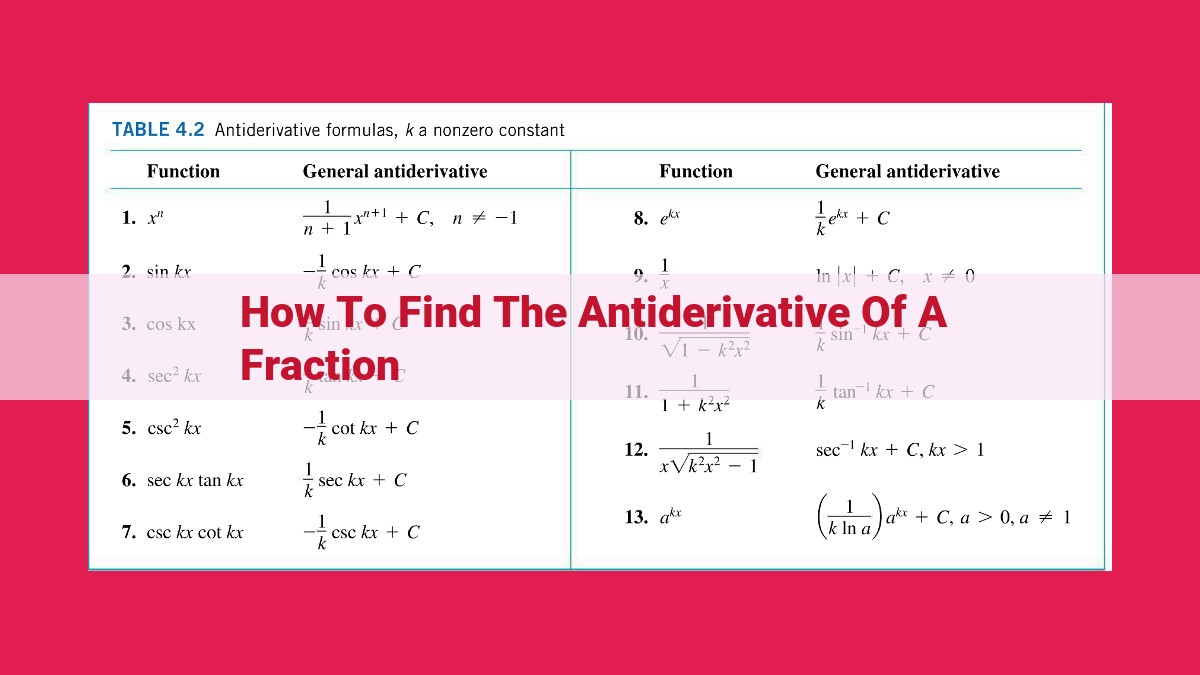
Finding the antiderivative of a fraction involves the Rule of Quotients for algebraic fractions and Partial Fractions Decomposition for proper rational functions. The Rule of Quotients provides a direct formula for integrating fractions by dividing their derivatives and antiderivatives. Partial Fractions Decomposition breaks down complex fractions into simpler ones, allowing for easier integration. These techniques enable the calculation of antiderivatives, crucial in areas like physics and engineering where complex functions and their rates of change are analyzed.

Finding Antiderivatives of Fractions: A Journey Through Calculus

In the realm of calculus, antiderivatives play a crucial role. They enable us to recover the original function when the derivative is applied. Among these functions, fractions pose unique challenges, requiring specialized techniques to find their antiderivatives.

Fractions emerge in various mathematical contexts, such as velocity in physics or instantaneous rate of change in economics. Finding their antiderivatives empowers us to solve problems related to area under curves or accumulation over time. Therefore, mastering the art of integrating fractions is essential for unlocking the full potential of calculus.

In this comprehensive guide, we will embark on a journey to explore two treasured methods for finding antiderivatives of fractions: the Rule of Quotients and Partial Fractions Decomposition. We’ll delve into each technique, uncovering its secrets and unraveling its practical applications.

The Rule of Quotients: Unlocking the Antiderivatives of Fractions

In the fascinating world of calculus, understanding the concept of antiderivatives is paramount for unlocking the secrets of functions and their behavior. Among the myriad of functions, fractions pose a unique challenge, demanding specialized techniques to derive their antiderivatives. One such technique is the Rule of Quotients, a powerful tool for conquering this mathematical enigma.

Essence of the Rule of Quotients

The Rule of Quotients provides a systematic formula for finding the antiderivative of a fraction, expressed as:

∫ (f(x)/g(x)) dx = (F(x)g(x) – f(x)G(x)) / g(x)^2

where:

• f(x) and g(x) are polynomials
• F(x) is the antiderivative of f(x)
• G(x) is the antiderivative of g(x)

Step-by-Step Guide to the Rule of Quotients

1. Factor the Denominator (g(x)) into Linear or Irreducible Quadratic Factors:

This step helps in identifying the appropriate integration technique.

2. Decompose the Fraction into Partial Fractions (If Necessary):

For proper rational functions where g(x) cannot be factored further, partial fraction decomposition may be required to simplify the integration process.

3. Integrate Each Partial Fraction Separately:

Using the power rule of integration, each partial fraction is integrated to obtain its antiderivative.

4. Substitute the Antiderivatives and Simplify:

Replace F(x) and G(x) in the Rule of Quotients formula with the antiderivatives obtained in step 3 and simplify the expression to get the final antiderivative.

Example: Applying the Rule of Quotients

Let’s find the antiderivative of f(x) = (2x^2 + 1) / (x – 1).

• Factor the denominator: x – 1
• Integrate using the power rule: F(x) = (2/3)x^3 + x + C
• G(x) = ∫1/(x – 1) dx = ln|x – 1| + C
• Substitute into the Rule of Quotients formula:

∫ (2x^2 + 1) / (x - 1) dx = (2/3)x^3 + x + C - (2x^2 + 1)ln|x - 1| + C

The Rule of Quotients empowers us to tackle the daunting task of finding antiderivatives of fractions, opening doors to solving complex calculus problems. Whether it’s understanding the dynamics of functions or delving into real-world applications, the Rule of Quotients is an indispensable tool in the calculus arsenal.

Partial Fractions Decomposition: A Technique to Conquer Antiderivatives of Fractions

In the realm of calculus, finding the antiderivatives of fractions is like navigating a treacherous terrain. That’s where partial fractions decomposition comes to the rescue, a technique that transforms complex fractions into a collection of simpler ones.

Consider a proper rational function, where the degree of the numerator is less than the degree of the denominator. These functions can be challenging to integrate directly, but partial fractions decomposition breaks them down into their constituent parts.

The first step is to factor the denominator into linear or irreducible quadratic factors. These factors will provide the denominators of the decomposed fractions.

Next, we decompose the fraction into a sum of simpler fractions. Each fraction has a different linear factor or irreducible quadratic factor in its denominator. The numerators of these fractions are determined by solving a system of equations.

Finally, we find the antiderivatives of each fraction. This involves using integration techniques like substitution or integration by parts.

By combining the antiderivatives of the decomposed fractions, we obtain the overall antiderivative of the original fraction. This powerful technique allows us to tackle integrals that would otherwise seem insurmountable.

Example:

Consider the fraction:

f(x) = 1 / (x^2 - 1)

• Factor the denominator: x^2 – 1 = (x + 1)(x – 1)
• Decompose the fraction: 1 / (x^2 – 1) = A / (x + 1) + B / (x – 1)
• Solve for A and B: Solving the system of equations gives A = 1/2 and B = -1/2
• Find the antiderivatives: Antiderivative of A / (x + 1) = ln(|x + 1|)
  Antiderivative of B / (x – 1) = -ln(|x – 1|)
• Combine the antiderivatives: Overall antiderivative = 1/2 * ln(|x + 1|) – 1/2 * ln(|x – 1|)

Through partial fractions decomposition, we’ve turned a challenging integral into a manageable sum of simpler ones, demonstrating the power of this technique in finding antiderivatives of fractions.

Finding Antiderivatives of Fractions: A Comprehensive Guide

In the realm of calculus, antiderivatives play a crucial role in understanding the behavior of functions. Among the various functions we encounter, fractions pose a unique challenge, requiring specialized techniques to find their antiderivatives. This guide will delve into the Rule of Quotients and Partial Fractions Decomposition, two powerful methods for tackling this challenge.

Rule of Quotients

The Rule of Quotients provides a direct formula for finding the antiderivative of a fraction:

∫(u/v)dx = (log |v|)u - ∫u * v' dx / v^2

where u and v are differentiable functions and v ≠ 0. To apply this rule, we employ the following steps:

• Factor the denominator v into its irreducible factors.
• Divide the numerator u by the factored denominator v, expressing the result as a quotient and remainder.
• Integrate the quotient and multiply the result by the remainder.
• Subtract the integral of u times the derivative of v divided by v squared.

Partial Fractions Decomposition

For more complex fractions, known as proper rational functions, Partial Fractions Decomposition offers a powerful approach. A proper rational function can be expressed as:

f(x) = (p(x)/q(x))

where p(x) is a polynomial of degree less than q(x). Partial fractions decomposition involves expressing f(x) as a sum of simpler fractions that can be easily integrated. The steps involved are:

• Factor the denominator q(x) into linear and irreducible quadratic factors.
• For each linear factor (ax + b), create a partial fraction of the form A/(ax + b).
• For each irreducible quadratic factor (ax^2 + bx + c), create a partial fraction of the form B(x + m)/(ax^2 + bx + c) or C/(ax^2 + bx + c), where m is a constant.
• Solve for the coefficients A, B, C using a system of equations.
• Integrate each partial fraction.

Examples

Using the Rule of Quotients:

Consider the fraction (x^2 + 1)/(x – 1).

• Factoring the denominator: x – 1
• Division: x^2 + 1 = (x – 1)(x + 1) + 2
• Applying the formula: ∫(x^2 + 1)/(x – 1)dx = (log |x – 1|)(x + 1) – ∫(x + 1) * (1) dx / (x – 1)^2

Using Partial Fractions Decomposition:

Consider the fraction (x^3 – 1)/(x^2 + 1).

• Factoring the denominator: x^2 + 1 = (x + i)(x – i)
• Partial fraction decomposition: (x^3 – 1)/(x^2 + 1) = (x + A)/(x + i) + (x + B)/(x – i)
• Solving for A and B: A = -1, B = 1
• Integration: ∫(x^3 – 1)/(x^2 + 1)dx = ∫(-1/(x + i) + 1/(x – i))dx

Applications: Antiderivatives of Fractions in the Real World

In the realm of calculus, finding antiderivatives of fractions plays a crucial role in unlocking a myriad of applications in various fields beyond the confines of mathematical theorems.

Physics: The Dance of Forces

In the symphony of forces that govern our universe, physicists rely on antiderivatives to unravel the intricate dynamics of motion. By integrating fractions that represent acceleration or velocity, they can unveil the secrets of projectiles soaring through the air, satellites orbiting celestial bodies, and the harmonic oscillations of springs.

Engineering: Bridges, Buildings, and Beyond

Engineers wield the power of antiderivatives to design structures that withstand the relentless forces of nature and human activity. They employ these techniques to calculate stress, strain, and bending moments in bridges, buildings, and aircraft. By integrating fractions that describe load distributions, they ensure the integrity of these vital structures, safeguarding lives and livelihoods.

Beyond Science: Exploring Real-World Phenomena

The applications of antiderivatives extend far beyond the realms of physics and engineering. Economists use them to model the change in supply and demand over time. Ecologists employ them to analyze the growth rates of populations. Even sociologists harness their power to decipher the ebb and flow of social trends.

In essence, finding antiderivatives of fractions serves as a versatile tool, empowering us to unravel the complexities of the world around us. By mastering these techniques, we unlock the ability to solve real-world problems, optimize designs, and gain a deeper understanding of the interplay between mathematics and the physical, biological, and social sciences.