Angle Bisector: Dividing Angles And Its Applications In Geometry
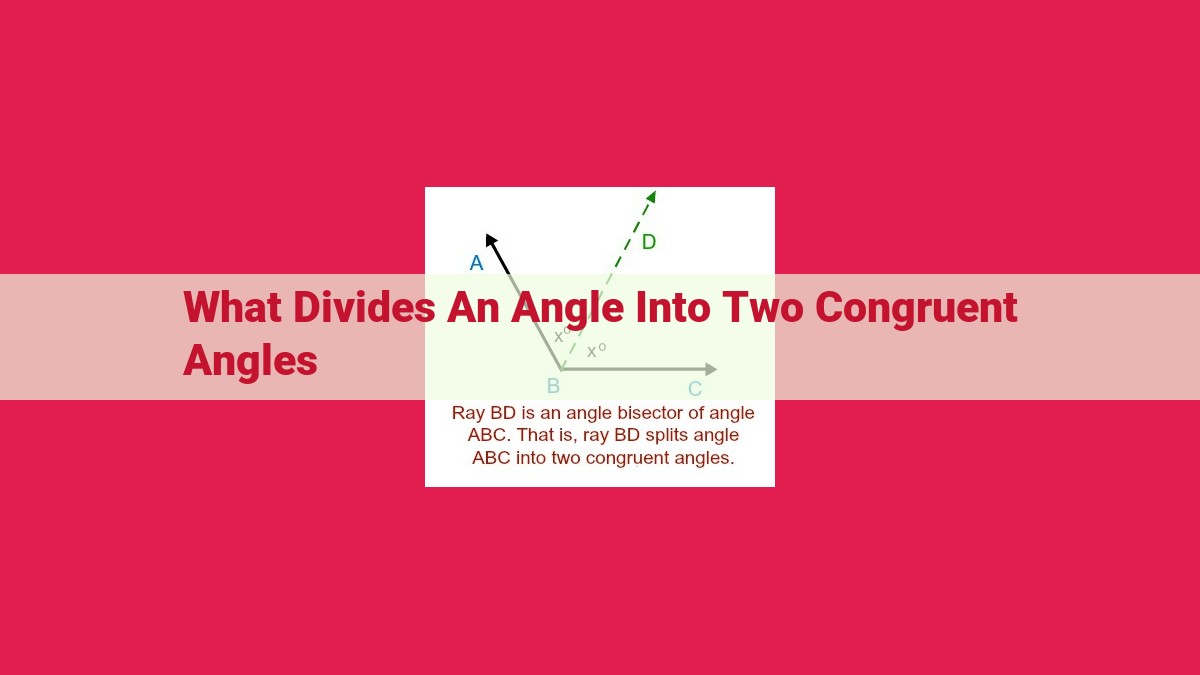
An angle bisector is a line or ray that divides an angle into two congruent angles. It originates from the vertex and bisects the angle, creating two equal parts. Angle bisectors have significant applications in geometry, including proving triangle congruence through the angle bisector theorem, trisecting angles through repeated bisections, and defining circles through perpendicular bisectors. Moreover, right bisectors intersect line segments at their midpoints, aiding in finding the shortest distance from a point to a line segment.
Angle Bisectors: The Secret to Dividing Angles with Precision
In the world of geometry, angles are everything. From triangles to circles, the angles between lines and curves play a crucial role in determining shapes and sizes. And when it comes to dividing angles precisely, there’s no better tool than an angle bisector.
An angle bisector is a line or ray that divides an angle into two equal parts. Imagine a pizza slice. The line from the tip of the slice to the center of the pizza is like an angle bisector, splitting the slice into two identical halves.
Angle Bisectors and Triangles
Angle bisectors play a vital role in triangle congruence. According to the Angle Bisector Theorem, if an angle bisector of a triangle is drawn to the opposite side, then the segments of that side adjacent to the bisector are congruent.
For instance, let’s say you have a triangle ABC with angle bisector AD. If AD is perpendicular to side BC, then segments BD and DC are equal in length. This powerful theorem helps us prove that triangles are congruent even when they don’t appear to be the same shape.
Angle Trisection: The Art of Dividing into Three
If you need to divide an angle into three equal parts, angle bisectors are your secret weapon. By repeatedly bisecting the angles formed by the initial bisections, you can create three congruent angles.
Imagine a pie cut into four equal slices. Each slice represents one-fourth of the pie. Now, if you cut each slice in half again, you’ll end up with eight equal slices, or one-eighth of the pie. By continuing this process, you can divide the pie into as many equal parts as you need.
Perpendicular Bisectors: Defining Circles
Perpendicular bisectors are lines or rays that pass through the midpoint of a line segment and are perpendicular to it. These bisectors create a circle that passes through the endpoints of the line segment.
Think of a compass. When you set the compass points on the endpoints of a line segment and draw a circle, you’re essentially creating a perpendicular bisector. The resulting circle has its center on the perpendicular bisector and passes through both endpoints of the line segment.
Right Bisectors: Distance from a Point to a Line
Right bisectors are lines or rays that are perpendicular to a line segment and pass through its midpoint. These bisectors are crucial for finding the shortest distance from a point to a line segment.
Imagine a tower located some distance away from a straight road. The shortest path from the tower to the road is a line perpendicular to the road from the tower. This line is the right bisector of the segment connecting the tower to the point where the line crosses the road.
Angle bisectors, perpendicular bisectors, and right bisectors are powerful tools in the geometer’s toolbox. They help us divide angles into equal parts, find congruent triangles, define circles, and determine distances. By understanding these bisectors, we unlock a deeper understanding of geometry and its applications in our world.
Triangle Congruence and Angle Bisectors
In the realm of geometry, angle bisectors hold a pivotal role in establishing the equality of triangles, a fundamental concept that underpins numerous mathematical proofs. The angle bisector theorem emerges as a guiding principle in this domain, empowering us to navigate the intricacies of triangle congruence with precision and elegance.
Unveiling the Essence of Angle Bisectors
An angle bisector, as its name suggests, is a line segment that originates from an angle’s vertex and intersects the opposite side at a right angle. Its primary mission is to divide an angle into two congruent halves, forming two new angles that are equal in measure. This unique property of angle bisectors bestows upon them an extraordinary ability to act as mediators in the world of triangles.
Angle Bisector Theorem: A Gateway to Triangle Congruence
The angle bisector theorem stands as a cornerstone of triangle congruence, providing a powerful tool for proving the equality of two triangles. According to this theorem, if an angle bisector of a triangle intersects the opposite side at a point, then it divides the opposite side into two segments that are proportional to the lengths of the adjacent sides.
Illustration Through Example
Consider triangle ABC, where angle bisector AD intersects side BC at point D. According to the angle bisector theorem, we have:
BD/DC = AB/AC
This remarkable relationship tells us that the ratio of BD to DC is equal to the ratio of AB to AC. In other words, the lengths of BD and DC are proportional to the lengths of AB and AC, respectively.
Significance in Triangle Congruence
The angle bisector theorem plays a pivotal role in proving triangle congruence using the Side-Angle-Side (SAS) theorem. It provides a shortcut for establishing the equality of two triangles by demonstrating that the ratios of corresponding sides are equal. This insight significantly simplifies the process of proving triangle congruence and opens up a realm of possibilities for solving geometric problems.
Angle bisectors, with their capacity to divide angles equally, serve as invaluable tools in the realm of geometry. They unlock the secrets of triangle congruence, enabling us to establish the equality of triangles effortlessly. The angle bisector theorem stands as a beacon, guiding us through the complexities of geometry with its clarity and elegance.
Angle Trisection Using Angle Bisectors: Unlocking the Secrets of Geometry
In the realm of geometry, there lies a fascinating challenge known as angle trisection: the task of dividing an angle into three equal parts. While this may seem like a simple undertaking at first glance, it’s a problem that has puzzled mathematicians for centuries and has no known precise solution using a compass and straightedge alone.
But fear not, for angle bisectors, those handy geometric tools, come to our aid. By harnessing their power, we can approximate the trisection of an angle with remarkable precision.
The process is remarkably elegant. We begin by bisecting the original angle, creating two congruent angles. Next, we bisect one of those new angles again, resulting in four angles that are one-fourth the size of the original. And by continuing this process of repeated bisection, we can create angles that are increasingly smaller, approaching one-third of the original angle.
In essence, this iterative approach transforms the seemingly impossible task of angle trisection into a manageable approximation. And while the result may not be exact, it can be arbitrarily close to the desired trisection.
So, while Euclid’s dream of trisecting an angle with just a compass and straightedge may forever elude us, the ingenuity of angle bisectors offers us a practical and reliable method to achieve the essence of this geometric feat.
**Perpendicular Bisectors: Unifying Locus of Points and Circle Definitions**
Meet perpendicular bisectors, the geometry superstars that bring together locus of points and the enigmatic world of circles. A perpendicular bisector is a line perpendicular to a line segment at its midpoint. It has a special relationship with the points that lie on it: they’re all equidistant from the endpoints of the line segment.
This intriguing property makes perpendicular bisectors the gatekeepers to a fascinating geometrical construct—locus of points. Remember that sneaky ruler you used to draw perfect circles? It wasn’t a magic trick; it was the manifestation of a perpendicular bisector!
Circles and perpendicular bisectors are kindred spirits, sharing a deep bond. When you draw a circle with a given line segment as its diameter, you’ll notice that the center of the circle lies on the perpendicular bisector of the line segment. This harmonious connection makes perpendicular bisectors essential for defining and constructing circles.
To find the perpendicular bisector of a line segment, follow this clever trick: construct two circles with each endpoint as its center and a radius greater than half the length of the line segment. The two circles will intersect at two points. The line connecting these intersection points is your perpendicular bisector!
Perpendicular bisectors are not just theorem-proving tools; they also have practical applications. For instance, if you’re trying to find the shortest distance from a point to a line segment, draw the perpendicular bisector of the line segment. Voila! The distance from the point to the perpendicular bisector is the coveted shortest distance.
Right Bisectors: Your Guide to Finding the Shortest Distance
In the realm of geometry, bisectors are like the peacemakers of the angle world. They have a knack for dividing angles and line segments into harmonious halves. One such bisector is the right bisector, a champion in determining the shortest distance from a point to a line segment.
Imagine you have a line segment, AB, and you want to find the point on the AB that is closest to an external point, P. This is where the right bisector steps in.
A right bisector is a line that intersects AB perpendicularly at its midpoint. This means it forms two right angles with AB. When you draw the right bisector of AB, it will intersect AB at a point we’ll call M.
Now, here comes the magic: the distance from P to M is the shortest possible distance from P to AB. It’s like a magical shortcut, guiding you to the closest point on AB without any detours.
How does this work? Well, let’s say you have another point on AB, N, that is not the midpoint. The right triangle formed by P, M, and N will always have a longer hypotenuse (PM) than the right triangle formed by P, M, and A or B. This is because the hypotenuse of a right triangle is always the longest side.
So, by finding the right bisector of AB and identifying the midpoint M, you have found the shortest distance from P to AB. It’s like a geometric GPS, leading you to the most efficient path.