Adiabatic Cooling: A Comprehensive Guide To Efficient Gas Cooling Without Heat Exchange
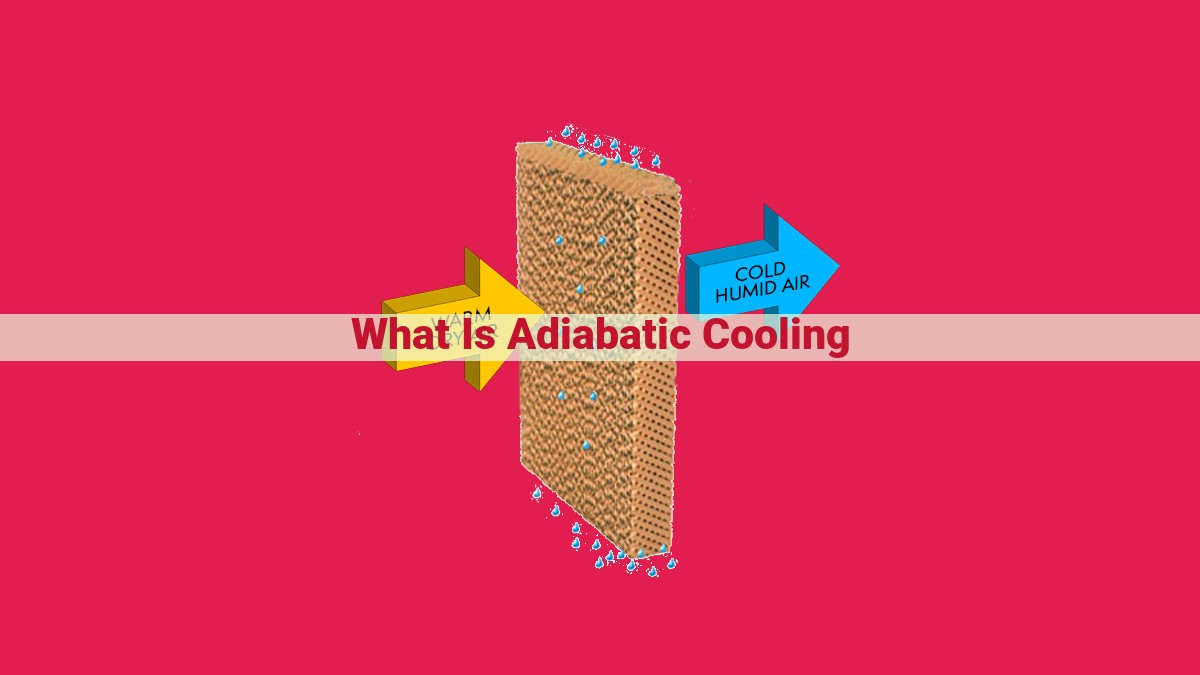
Adiabatic cooling is a process where gas cools without exchanging heat. It occurs when a gas is compressed or expanded, causing a change in its pressure and volume. This process is significant in thermodynamics, gas dynamics, and HVAC, as it affects gas behavior, flow properties, and cooling efficiency. Adiabatic cooling is an isentropic process, meaning entropy remains constant, and the specific heat ratio of the gas determines the temperature change during cooling. Applications include refrigeration, air conditioning, and energy conservation, offering potential for environmental sustainability.
Adiabatic Cooling: A Journey into Gas Dynamics and Thermodynamics
In the realm of energy transfer, there exists a captivating phenomenon known as adiabatic cooling. Simply put, it’s the art of cooling a gas without involving any heat exchange. This intricate process holds immense significance in fields such as thermodynamics, gas dynamics, and even HVAC (heating, ventilation, and air conditioning).
Delving into the Thermodynamics of Adiabatic Cooling:
Thermodynamics unveils the intricate dance of gas behavior, providing the blueprint for understanding adiabatic cooling. When a gas expands without any loss or gain of heat, its volume increases while its pressure decreases, leading to a temperature drop. This phenomenon is driven by the conservation of energy, where the energy lost due to expansion is converted into kinetic energy of the gas molecules.
Adiabatic Cooling in the Flow of Gas Dynamics:
Gas dynamics takes us on a journey into the fascinating world of gas motion. Here, adiabatic cooling plays a crucial role in shaping the flow and properties of gases. As a gas expands, its velocity increases, resulting in a further drop in temperature. This effect has profound implications in applications like supersonic nozzles and turbine blades.
Unveiling the Secrets of the Ideal Gas Law and Adiabatic Cooling:
The ideal gas law serves as a cornerstone in understanding gas behavior under adiabatic conditions. It provides a mathematical framework to calculate changes in pressure, volume, and temperature during adiabatic cooling. By harnessing this law, engineers and scientists can accurately predict the behavior of gases in various applications.
Adiabatic Cooling and the Enigmatic World of Isentropic Processes:
Isentropic processes embody the concept of constant entropy. They play a pivotal role in adiabatic cooling, as they represent the ideal scenario where there is no heat transfer between the gas and its surroundings. Understanding the connection between adiabatic cooling and isentropic processes is paramount in unraveling the intricacies of gas behavior.
The Significance of Specific Heat Ratio in Adiabatic Cooling Equations:
The specific heat ratio measures a gas’s ability to absorb and release energy. It holds immense significance in adiabatic cooling equations, as it determines the extent to which a gas cools during expansion. By incorporating the specific heat ratio into calculations, researchers and engineers can precisely predict temperature changes under adiabatic conditions.
Practical Applications of Adiabatic Cooling:
Adiabatic cooling finds widespread applications in various domains. It forms the cornerstone of refrigeration, air conditioning, and energy conservation systems. Additionally, it has promising potential in environmental sustainability, offering energy-efficient cooling solutions that minimize greenhouse gas emissions.
Thermodynamics of Adiabatic Cooling
Adiabatic cooling, a fascinating phenomenon in thermodynamics, occurs when a gas cools without exchanging heat with its surroundings. This intriguing process encompasses a range of principles that govern the behavior of gases.
Let’s delve into the thermodynamic perspective that underpins adiabatic cooling. Thermodynamics meticulously details the behavior and changes that transpire within systems. One of its fundamental tenets is the conservation of energy. According to this law, energy cannot be created or destroyed but can transform from one form to another.
In the case of adiabatic cooling, as a gas expands or contracts, it does so without heat exchange. This means the energy lost by the expanding gas is not absorbed by the surroundings. Instead, it is converted into work. This work is manifested in the movement of the gas, resulting in a decrease in its temperature.
This interplay between energy conservation and gas movement forms the basis of adiabatic cooling. The gas loses energy and cools down as it expands, while the work done by the gas is converted into kinetic energy. This process aligns perfectly with the conservation of energy, showcasing the intricate interplay of thermodynamics in shaping the behavior of gases.
Gas Dynamics and Adiabatic Cooling
Gas Dynamics Unveiled
Gas dynamics, a captivating field of study, delves into the fascinating world of gas motion. By understanding the intricate dance of gases, we can unravel the secrets of complex phenomena like fluid flow, shock waves, and the behavior of gases in various environments.
Adiabatic Cooling: A Dynamic Force
Adiabatic cooling, a remarkable process in gas dynamics, occurs when a gas cools without exchanging heat with its surroundings. As a gas expands, it does work against external pressure. This work comes at the expense of the gas’s internal energy, resulting in a drop in temperature.
The Ripple Effect of Adiabatic Cooling
This cooling has profound effects on gas flow and properties. As the gas cools, its velocity increases, obeying the laws of conservation of energy. Consequently, the gas becomes denser, leading to changes in pressure and volume. Moreover, adiabatic cooling can influence a gas’s specific heat ratio, a measure of its energy capacity, which plays a crucial role in understanding its thermodynamic behavior.
Applications: Harnessing the Power of Adiabatic Cooling
Adiabatic cooling finds widespread applications across diverse industries. In refrigeration and air conditioning, it serves as a cost-effective and environmentally friendly cooling method. By harnessing the power of adiabatic cooling, we can reduce energy consumption and minimize greenhouse gas emissions. Additionally, adiabatic cooling has potential applications in energy conservation, such as in gas turbines and compressors, where it enhances efficiency and reduces operating costs.
Ideal Gas Law and Adiabatic Cooling
In the realm of thermodynamics, the ideal gas law reigns supreme as a fundamental principle describing the behavior of gases. This enigmatic equation encapsulates the relationship between four pivotal variables: pressure (P), volume (V), temperature (T), and the number of moles (n).
$$PV = nRT$$
Where R represents the universal gas constant.
The significance of the ideal gas law lies in its ability to predict the behavior of gases under diverse conditions. By manipulating its variables, we can unlock insights into a myriad of gas-related phenomena. One such phenomenon is adiabatic cooling.
Adiabatic Cooling and the Ideal Gas Law
Adiabatic cooling is a unique process that transpires without the exchange of heat between the system and its surroundings. Envision a gas trapped within a container that is insulated to prevent heat transfer. As the gas expands, it performs work on the container, causing its volume to increase. This expansion leads to a decrease in pressure and temperature. The absence of heat exchange is the hallmark of adiabatic cooling.
The ideal gas law plays a crucial role in unraveling the intricacies of adiabatic cooling. By applying this law to an adiabatic process, we can establish a relationship between the initial and final states of the gas:
$$\frac{P_1V_1}{T_1} = \frac{P_2V_2}{T_2}$$
This equation elucidates how the pressure, volume, and temperature of a gas vary during adiabatic cooling. By measuring these parameters, we can quantify the extent of cooling and discern the gas’s behavior under adiabatic conditions.
The ideal gas law empowers us to calculate changes in temperature during adiabatic processes. This calculation hinges on the gas’s specific heat ratio (γ), which quantifies the ratio of its specific heats at constant pressure and constant volume. Armed with this knowledge, we can determine the final temperature of the gas after undergoing adiabatic expansion:
$$T_2 = T_1(\frac{V_1}{V_2})^{\gamma-1}$$
The ideal gas law and adiabatic cooling are intertwined concepts that provide a comprehensive understanding of gas behavior in the absence of heat exchange. Their applications extend to refrigeration, air conditioning, and energy conservation, making them indispensable tools for engineers and scientists alike.
Isentropic Processes and Adiabatic Cooling
In the realm of thermodynamics, isentropic processes are akin to an enchanting dance where entropy, a measure of disorder, remains constant. Picture a gas flowing through an insulated tube, where no heat is exchanged with its surroundings. As the gas glides along, its pressure, volume, and temperature undergo a captivating transformation. Through this marvelous journey, the gas undergoes an adiabatic transformation, defined by the absence of heat exchange.
Imagine a magician performing a mesmerizing trick, where an adiabatic process unfolds before your very eyes. A gas is trapped in a sealed container, and as its volume expands, its pressure gracefully diminishes. Witnessing this spectacle, you might be inclined to question the gas’s fate. Did the gas somehow exchange heat with the magician’s hat?
Fear not, for the gas has merely embarked on an isentropic journey. As it expands, it performs work on its surroundings, converting its internal energy into kinetic energy. This conversion results in a decrease in temperature, effortlessly achieving the illusion of cooling without any external heat exchange.
In essence, isentropic processes provide a profound connection between adiabatic cooling and the intricate dance of thermodynamics. They unveil a realm where entropy remains unyielding, and gases transform their energy into motion, resulting in the enigmatic phenomenon we know as adiabatic cooling.
Specific Heat Ratio and Adiabatic Cooling
In the realm of thermodynamics, gas properties play a crucial role in understanding and manipulating gases. Adiabatic cooling, a fascinating process of cooling gases without heat exchange, hinges on the concept of the specific heat ratio. This ratio, denoted by the Greek letter gamma (γ), measures a gas’s energy capacity and unveils its significance in adiabatic cooling.
Significance of Specific Heat Ratio
The specific heat ratio is a dimensionless quantity defined as the ratio of a gas’s specific heat at constant pressure (Cp) to its specific heat at constant volume (Cv). It represents the ability of a gas to store energy. A higher specific heat ratio indicates that a gas can store more energy as internal energy, while a lower ratio implies that the gas prefers to release energy as external work.
Role in Adiabatic Cooling Equations
In adiabatic cooling, the specific heat ratio plays a pivotal role in determining the temperature change and pressure variation of the gas. Adiabatic cooling occurs when a gas expands or compresses without heat exchange. During this process, the gas’s internal energy is converted into work, resulting in a temperature drop or pressure increase.
The relationship between the specific heat ratio, temperature, and pressure in adiabatic cooling is given by the following equation:
T2 / T1 = (P2 / P1)^(γ - 1 / γ)
where:
- T1 and T2 are the initial and final temperatures
- P1 and P2 are the initial and final pressures
Applications in Engineering and Beyond
The specific heat ratio finds numerous applications in engineering and scientific fields, including:
- Designing efficient engines and turbines
- Predicting gas behavior in combustion processes
- Optimizing refrigeration and air conditioning systems
- Understanding atmospheric phenomena
The specific heat ratio is a fundamental property of gases that provides valuable insights into their behavior under adiabatic conditions. Its significance in adiabatic cooling equations highlights its importance in understanding and utilizing this process in various engineering and scientific applications. By comprehending the role of the specific heat ratio, engineers and scientists can harness the power of adiabatic cooling for a wide range of practical and innovative solutions.
Applications of Adiabatic Cooling: Unlocking Energy Efficiency and Environmental Sustainability
Beyond its theoretical underpinnings, adiabatic cooling finds widespread applications in various industries and holds immense potential for advancing environmental sustainability.
Refrigeration and Air Conditioning
Adiabatic cooling plays a crucial role in refrigeration systems. By compressing and expanding a refrigerant, adiabatic cooling helps achieve the necessary temperature drop to effectively remove heat from perishable goods. Similarly, in air conditioning systems, adiabatic cooling can lower the temperature of incoming air without consuming additional energy. By reducing the load on compressors, adiabatic cooling can significantly enhance the energy efficiency of these systems.
Energy Conservation
Adiabatic cooling offers a promising path towards energy conservation. In industrial processes involving the compression and expansion of gases, adiabatic cooling can be harnessed to recover waste heat. This recovered energy can then be utilized for other purposes, reducing overall energy consumption. Moreover, in data centers and other facilities with high cooling demands, adiabatic cooling systems can drastically reduce electricity usage, leading to substantial cost savings.
Environmental Sustainability
Adiabatic cooling aligns seamlessly with the drive towards environmental sustainability. By reducing energy consumption, adiabatic cooling lowers greenhouse gas emissions. Additionally, it can minimize the use of harmful refrigerants, often employed in conventional cooling systems. By embracing adiabatic cooling technologies, we can significantly mitigate our impact on the environment while achieving efficient cooling solutions.