Understanding The Additive Inverse Of Polynomials: Its Role In Mathematical Operations

The additive inverse of a polynomial is a polynomial that, when added to the original polynomial, results in the zero polynomial (0). It represents the opposite of the original polynomial in terms of addition. Every polynomial has a unique additive inverse. The zero polynomial, consisting of all zero coefficients, acts as the additive identity, with the additive inverse of any polynomial being its reflection across the x-axis. Each term in a polynomial has a coefficient, variable, and exponent, with the additive inverse simply changing the sign of all the coefficients. For instance, the additive inverse of the polynomial 2x³ – 5x² + 3 is -2x³ + 5x² – 3. Understanding the additive inverse is crucial for polynomial operations like subtraction and multiplication, where it is used to isolate terms and simplify expressions.

Unraveling the Secrets of Polynomial Additive Inverses: A Storytelling Guide

In the captivating world of mathematics, polynomials reign supreme, captivating us with their ability to represent real-life phenomena. Understanding their intricacies is crucial, and among them lies a fundamental concept that often goes unnoticed: the additive inverse.

Imagine two friends, Polynomial A and Polynomial B, who possess an intriguing relationship. While they may appear identical, they couldn’t be more different in essence. Polynomial A is a delightful creature, with each of its terms brimming with positivity. In stark contrast, Polynomial B exudes a somber mood, its terms adorned with negative signs.

Now, here’s the magical twist: when these two friends are combined, they cancel each other out, resulting in the birth of a new entity – the zero polynomial. It’s like a mathematical truce, where opposing forces neutralize each other. This special polynomial possesses remarkable properties and serves as the cornerstone of polynomial operations.

So, what exactly is the additive inverse of a polynomial? Simply put, it’s a polynomial that, when added to the original polynomial, yields the zero polynomial. It’s like a mirror image, reflecting the original polynomial but with all its signs reversed. Each positive term transforms into a negative one, and vice versa.

The significance of the additive inverse in polynomials cannot be overstated. It plays a pivotal role in operations such as subtraction and multiplication, allowing us to manipulate these mathematical expressions with ease. It’s like the secret ingredient that unlocks the full potential of polynomials, enabling us to delve deeper into their intriguing world.

Understanding the Zero Polynomial: The Additive Identity of Polynomials

Have you ever wondered about the special polynomial that acts as the starting point in the realm of polynomial operations? It’s called the zero polynomial, and it holds a unique and fundamental position in the mathematical world of polynomials.

The zero polynomial is a polynomial that has no terms. In other words, it’s like an empty slate, a polynomial with a total absence of variables and coefficients. This may seem like a trivial concept, but it plays a pivotal role in polynomial operations.

The zero polynomial is the additive identity in the world of polynomials. This means that when added to any polynomial, the result is always the original polynomial. Think of it as a magic wand that leaves everything unchanged when added. For instance, if you add the zero polynomial to 3x^2 + 2x – 1, you get the same polynomial back: 3x^2 + 2x – 1.

This additive identity property makes the zero polynomial the starting point for polynomial subtraction and multiplication. When subtracting one polynomial from another, the additive inverse of the second polynomial is added to the first polynomial. And when multiplying two polynomials, the zero polynomial acts as the neutral element, resulting in the zero polynomial.

The zero polynomial is like the zero in the world of numbers; it may seem insignificant, but it enables essential operations and plays a crucial role in the intricate realm of polynomial manipulations. It’s the foundation upon which the operations of polynomial addition, subtraction, and multiplication are built.

Understand the Building Blocks of Polynomials: Coefficients, Variables, and Terms

In the realm of mathematics, polynomials play a pivotal role in algebra, calculus, and beyond. To comprehend the intricate world of polynomials, it’s essential to delving into the foundational components that make them up: coefficients, variables, and terms.

A polynomial is a mathematical expression consisting of a sum of terms, where each term is a product of a numerical coefficient and a variable raised to a non-negative integer exponent.

• Coefficients: Each term in a polynomial has a numerical coefficient that multiplies the variable. Coefficients determine the magnitude and sign of the term.

• Variables: Variables are letters that represent unknown or changing values. They are the symbols that “vary” in a polynomial.

• Terms: Individual components of a polynomial are called terms. Each term is a product of a coefficient and a variable raised to a power.

For example, in the polynomial 2x³ – 5x² + 7, we have:

• Coefficient: 2
• Variable: x

• Exponent: 3

• Coefficient: -5

• Variable: x

• Exponent: 2

• Coefficient: 7

• Variable: No variable (known as a constant term)

By understanding these components, we can manipulate, simplify, and solve polynomial equations effectively. So, next time you encounter a polynomial, remember to break it down into its constituent parts for a deeper understanding of its behavior.

Finding the Additive Inverse: It’s All About a Sign Change

Imagine you’re walking down a path, and you encounter an obstacle that seems to be pulling you back. This obstacle represents the additive inverse of your progress. In the world of polynomials, finding the additive inverse is a crucial step in various operations.

To find the additive inverse of a polynomial, we need to understand that each term of the polynomial has a coefficient, a variable, and an exponent. The coefficient is a numeric value in front of the variable, while the variable is typically a letter like x or y. The exponent is the small number written at the top-right corner of the variable.

The additive inverse of a polynomial is a polynomial that, when added to the original polynomial, results in the special zero polynomial. The zero polynomial is like a neutral ground, where all the obstacles cancel each other out, leaving you with zero progress.

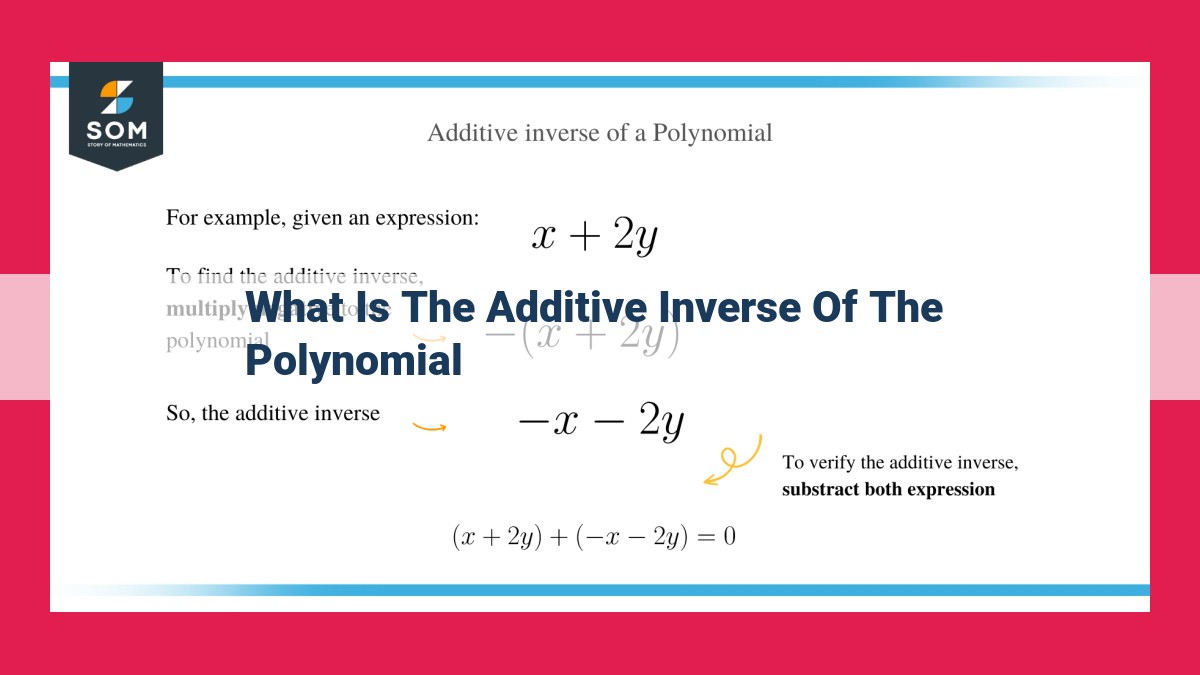
To find the additive inverse of a polynomial, we simply change the sign of each term. That means if a term is positive, we make it negative, and if it’s negative, we make it positive. This sign change reverses the direction of the polynomial, much like turning around on a path.

For example, let’s take the polynomial 2x² – 5x + 7. Its additive inverse would be -2x² + 5x – 7. Notice how the signs of all the terms have been reversed.

Adding the polynomial and its additive inverse gives us the zero polynomial, which is 0. This confirms the inverse relationship between the two polynomials.

Understanding the additive inverse is essential for various polynomial operations, including subtraction and multiplication. It’s like having a secret weapon in your algebraic toolbox, allowing you to conquer polynomial obstacles with ease.

Understanding the Additive Inverse of Polynomials: A Comprehensive Guide

In the realm of polynomials, the additive inverse plays a pivotal role, allowing us to manipulate and solve polynomial equations with greater ease. Let’s embark on a journey to unravel the secrets of the polynomial universe, starting with the fundamental concept of the additive inverse.

The Definition of the Additive Inverse

Every polynomial, no matter how complex, possesses an additive inverse, which is essentially its “mirror image” under the operation of addition. The additive inverse of a polynomial cancels out the original polynomial when added together, resulting in the zero polynomial.

The significance of the additive inverse lies in its ability to transform subtraction into addition. By subtracting a polynomial from itself, we are effectively adding its additive inverse, which simplifies the operation and avoids the hassle of negative coefficients.

Coefficients, Variables, and Terms

Before we delve deeper into the additive inverse, let’s briefly review the components of a polynomial. A polynomial consists of one or more terms, each of which comprises three key elements:

• Coefficient: A numerical factor that multiplies the variable.
• Variable: An algebraic symbol representing an unknown quantity.
• Exponent: The power to which the variable is raised.

The Additive Inverse as Sign Change

To find the additive inverse of a polynomial, simply change the sign of every term. For example:

• Additive inverse of 3x^2 – 2x + 1: -3x^2 + 2x – 1

An Example of Additive Inverse

Consider the polynomial: 2x^3 – 5x^2 + 7x – 1

Its additive inverse is: -2x^3 + 5x^2 – 7x + 1

If we add these two polynomials:

(2x^3 – 5x^2 + 7x – 1) + (-2x^3 + 5x^2 – 7x + 1) = 0

As expected, the result is the zero polynomial, confirming the additive inverse’s ability to cancel out the original polynomial.

Importance of the Additive Inverse

The additive inverse is an indispensable tool in polynomial operations. Here are some of its critical applications:

• Subtraction: Instead of subtracting one polynomial from another, we can add its additive inverse.
• Multiplication: When multiplying two polynomials with non-zero additive inverses, we can introduce the additive inverses to simplify the computation.

The additive inverse of a polynomial is a fundamental concept that empowers us to effortlessly manipulate and solve polynomial equations. By understanding this concept, we gain a deeper appreciation for the intricacies of polynomial operations and unlock a treasure trove of algebraic possibilities.

The Additive Inverse in Polynomial Operations

In the realm of polynomials, the additive inverse plays a crucial role in manipulating and solving algebraic equations. Understanding its significance is akin to unlocking a secret key that empowers us to perform mathematical operations with finesse.

When subtracting one polynomial from another, we essentially add its additive inverse. For instance, subtracting (2x^2 – 5x + 3) from (4x^2 + 3x – 2) is equivalent to adding its additive inverse ((-2x^2 + 5x – 3)). This inverse possesses the remarkable property of canceling out terms, leaving us with a simplified expression.

Multiplication also benefits from the additive inverse. If we multiply a polynomial by (-1), we effectively create its additive inverse. This concept is particularly useful when multiplying binomial polynomials. For example, multiplying (x – 2) by (-1) gives us (-x + 2), which allows us to multiply these binomials and obtain the difference of squares ((x – 2)(x + 2) = x^2 – 4).

Moreover, the additive inverse is indispensable for factoring polynomials. The difference of squares factorization, for instance, relies on subtracting the additive inverse of one term from the other term, revealing a factorable expression.

In essence, the additive inverse is a mathematical tool that empowers us to perform polynomial operations with ease and precision. By understanding its role in subtraction, multiplication, and factoring, we unlock the full potential of polynomial manipulations, enabling us to navigate algebraic equations with confidence and elegance.