Unlocking The Power Of The Addition Property Of Equality: Solving Equations And Beyond

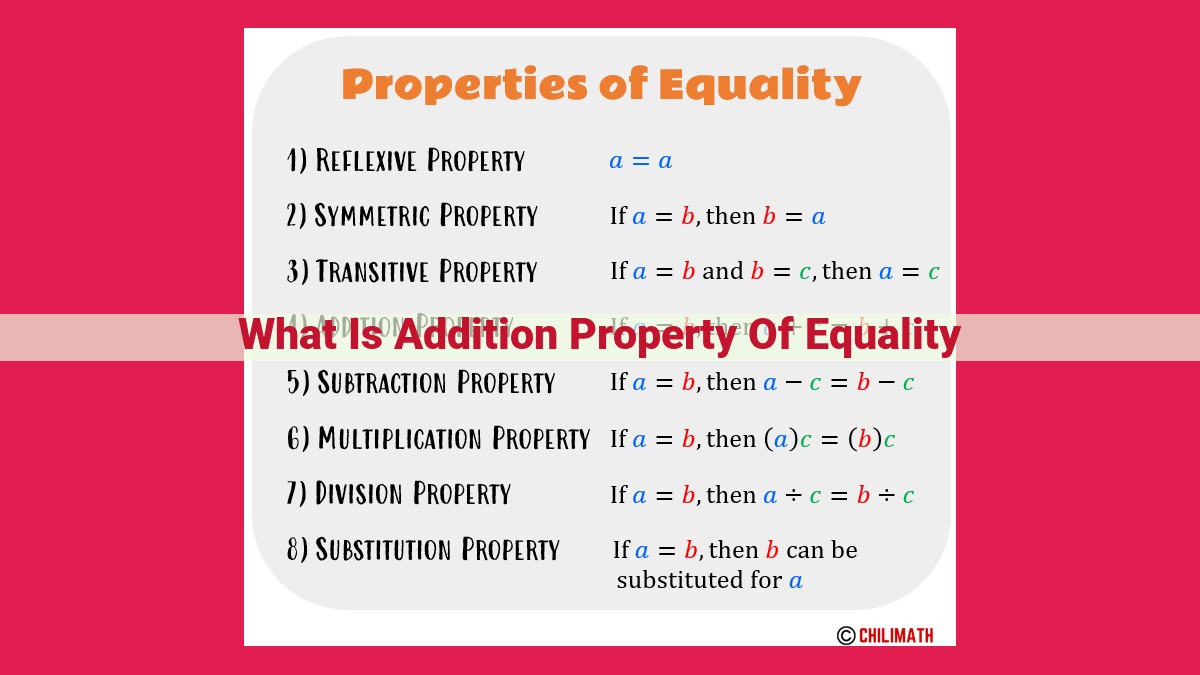
The Addition Property of Equality states that if you add the same number to both sides of an equation, the equation remains equal. In other words, if a = b, then a + c = b + c. This property is related to equations, addition, and inverse operations (subtraction). It is applied in various applications, such as solving equations, simplifying expressions, and proving mathematical theories. For instance, if you have the equation 2x = 6, you can add 4 to both sides to obtain 2x + 4 = 6 + 4, which simplifies to 2x + 4 = 10.

The Addition Property of Equality: Unraveling the Power of Equal Additions

In the realm of mathematics, we often encounter problems that require us to manipulate equations and expressions. One of the fundamental tools we use is the Addition Property of Equality, a pivotal concept that allows us to maintain the balance of equations while making them easier to solve.

Defining the Addition Property of Equality

The Addition Property of Equality simply states that if we add the same number to both sides of an equation, the equality remains unchanged. In other words, it allows us to add a number to both sides of an equation without affecting the truthfulness of the equation.

Understanding the Implications

This property is grounded in the idea that addition is an inverse operation of subtraction. When we subtract a number from both sides of an equation, we essentially add its opposite (negative) to both sides. Similarly, adding a number to both sides of an equation is equivalent to subtracting its opposite from both sides.

Applications in Practice

The Addition Property of Equality has wide-ranging applications in mathematics, including:

• Solving Equations: By adding the same number to both sides of an equation, we can isolate the variable and find its value.
• Simplifying Expressions: We can use the Addition Property to combine like terms and simplify algebraic expressions, making them easier to work with.
• Mathematical Proofs: The Addition Property is a fundamental building block for mathematical proofs, allowing us to manipulate equations logically and derive conclusions.

An Illustrative Example

Let’s consider an equation: 2x – 5 = 11

To solve for x, we can add 5 to both sides:

2x – 5 + 5 = 11 + 5

2x = 16

Dividing both sides by 2:

x = 8

This example shows how the Addition Property of Equality allows us to isolate the variable and find its value.

Embracing the Power of Equality

The Addition Property of Equality is a powerful tool that empowers us to manipulate equations and expressions with confidence. By understanding its implications and applications, we can unlock the secrets of mathematics and tackle even the most challenging problems with ease.

Related Concepts: Intertwining Equations, Addition, and Inverse Operations

Understanding the Addition Property of Equality requires a solid grasp of related concepts. Let’s unravel these key ingredients:

1 Equations: Balancing the Scales

An equation is a mathematical statement that asserts the equality of two expressions. It’s like a balancing scale, where the expressions on both sides represent equal weights. The Addition Property of Equality plays a crucial role in equations, allowing us to maintain this balance while performing operations.

2 Addition: The Building Blocks

Addition is a fundamental operation that combines two or more numbers to form a sum. In the Addition Property of Equality, addition is the key action that preserves the equivalence between expressions. By adding or subtracting the same quantity from both sides of an equation, we keep the scale in equilibrium.

3 Inverse Operation: Undoing Addition

An inverse operation is one that reverses the effect of another operation. In the case of addition, subtraction is its inverse operation. This means that adding and subtracting the same number from an expression cancel each other out, leaving the original value unchanged. This concept is central to the Addition Property of Equality, as it allows us to manipulate equations without altering their validity.

Applications of the Addition Property of Equality

Beyond its basic definition, the Addition Property of Equality finds widespread application in various mathematical contexts, empowering us to tackle complex problems with ease. Let’s explore its diverse uses:

Solving Equations

The Addition Property of Equality shines in the realm of equation-solving. By adding the same quantity to both sides of an equation, we can isolate the variable and find its value. This technique allows us to balance equations and determine unknown quantities, unlocking the secrets hidden within mathematical expressions.

Simplifying Algebraic Expressions

Algebraic expressions often present a tangled web of terms and variables. The Addition Property of Equality provides a guiding light through this complexity. By combining like terms on either side of an expression, we can simplify it, removing clutter and revealing its underlying structure. This streamlined form makes it easier to analyze and solve algebraic problems.

Proving Mathematical Theories

The Addition Property of Equality plays a pivotal role in the construction and validation of mathematical theories. In the intricate world of proofs, deductions, and theorems, this property forms the cornerstone of logical arguments. It allows mathematicians to manipulate equations, adding and subtracting quantities to demonstrate equalities and derive conclusions. Without this property, the edifice of mathematical knowledge would crumble, leaving us adrift in a sea of unproven claims.

The Addition Property of Equality: An Essential Tool for Problem Solving

Defining the Addition Property of Equality

In mathematics, the Addition Property of Equality states that if you add the same number to both sides of an equal equation, the two sides remain equal. In other words, for any numbers a, b, and c, if a = b, then a + c = b + c.

Understanding Related Concepts

Equations: An equation is a mathematical statement that two expressions are equal. The Addition Property of Equality is crucial for solving equations, as it allows us to isolate the variable we want to solve for.

Addition: Addition is the mathematical operation of combining two numbers to get a sum. In the Addition Property of Equality, we add the same number to both sides to maintain the equality.

Inverse Operation: Subtraction is the inverse operation of addition. If we add a number to both sides of an equation, the reverse operation, subtraction, is used to isolate the variable on one side.

Applications of the Addition Property of Equality

Solving Equations: The Addition Property of Equality is widely used in solving equations. By adding the same number to both sides, we can isolate the variable and determine its value.

Simplifying Algebraic Expressions: We can also use this property to simplify algebraic expressions. By adding or subtracting the same term from both sides, we can simplify complex expressions and make them easier to work with.

Proving Mathematical Theories: The Addition Property of Equality is fundamental in proving mathematical theorems. By applying this property, mathematicians can manipulate equations and expressions to demonstrate the validity of mathematical statements.

Example: Using the Addition Property of Equality to Solve an Equation

Let’s say we have the equation: x – 5 = 2

To solve for x, we need to isolate x on one side of the equation. We can do this by adding 5 to both sides:

x – 5 + 5 = 2 + 5

Simplifying the expressions:

x = 7

Therefore, the solution to the equation x – 5 = 2 is x = 7.