Accelerate Your Velocity To Speed: A Comprehensive Guide
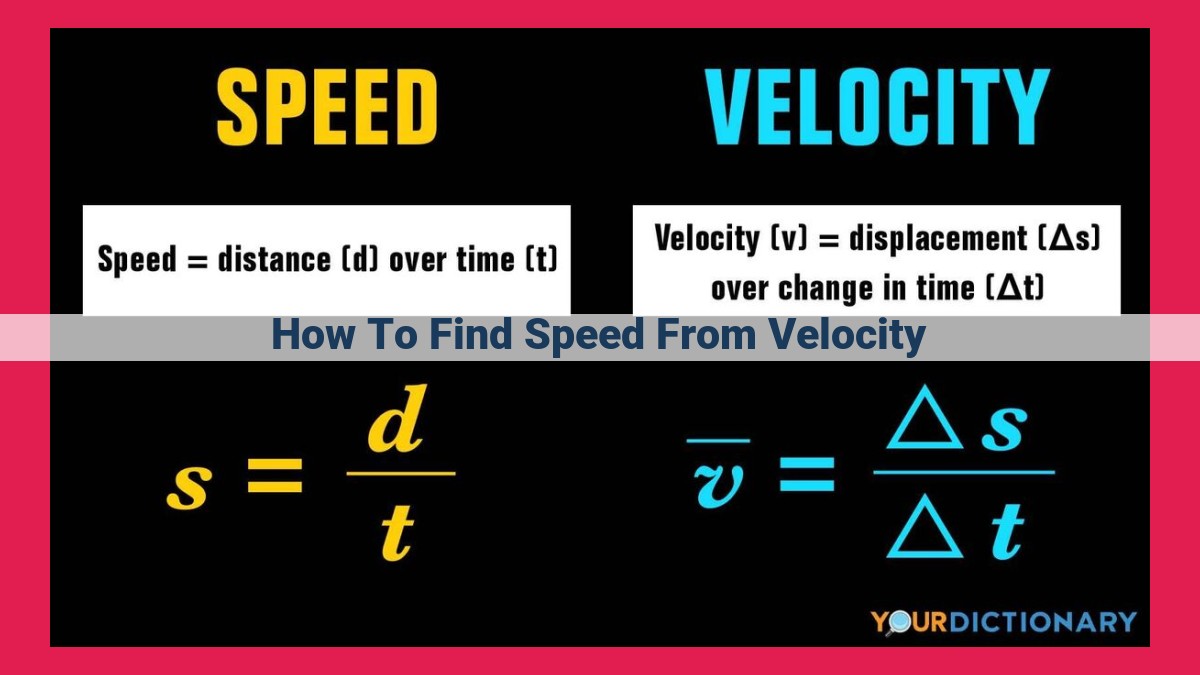
To find speed from velocity, first understand that velocity is a vector with both magnitude (speed) and direction. To calculate speed, use the formula: Speed = √(vx² + vy²), where vx and vy are velocity components. This yields a scalar value representing the object’s speed. Alternatively, speed can be calculated directly using the formula: Speed = Distance / Time, where distance is the displacement and time is the duration of movement. It’s crucial to distinguish between speed and velocity to ensure accurate calculations.
- Define velocity and speed and explain their differences.
- Emphasize the importance of understanding the distinction for accurate speed calculation.
Understanding Speed and Velocity: It’s Not Just About Fast or Slow
Welcome to the world of physics, where motion is a captivating dance, and understanding the difference between speed and velocity is like deciphering a secret code. Let’s embark on a storytelling journey to unravel this mystery.
Speed: The Lone Ranger of Motion
Speed, like a lone cowboy galloping across the vast prairie, is all about how fast an object is moving. It’s a scalar quantity, meaning it has only magnitude, like a speedometer that tells you how many miles per hour you’re traveling. It doesn’t care about which direction you’re heading, just how quickly you’re covering ground.
Velocity: The Guiding Star of Motion
Now, let’s introduce its cousin, velocity. Unlike speed, velocity is a vector quantity. This means it has both magnitude (speed) and direction. Imagine a compass, pointing to where an object is moving. Velocity is like the arrow on that compass, telling you both how fast and in which direction an object is traveling.
The Key to Accurate Speed Calculation
Understanding the distinction between speed and velocity is crucial for accurate speed calculation. Velocity provides the essential directional information that speed lacks. Just like a car can be moving at a constant speed but changing direction, speed alone cannot fully describe the motion.
Related Concepts: Distance and Time
As we delve deeper into the world of motion, we meet distance and time, two concepts that dance hand in hand with speed. Distance is the total length an object travels, while time is the duration it takes to cover that distance. Their relationship is like a recipe: speed equals distance divided by time.
Example: The Case of the Curious Car
Let’s say we have a car traveling at a speed of 60 miles per hour. So far, so good. But if we want to know more than just its speed, we need velocity. Let’s imagine the car is heading due north. Now we have all the information we need. The car’s velocity is 60 miles per hour due north.
In conclusion, understanding the difference between speed and velocity is like having a secret decoder ring for the language of motion. Speed is a measure of how fast something is moving, while velocity tells us both speed and direction. It’s all part of the fascinating world of physics, where motion becomes a story of speed, direction, and the dance of related concepts.
Understanding Velocity: Unraveling the Essence of Motion
What is Velocity?
Velocity, unlike its often confused counterpart, speed, is more than just the measure of how fast an object is moving. Velocity is a vector quantity, meaning it has both magnitude (speed) and direction. To truly understand an object’s motion, we need to know not only how fast it’s going but also which way it’s heading.
Breaking Down the Concept
Think of velocity as a compass needle. The length of the needle represents the object’s speed, while the direction it points indicates the object’s path. Velocity tells us the rate at which an object’s position changes over time, taking into account both distance and direction.
Related Concepts
Displacement is the vector that represents the change in an object’s position. Acceleration is the vector that describes how velocity changes over time. Jerk is the vector that measures how acceleration changes over time. Understanding these concepts helps us paint a complete picture of an object’s motion.
Calculating Speed from Velocity: A Guide to Understanding Scalar and Vector Quantities
In our exploration of motion, we often encounter the terms velocity and speed. While these concepts may seem interchangeable, there’s a fundamental difference between them that’s crucial for accurate speed calculation. Let’s delve into the distinction and discover how to calculate speed from velocity.
Velocity: A Vector Quantity
Velocity is a vector quantity, meaning it has both magnitude (speed) and direction. It describes the rate of change in an object’s position over time and is represented by an arrow that points in the direction of motion. Velocity encompasses related concepts such as displacement, acceleration, and jerk.
Calculating Speed from Velocity
To calculate speed from velocity, we need to extract the magnitude of the velocity vector, which represents the object’s speed. Speed is a scalar quantity, meaning it only has a numerical value without a specific direction. The formula for calculating speed from velocity is:
Speed = √(vx² + vy²)
where vx and vy are the components of the velocity vector in the x and y directions, respectively.
By applying this formula, we obtain a scalar value that represents the object’s speed, without any directional information.
Speed vs. Velocity: Unraveling the Difference and Calculating Speed
In the world of motion, velocity and speed are two concepts that often get tangled up, leaving us confused. But understanding the distinction between them is crucial for understanding motion accurately.
Understanding Velocity
Velocity is a vector quantity, meaning it has both magnitude (speed) and direction. Think of it as an arrow pointing from the starting point to the ending point of an object’s motion. Velocity describes the rate of change in displacement, the shortest distance between an object’s starting and ending points. It’s closely related to concepts like acceleration, jerk, and *time_.
Calculating Speed from Velocity
While velocity is a vector, speed is a scalar, meaning it’s just a magnitude or the rate of movement. To calculate speed from velocity, we use a simple formula:
Speed = √(vx² + vy²)
where vx and vy are the velocity components along the x and y axes, respectively. This formula essentially gives us the length of the velocity vector, representing the object’s overall rate of movement.
Related Concepts: Distance and Time
Two other key concepts related to speed are distance and time. Distance is the total length of the path an object travels, while time is the duration of its motion. The relationship between these three quantities is expressed in a fundamental formula:
Speed = Distance / Time
This formula allows us to calculate speed if we know the distance traveled and the time taken. Conversely, if we know the speed and one of the other quantities, we can find the missing value.
Velocity vs. Speed: Understanding the Distinction for Accurate Calculation
In the realm of physics, understanding the difference between velocity and speed is crucial for accurate motion analysis. While both terms describe the rate at which an object moves, they convey distinct information.
Defining Velocity and Speed
- Velocity is a vector quantity that not only indicates the magnitude (speed) but also the direction of an object’s motion.
- Speed, on the other hand, is a scalar quantity representing the magnitude of velocity, without specifying any direction.
Calculating Speed from Velocity
Since velocity is a vector, we can derive speed from it using the following formula:
Speed = √(vx² + vy²)
where:
- vx and vy represent the velocity components in the x and y directions, respectively.
Practical Application
Let’s illustrate this calculation through an example. Suppose an object moves with a velocity of 5 m/s north and 12 m/s east. To find its speed:
- Calculate the squared velocity components:
vx² = (5 m/s)² = 25 m²/s²
vy² = (12 m/s)² = 144 m²/s² - Plug these values into the formula:
Speed = √(25 m²/s² + 144 m²/s²) = √169 m²/s² = 13 m/s
Understanding the distinction between velocity and speed is essential for accurate calculations involving motion. Velocity provides a complete description of an object’s motion by encompassing both speed and direction, while speed solely represents the magnitude of the motion. By grasping this difference, individuals can confidently derive speed values from velocity data.